|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Sokolnikoff I.S. — Higher Mathematics for Engineers and Physicists |
|
|
 |
| Предметный указатель |
Line of equal potential 277
Line of flow 475
Line, contour 144
Line, coordinate 434
Line, direction cosines of 146 147 151
Line, normal 144 146—149
Line, stream 277 432 467
Line, tangent 143 147 151
Line, vector equation of 395
Linear dependence or independence 116 317
Linear differential equations 283—349 357
Linear differential equations with constant coefficients 287 357
Linear differential equations with variable coefficients 284 315—349
Linear differential operator 287—299
Log x 446
Logarithmic paper 526
M test 27
Maclaurin formula 36
Maclaurin's series 37 249
Magnitude of a vector 393
Map of a curve 466
Map, geographic 479
Mapping functions 467
Matrix 114—122
Matrix, augmented 118
Matrix, determinants of 115
Matrix, rank of 115
Maxima and minima for functions of one variable 158
Maxima and minima for functions of several variables 160
Maxima and minima, constrained 163
Mean error 516 522
Mean-value theorems 21
Measure numbers 397
Mechanical quadrature 554
Membrane, vibration of 377
Mercator's projection 479
Metric coefficients 437
Minima see “Maxima and minima”
minimax 162
Modulus of complex number 441 442
Modulus of elliptic function, k 51
Moment of inertia 177 180 182 183 187 190 191 196 241
Moment, bending 241
Moments, method of 544
Most probable value 505
Most probable value, approximation for probability of 511
Motion of a membrane 377
Motion under gravity 232
Motion, dead-beat 304
Motion, fluid 220
Motion, Laws of 231 234
Motion, oscillatory 304
Motion, pendulum 48 234
Motion, simple harmonic 233 301 314 380
Multiple integrals 172—196
Multiple integrals, definition and evaluation of 173 179
Multiple integrals, geometric interpretation of 177
Multiplication of complex numbers 442
Multiplication of determinants 110
Multiplication of series 21
Multiplication of vectors 399
Multiplicity of root 93 294
Multiplier, Lagrangian 165
Multiply connected region 205 212 455
Mutually exclusive events 497
Nabla, or del,  194 195 407 414 422 194 195 407 414 422
Newton's Law of attraction 218
Newton's law of cooling 254
Newton's law of dynamics, first law 231
Newton's law of gravitation 232
Newton's law, second law 231 272 363
Newton's law, third law 231 234
Newton's method of solution 97
Newtonian potential 196
Normal approximation 515
Normal derivative 144 146 152;
Normal distribution curve 516
Normal equations 537 540
Normal form 146
Normal law see “Gaussian law of error”
Normal line 144 146—149
Normal orthogonal functions 81
Normal to a curve 144
Normal to a plane 146 147
Normal to a surface 147 188 407
Numbers, complex 440
Numbers, measure 397
Numerical integration 554—560
Numerical solution of differential equations 346
Odd function 68
Operator 528
Operator, differential 287—299 357 406
Operator, vector see “Curl” “Divergence” “Gradient” “Nabla”
Order of differential equation 225
Ordinary differential equations 225—349; see also “Differential equations”
Ordinary discontinuity 64
Origin of a vector 393
Orthogonal curves 277 468
Orthogonal functions 81 339 345
Orthogonal systems 434
Orthogonal trajectories 277—279
Orthogonal vectors 398
Oscillation of a spring 299
Oscillatory motion 304
Overdamped 303
p series 10
parabola 244
Parabolic coordinates 439
Paraboloid, hyperbolic 162
Parachute 253 255
Parallelogram law of addition 394
Parameters 277 280
Parameters, integrals containing 167
Parameters, variation of 318
Parametric equations 143 149 150 199 215 247
Partial derivatives 125—143 153
Partial differential equation 350—391
Partial differential equation of elastic membrane 377
Partial differential equation of electric circuits 386
Partial differential equation of heat conduction 367 425
Partial differential equation of vibrating string 361
Partial differential equation, derivation of 351
Partial differential equation, Fourier 425
Partial differential equation, integration of 353
Partial differential equation, Laplace's 369 382 385 386 439
Partial differential equation, linear 357
Partial differentials 128—143
Partial differentiation 123—171
Partial fractions, method of 297
Partial sum 4
Particular integral 290 292 297 318 359
Particular solution 230
Path, integrals independent of 208 216 452 455
Pendulum, simple 44 234—238 306
Periodic function 64
Picard's method 347
Plane, equation of 147
Plane, inclined 280 282 306
Plane, normal form for 146
Plane, tangent 146—149
Point of inflection 159
Point, singular 451
Poisson formula 512
Polar coordinates 183 184 276 279 386 438
Polygon, rectilinear 478 485
Polynomials, Legendre 344 384
Porous solids, drying of 369
Potential function 219 411
Potential, electrostatic 487
Potential, gravitational 219 408
| Potential, lines of equal 277
Potential, Newtonian 196
Potential, velocity 221 222 277 430 432 453 467 480
Power series 30—62
Power series solutions of differential equations 325—346
Power series whose terms are infinite series 40
Power series, differentiation of 33 34
Power series, evaluation of integrals by 43—46
Power series, expansion in 35—46
Power series, functions defined by 33
Power series, integration of 33 34
Power series, interval of convergence of 31 33
Power series, operations on 33—35
Power series, theorems on 31—35
Power series, uniform convergence of 33
Power series, uniqueness of expansion in 38
Precision constant 520 521
Pressure on dam 484
primitive 458
Principal part of increment 128
probability 492—524
Probability curve 521
Probable error 521
Probable value, most 505
Probable value, probability of 511
Product of determinants 110
Product, scalar 399
Product, vector 400
Projection, Mercator's 479
Projection, stereographic 479
Pulley, slipping of belt on 239
pv diagram 223
Quadrature, mechanical 554
Quotient of complex numbers 444
Quotient of power series 40
Radius of convergence 31 33
Radius vector 195
Rank of matrix 115
Ratio Test 11 20 31
Reaction, chemical 258
Rearrangement of series 17
Rectilinear polygon 478 485
Recursion formula 273 328 331 334
Region of integration 173
Region, multiply connected 205 212 455
Region, simply connected 205
Regula Falsi 101
Regular functions 451
Remainder in Taylor's series 36—37
Remainder theorem 92
Repeated trials 501
Representation, applications of con-formal 479—491
Residuals 534 537
Resonance 310
Riemann surface 473
Right-handed system of axes 397
Rod, flow of heat in 373
Rod, vibrations of 366 367
Roots of equations 83—102
Roots of unity,  , , 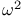 87 87
Roots, isolation of 92
Roots, theorems on 92—94
ROT see “Curl”
Rotational field 418
Rule, Cramer's 113
Rule, Simpson's 556
Rule, trapezoidal 556
Scalar field 406 408
Scalar point function 406 418
Scalar product 399
Scalar product, application of 404
Scalars 392
Schwartz transformation 478 485 491
Seepage flow 483
Separation of variables 257
Sequences 2
Sequences, fundamental principle of 6
Sequences, limit of 3
Series of constants 6—22
Series of functions 23—62
Series, asymptotic 524
Series, binomial 40
Series, evaluation of integrals by 43—46
Series, Fourier 63—82
Series, infinite 1—62
Series, integration and differentiation 29 33 34
Series, power 30—62
Series, solution of differential equations by 228 325—346
Series, Taylor's and Maclaurin's 37 155 228 249 464 539
Series, tests for convergence of 9 11 12 15 20 27 31 33
Series, theorems on 17 21 27 28 29 31 33 34 36 38
Series, uniform convergence of 23—30
Shearing stresses 485
Simple closed curve 200
simple harmonic motion 233 301 314 380
Simple harmonic motion, equation of 234
Simple harmonic motion, period of 234
simple pendulum 44 234—238 306
Simply connected region 205
Simpson's rule 556
Simultaneous differential equations 312—315
Simultaneous equations 102—122 139—141
Sin x 41 250
Sine series 73
Sine, hyperbolic 247
Sine, length of curve 55
Sine, power series for 40 41
Singular point 451
Singular solution 279
Singularities of function 222
sinh x 247
Sink see “Source and sink”
Six-ordinate scheme 548
Slipping of belt on pulley 239
Small numbers, law of 512
sn u 51
Solenoidal field 423
Solid angle 195
Solids, drying of porous 369
Solution of cubic 86—91
Solution of differential equations 226 228 325
Solution of differential equations, singular 279
Solution of equations 83—122
Solution of equations, algebraic 86 95
Solution of equations, graphical method of 83
Solution of equations, transcendental 85 97
Solution of systems of linear algebraic equations 102—122
Solution, genera 230 290 292 350 358
Solution, particular 230
Solution, steady-state 309 310
Source and sink 221 411 416 424 427 429
Space curves 149—152
Spherical coordinates 152 185 382 386 434 439
Spring 299 308 313
Spring, oscillation of 299
Standard deviation 523
Steady heat flow 256 368 369 427
Steady-state solution 309 310
Stereographic projection 479
Stirling's formula 508
Stokes's theorem 421
Stream function 221 432 453 481
Stream lines 277 432 467
Stresses, shearing 485
String, vibration of 361
Sum of a series 4
Sum of vectors 393
Superposition of effects 129 223
Surface integral 188—196 415 421
Surface, equation of 144
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте




 ,
,