|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Sokolnikoff I.S. — Higher Mathematics for Engineers and Physicists |
|
|
 |
| Предметный указатель |
Electrodynamics 422 42
Electron 315
Electrostatic field 475 477 479
Electrostatic force 487
Electrostatic potential 487
Electrostatics 486—491
Element of arc 467
Element of area 184 190 437
Element of distance 435
Element of volume 185 187 190 437
Elementary functions 315
Elementary functions, expansion of 35—46 65—82 465
Ellipse, area of 177 202
Ellipse, center of gravity of 177
Ellipse, length of arc of 47
Ellipsoidal coordinates 433
Elliptic functions 51
Elliptic integrals 47—55
Elliptic integrals, complete 48
Elliptic integrals, first kind,  48—55 238 48—55 238
Elliptic integrals, second kind, 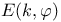 48—54 48—54
Elliptic integrals, third kind,  50 50
Empirical formulas 525—560
entropy 224
Envelope 279
Equation of continuity 221 429 481
Equation of plane 147
Equation, auxiliary 292
Equation, Bernoulli's 286
Equation, Bessel's 332 380
Equation, characteristic 292
Equation, cubic 86
Equation, Euler 322
Equation, Fourier 425
Equation, indicial 334
Equation, integral 347
Equation, Laplace's 195 369 382 385 386 439 451 470 481
Equation, Legendre's 342 384
Equation, wave 432
Equations, Cauchy — Riemann 221 450 455
Equations, consistent 117—122
Equations, dependent 105
Equations, differential 225—391
Equations, Euler's 430
Equations, inconsistent 105 117—122
Equations, normal 537 540
Equations, parametric 143 149 150 199 215
Equations, representing special types of data 528
Equations, simultaneous 102—122 139—141
Equations, solution of 83—122
Equations, systems of 102—122
Equations, systems of homogeneous linear 119—122
Equations, systems of non-homogeneous linear 113—119
Error function 516
Error of observation 516
Error, Gaussian law of 520 536
Error, mean 516
Error, mean absolute 522
Error, mean square 522
Error, probable 521
Error, small 56
Euler equation 322
Euler formulas 78 251
Euler's equations 430
Euler's theorem 136
Evaluation of integrals in series 43—46
Evaluation of integrals, by differentiation 169
Even function 68
Events, dependent 495
Events, independent 495
Events, mutually exclusive 497
Exact differential 211 212 216 222.224 262 411 418 420
Exact differential equation 262
Expansion in Bessel functions 339
Expansion in Fourier series 65—82
Expansion in Legendre polynomials 346
Expansion in Maclaurin's series 37
Expansion in power series 37—46
Expansion in Taylor's series 37
Expansion in trigonometric series 65
Expansion, uniqueness of 38
Expectation 500
Expected number of successes 508
Exponential form for trigonometric functions 78 251 446 447
Exponential function, expansion for 42 446
Extremal values 164
Extremum 164
Factor Theorem 92
Factor, integrating 265
Factorial, n!, approximation for 509; see also “Gamma functions”
Falling body 58 232
Field 406
Field, conservative 411
Field, electrostatic 475 477 479
Field, irrotational 418
Finite discontinuity 64
Fitting, curve 525—560
Flexure 298
Flow of a liquid 220 424 428 477 478 480—484
Flow of electricity in a cable 386
Flow of heat 256 368—377 423 425
Flow, seepage 483
Fluid Motion 220 424 428 475—478 480—484
Flux 416
Flux density, magnetic 52
force 217 239 392 406 409
Force function 411
Force, components of 217
Force, conservative field of 219
Force, electrostatic 487
Forced vibrations 308 310
Formula, asymptotic 509
Formula, Cauchy's integral 461
Formula, empirical 525—560
Formula, interpolation 550
Formula, Lagrange's 552
Formula, Poisson 512
Formula, Stirling's 508
Formula, Wallis's 45
Fourier coefficients 65
Fourier equation 425
Fourier series 63—82
Fourier series, complex form of 78
Fourier series, differentiation of 80
Fourier series, expansion in 65—82
Fourier series, integration of 80
Fourier series, solution of equations with 364
Functional dependence 2
Functional determinant 183
Functions 1
Functions of a complex variable 444—491
Functions of several variables 123 160
Functions, analytic 451
Functions, Bessel 336
Functions, beta 276
Functions, complementary 290
Functions, Conjugate 468 470
Functions, continuous 23 448
Functions, elementary 315
Functions, expansion of 35 65 155
Functions, gamma 272—277
Functions, holomorphic 451
Functions, homogeneous 136 259
Functions, hyperbolic 247—256
Functions, orthogonal 81 339 345
Functions, periodic 64
Functions, potential 219 411
Functions, power 30
Functions, real 2 123
Functions, regular 451
| Functions, scalar point 406
Functions, singularities of 222
Functions, stream 221 432 453 481
Functions, vector point 406
Fundamental principle of combinatory analysis 493
Fundamental principle of sequences 6
Fundamental theorem of algebra 92
Fundamental theorem of integral calculus 172 457
Gamma functions 272—277
Gauss — Argand diagram 440
Gauss's theorem 193
Gaussian law of error 520 536
General solution of differential equation 230 290 292 350 358
Geometric series 9
Gradient,  144 152 407 410 438 144 152 407 410 438
Graphical method of curve fitting 525
Graphical method of solution of equations 83
Gravitational constant 232
Gravitational law see “Attraction”
Gravitational potential 219 408
Gravity dam 483
Gravity, center of 177 182 183 187 190 191 196 522
Green's theorem for the plane 202
Green's theorem in space 191 418
Green's theorem, symmetric form of 194 418
Harmonic analysis 545
Harmonic series 8
Heat conduction 367
Heat flow 368—377
Heat flow, equation of 368 425
Heat flow, steady 256 368 427
Heat flow, variable 368 373 425
Helix 151 152
Holomorphic functions 451
Homogeneous equations, differential 259 261
Homogeneous equations, linear algebraic 119—122
Homogeneous equations, linear differential 290
Homogeneous function 136 259
Homogeneous function, definition of 136
Homogeneous function, Euler's theorem on 136
Hooke's law 241 299
Horner's method 95
Hydrodynamics 221 422 428—433 480—484
Hyperbola 247
Hyperbolic functions 247—256
Hyperbolic paraboloid 162
Imaginary roots 94
Implicit functions 132 137—142
Inclined plane 280 282 306
Incompressible fluid 424 430
Inconsistent equations 105 117—122
Independence of path 208 216 452 455
Independence, linear 116 317
Independent events 495
Independent trials 501
Indicial equation 334
Infinite series 1—62
Infinite series of constants 6—22
Infinite series of functions 23—62
Infinite series of power functions 30
Infinite series of trigonometric functions 63—82
Infinite series, absolute convergence of 16 17 20
Infinite series, conditional convergence of 16 17
Infinite series, definition of 4
Infinite series, expansion in 35—46 155—158
Infinite series, operations on 21 29 33—35
Infinite series, sum of 4
Infinite series, tests for convergence of 9 11 12 15 20 27 31 33
Infinite series, theorems on 17 21 27 28 29 31 33 34 36 38
Infinite series, uniform convergence of 23—30 33
Inflection, point of 159
Initial conditions 235 351
Integral calculus, fundamental theorem of 172 457
Integral curve 226 228 279
Integral equation 347
Integral formula, Cauchy's 461
Integral test for series 12
Integral theorem, Cauchy's 455
Integrals around closed curve 201 203 206 216 421
Integrals, change of variable in 183—188
Integrals, definite 172
Integrals, double 173 192 202 275
Integrals, elliptic 47—55 238
Integrals, evaluation of, by means of series 43—46
Integrals, iterated 175 180
Integrals, line 197—224 410 421 454 458
Integrals, mean-value theorem for 210
Integrals, multiple 172—196
Integrals, particular 290 292 297 318
Integrals, surface 188—196 415 421
Integrals, transformation of see “Green's theorem” “Stokes's
Integrals, triple 177 193
Integrals, volume 180 415
Integrals, with a parameter 47 167
Integrating factor 265
Integration by parts 276
Integration of complex functions 453
Integration of series 29 33 34 80
Integration, numerical 554—560
Integration, term by term 33 34 80
Interpolation formulas 550—554
Interpolation, method of 101
interval 4
Interval of convergence 31 33
Interval of expansion 38 76
Inverse hyperbolic functions 249 255
Inversions 106
Irrotational field 418 423 430
Isolation of roots 92
Isothermal process 224
Iterated integrals 175 180
Iteration, method of 297
Jacobian 141 183 190
Lagrange's interpolation formula 552
Lagrange's method of multipliers 163—167
Lamellar field 423
Laplace's approximation 515
Laplace's equation 195 369 382 385 386 439 451 470 481
Law of attraction 218
Law of conservation of matter 429
Law of cooling 254
Law of dynamics 231
Law of error 520 536
Law of gravitation 232
Law of small numbers 512
Law, Bernoulli — Euler 241
Law, binomial 502 512
Least squares, method of 536
Least squares, theory of 521
Legendre polynomials 344 384
Legendre polynomials, expansion in 346
Legendre's equation 342 384
Leibnitz's rule see “Differentiation under integral sign”
Leibnitz's test see “Test for alternating series”
Length of arc 143
Length of ellipse 47
Length of sine curve 55
Level surface 406
LIMIT 2 124 454
Line integrals 197—224 410 421 454
Line integrals around a closed curve 202 206 216 421
Line integrals for angle 195
Line integrals for area 201
Line integrals for work 217
Line integrals in space 215 410 421
Line integrals, applications of 217—224
Line integrals, definition of 197 454
Line integrals, evaluation of 202—206 458
Line integrals, properties of 206—217
Line integrals, transformation of 202 421
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте