Авторизация
Поиск по указателям
Shiryaev A.N. — Probability
Обсудите книгу на научном форуме Нашли опечатку?
Название: ProbabilityАвтор: Shiryaev A.N. Аннотация: This book contains a systematic treatment of probability from the ground up, starting with intuitive ideas and gradually developing more sophisticated subjects, such as random walks, martingales, Markov chains, ergodic theory, weak convergence of probability measures, stationary stochastic processes, and the Kalman-Bucy filter. Many examples are discussed in detail, and there are a large number of exercises. The book is accessible to advanced undergraduates and can be used as a text for self-study. This new edition contains substantial revisions and updated references. The reader will find a deeper study of topics such as the distance between probability measures, metrization of weak convergence, and contiguity of probability measures. Proofs for a number of some important results which were merely stated in the first edition have been added. The author included new material on the probability of large deviations, and on the central limit theorem for sums of dependent random variables.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Издание: Second EditionГод издания: 1995Количество страниц: 644Добавлена в каталог: 11.04.2008Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Mean value 37 Mean value vector 301 Mean-square 42 Mean-square, ergodic theorem 438 Measurable function 170 Measurable random variable 80 Measurable set 154 Measurable spaces 133 Measurable spaces 150 Measurable spaces 150 Measurable spaces 151 Measurable spaces 159 Measurable spaces 166 Measurable spaces 162 Measurable spaces 150 Measurable transformation 404 Measure 133 Measure counting 233 Measure discrete 155 Measure elementary 424 Measure, 133 Measure, 133 Measure, absolutely continuous 155 524 Measure, complete 154 Measure, consistent 567 Measure, countably additive 133 Measure, extending a 152 249 427 Measure, finite 132 Measure, finitely additive 132 Measure, Gaussian 268 Measure, Lebesgue 154 Measure, Lebesgue — Stieltjes 158 Measure, orthogonal 366 425 524 Measure, probability 134 Measure, restriction of 165 Measure, signed 196 Measure, singular 158 366 524 Measure, stochastic 423 Measure, Wiener 169 Measure-preserving transformations 404ff. Median 44 Method of characteristic functions 321ff. Method of moments 4 321 Metrically transitive 407 Minkowski inequality 194 Mises, R.von 4 Mixed model 421 Mixed moment 289 Mixing 409 Moivre see De Moivre moment 182 Moment and semi-invariant 290 Moment, absolute 182 Moment, method 4 Moment, mixed 289 Moment, problem 294ff. Monotone Convergence Theorem 186 Monotonic class 140 Monte Carlo method 225 394 moving averages 418 421 Multinomial distribution 20 21 Multiplication formula 26 Mutual variation 483 Needle (Buffon) 224 Negative binomial 155 Noise 418 435 Nonclassical hypotheses 328 337 nondeterministic 447 Nonlinear estimator 453 Nonnegative definite 235 Nonrecurrent state 574 Norm 260 Normal correlation 303 307 Normal density 66 161 Normal distribution function 62 66 156 161 Normal number 394 Normally distributed 299 Null state 574 Occurrence of event 136 Optimal estimator 71 237 303 454 461 463 469 Optional stopping 601 Ordered sample 6 Orthogonal decomposition 265 Orthogonal increments 428 Orthogonal matrix 235 265 Orthogonal random variables 263 Orthogonal stochastic measures 423 425 Orthogonal system 263 Orthogonalization 266 Orthonormal 263 267 271 Oscillations of submartingales 503 Oscillations of water level 420 Ottaviani’s inequality 507 Outcome 5 136 P-almost surely, almost everywhere 185 Pairwise independence 29 Parallelogram property 274 Parseval’s equation 268 Partially observed sequences 460ff. Parzen’s estimator 445 Pascal, B. 2 Path 48 85 95 Pauli exclusion principle 10 Period of Markov chain 571 Periodogram 443 Permutation 7 382 Perpendicular 265 Phase space 112 565 Physically realizable 434 451 Poincare recurrence principle 406 Poisson distribution 64 155 Poisson law of large numbers 599 Poisson limit theorem 64 327 Poisson — Charlier 269 Poisson — Charlier polynomials 269 Poisson, D. 3 Polya’s theorems, characteristic functions 287 Polya’s theorems, random walk 595 Polynomials, Bernstein 54 Polynomials, Hermite 268 Positive semi-definite 287 Positive state 574 Pratt’s lemma 211 599 Predictable quadratic variation characteristic 483 Predictable sequence 446 474 Preservation of martingale property 484 Principle of appropriate sets 141 Probabilistic model 5 14 131 Probabilistic model in the extended sense 133 probability 2 134 probability distribution 33 170 178 Probability distribution, discrete 155 Probability distribution, lognormal 240 Probability distribution, stationary 569 Probability distribution, table 155 156 Probability measure 134 154 524 Probability measure, absolutely continuous 524 Probability measure, complete 154 Probability of error 361 Probability of first arrival or return 574 Probability of mean duration 90 Probability of ruin 83 Probability of ruin in insurance 558 Probability of success 70 Probability space 14 138 Probability space, canonical 247 Probability space, complete 154 Probability space, universal 252 Probability transition 566 Probability, a posteriori, a priori 27 Probability, classical 15 Probability, conditional 23 76 214 Probability, finitely additive 132 Probability, measure 131 151 Probability, multiplication 26 Probability, total 25 77 79 Problems on arrangements 8 Problems on coincidence 15 Problems on ruin 88 Process with independent increments 306 Process, branching 115 Process, Brownian motion 306 Process, construction of 245ff. Process, Gauss — Markov 307 Process, Gaussian 306 Process, Markov 248 Process, stochastic 4 177 Process, Wiener 306 307 Prohorov, Yu.V. vii 64 318 Projection 265 273 Pseudoinverse 307 Pseudotransform 462 Purely nondeterministic 447 Pythagorean property 274 Quadratic characteristic 483 Quadratic covariation 483 Quadratic variation 483 Queueing theory 114 Rademacher system 271 Radon — Nikodym derivative 196 Radon — Nikodym theorem 196 599 Random elements 176ff. Random function 177 Random process 177 306 Random process with orthogonal increments 428 Random sequences 4 Chap.V 404 Random sequences, existence of 246 249 Random sequences, orthogonal 447 Random variables 32ff. 166 234ff. Random variables, absolutely continuous 171 Random variables, almost invariant 407 Random variables, complex 177 Random variables, continuous 171 Random variables, degenerate 298 Random variables, discrete 171 Random variables, E-valued 177 Random variables, exponential 156 244 245 Random variables, extended 173 Random variables, Gaussian 234 243 298 Random variables, invariant 407 Random variables, normally distributed 234 Random variables, simple 170 Random variables, uncorrelated 234 Random vectors 35 177 Random vectors, Gaussian 299 301 Random walk 18 83 381 Random walk in two and three dimensions 592 Random walk with curvilinear boundary 536 Random walk, simple 587 Random walk, symmetric 94 381 Rao — Cramer inequality 72 Rapidity of convergence 373 376 400 402 Realization of a process 178 Recurrent state 574 593 Reflecting barrier 592 Reflection principle 94 96 Regression 238 Regular conditional distribution 227 Regular conditional probability 226 Regular stationary sequence 447 Relatively compact 317 Reliability 74 Restriction of a measure 165 Reversed martingale 105 403 484 Reversed sequence 130 Riemann integral 204 Riemann — Stieltjes integral 204 Ruin 84 87 489 Sample points, space 5 Sampling with replacement 6 Sampling without replacement 7 21 23 Savage, L.J. 389 Scalar product 263 Schwarz inequality 38 see Cauchy Semi-definite 287 Semi-invariant 290 Semi-norm 260 Semicontinuous 313 Separable 267 Sequences almost periodic 417 Sequences of independent random variables 379 Sequences partially observed 460 Sequences, moving average 418 Sequences, predictable 446 474 Sequences, random 176 404 Sequences, regular 447 Sequences, singular 447 Sequences, stationary (strict sense) 404 Sequences, stationary (wide sense) 416 Sequences, stochastic 474 483 Sequential compactness 318 Series of random variables 384 Sets of convergence 515 Shifting operators 568 Sigma algebra 133 138 Sigma algebra generated by 174 Sigma algebra tail, terminal 380 Sigma algebra, asymptotic 380 Signal, detection of 462 Significance level 74 Simple moments 291 Simple random variable 32 Simple random walk 587 Simple semi-invariants 291 Singular measure 158 Singular sequence 447 Singularity of distributions 524 Skorohod, A.V. 150 Slowly varying 537 Spectral characteristic 434 Spectral density 418 Spectral density, rational 437 456 Spectral function 422 Spectral measure 422 Spectral representation of covariance function 415 Spectral representation of sequences 429 Spectral window 444 Spectrum 418 Square-integrable martingale 482 493 518 538 Stable 344 Standard deviation 41 234 State space 112 States, classification of 234 569 573 Stationary distribution 120 569 580 Stationary Markov chain 110 Stationary sequence Chap.V 404 Chap.VI 415 Statistical estimation, regularity 440 Statistically independent 28 Statistics 4 50 Stieltjes, T.J. 183 204 Stirling’s formula 20 22 Stochastic exponential 504 Stochastic integral 423 426 Stochastic matrix 113 587 Stochastic measure 403 424 Stochastic measure with orthogonal values 425 426 Stochastic measure, extension of 427 Stochastic measure, orthogonal 425 Stochastic process 4 177 Stochastic sequence 474 564
Реклама
 |
|
О проекте
|
|
О проекте



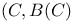





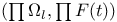
 -additive
-additive