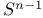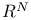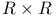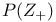|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Munkres J.R. — Topology: A First Course |
|
|
 |
| Предметный указатель |
Product space, local compactness 186
Product space, metrizability 131 132
Product space, normality 197 201 202 205
Product space, paracompactness 256 259
Product space, regularity 197
Product space, second-countability 191
Product space, subbasis 88 113
Products of continuous maps 112
Products of covering maps 336
Products of open maps 139
Products of quotient maps 138 141 187 289
Projection maps 88 113
Projective n-space 353
Projective plane 352 see
Proper map 285 315
Proper subset 4
Punctured euclidean space 155 see
Punctured plane 321 see
q 32
Quantifiers, logical 9
Quasicomponent 163
Quasicomponent vs. component 163 235
Quotient map 135
Quotient map, composites 138
Quotient map, products 138 141 187 289
Quotient operation in R 31
Quotient space 136
Quotient topology 136
Quotient topology vs. product topology 138 141 187
Quotient topology, Hausdorff condition 139 140 206
Quotient topology, normality 206
Quotient topology, subspace 138 '
R 30
R, compact sets in 174
R, connected sets in 154
R, metric for 118
R, paracompactness 255
R, standard topology 82
R, uncountability 176
Range of a function 16
Rational numbers 32
Ray in ordered set 86
Real numbers 30 see also “R”
Reciprocal 31
Recursion formula 48 55
Recursive definition 48
Recursive definition, principle 49 55
Recursive definition, transfinite 68
Refinement of a collection 225 251
Regular covering map 398
Regular Lindelof space, metrizability 220
Regular Lindelof space, normality 205
Regular Lindelof space, paracompactness 259
Regular space 195 see
Regularity 195
Regularity of G/H 145
Regularity of products 197
Regularity of subspaces 197
Regularity of topological groups 145
Regularity vs. complete regularity 238
Regularity vs. Hausdorff condition 195 200
Regularity vs. metrizability 217 249
Regularity vs. normality 195 200 201 205
Relation 21
Restriction of a function 16
Restriction of a relation 28
retract 216 345 see “Retraction”
Retraction 330
Retraction, 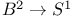 342 342
Retraction, 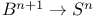 368 373 368 373
Retraction,  onto logarithmic spiral 221 onto logarithmic spiral 221
Retraction, 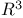 onto knotted x-axis 221 onto knotted x-axis 221
Retraction, deformation 373
Retraction, strong deformation 345
Reverse of a path 320
Right inverse 21
Rule of assignment 15
Russell’s paradox 62
Saturated set 135
Schoenflies theorem 385
Schroeder — Bernstein theorem 52
Second category 294
Second coordinate of ordered pair 13
Second-countability 190
Second-countability of  190 190
Second-countability of 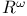 190 190
Second-countability of compact metric space 194
Second-countability of connected locally compact metric space 261
Second-countability of products 191
Second-countability of subspaces 191
Second-countability vs. countable dense subset 191 194
Second-countability vs. Lindeloef condition 191 194
Second-countable space 190 see also “Second-countability”
Section of 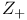 40 40
Section of ordered set 66
Semilocally simply connected 393
Separable 192 see
Separation by continuous functions of closed sets 211
Separation by continuous functions of points from closed sets 220
Separation of a space 147
Separation theorems,  by simple closed curve 377 386 by simple closed curve 377 386
Separation theorems, 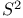 by by  377 386 377 386
Separation theorems, 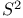 by simple closed curve 375 383 by simple closed curve 375 383
Separation theorems,  385 385
Sequence lemma 128
Sequences 37
Sequences in product spaces 116 281
Sequences vs. closure 128 190
Sequences vs. compactness 179 181
Sequences vs. continuity 128 190
Sequences, sums, differences, products and quotients 132
Sequential compactness 179
Sequential compactness vs. compactness 181 194
Set 3 4
Set, "Set of all sets" paradox 62
Set, rules for specifying 58
Shrinking lemma 224
Simple closed curves 375
Simple closed curves, generates 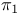 of of 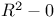 387 387
Simple closed curves, separates  377 385 377 385
Simple closed curves, separates 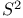 375 383 375 383
Simple order 24
Simplicial 2-complex 306 see
Simply connected space 328
Simply connected space, 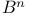 327 327
Simply connected space, 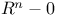 350 350
Simply connected space,  326 326
Simply connected space, 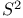 347 351 347 351
Simply connected space,  350 350
Simply connected space, contractible space 361
Slice in covering space 3 31
Slice in product space 168
Smaller topology 78
Smallest element of ordered set 27
Smirnov metrization theorem 260
Sorgenfrey plane 193 see
Space-filling curve 271
Special Van Kampen theorem 348
Sphere, unit 138 156 see “
Square metric 120 267 see
Square root of 2, irrationality 36
Square roots, existence 35
Star convex set 330
Stereographic projection 350 367
Stone — Cech compactification 241
Stone — Cech compactification of 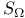 243 243
Stone — Cech compactification of 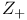 243 243
Stone — Cech compactification, connectedness 243
Stone — Cech compactification, metrizability 243
Stone — Cech compactification, uniqueness 242
| Stone’s theorem 256
Straight-line homotopy 321
Strict partial order 69
Strictly coarser topology 77
Strictly finer topology 77
Strong deformation retract 345
Strong deformation retract, fundamental group 345
Strong deformation retraction 345
Strong deformation retraction of 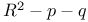 onto onto  and eight 345 346 and eight 345 346
Strong deformation retraction of 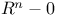 onto onto 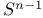 345 345
Strong deformation retraction vs. homotopy equivalence 372
Stronger topology 78
Subbasis for a topology 82
Subnet 188
Subsequence 179
Subset 4
subspace 89
Subspace of metric space 126
Subspace vs. box topology 114
Subspace vs. order topology 90
Subspace vs. product topology 90 114
Subspace vs. quotient topology 138
Subspace, basis for 89
Subspace, compactness 165
Subspace, complete regularity 236
Subspace, connectedness 147
Subspace, countable dense subset 193
Subspace, first-countability 191
Subspace, Hausdorff condition 99 197
Subspace, Lindeloef condition 194 220
Subspace, normality 197 201.205
Subspace, paracompactness 256
Subspace, regularity 197
Subspace, second-countability 191
Subtraction operation 31
Sup metric 267
Sup metric vs. uniform metric 267
Sup metric, completeness 267 268
Superset 232
Support 222
surface 223
Surjective function 19
Theta space 346 373
Theta space, separates 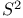 386 386
Tietze extension theorem 212
Topological dimension 302
Topological dimension in a metric space 304
Topological dimension of a 2-complex 306
Topological dimension of a 2-manifold 306
Topological dimension of a linear graph 305
Topological dimension of a manifold 314 315
Topological dimension of a set in  305 305
Topological dimension of a set in 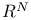 313 315 313 315
Topological dimension of a subspace 302
Topological dimension of a triangular region 306 359
Topological dimension of a union 304 315
Topological dimension of [a, b] 304
Topological group 144
Topological group, 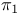 is abelian 331 is abelian 331
Topological group, closedness of  173 188 173 188
Topological group, complete regularity 237
Topological group, covering spaces of 342 398
Topological group, Hausdorff condition 145
Topological group, normality 207
Topological group, paracompactness 260
Topological group, regularity 145
Topological imbedding 105
Topological property 104
Topological space 76
Topologically complete space 270 see
Topologist’s sine curve 158
topology 76
Topology, generated by a basis 78 80
Topology, generated by a subbasis 82
Torus 134 333
Torus equals doughnut surface 334
Torus, covering by 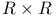 333 333
Torus, double 355
Torus, fundamental group 352
Torus, vector fields on 366
Totally bounded 275
Totally disconnected 152
Tower 74
Transcendental number 52
Transfinite induction 67
Transfinite recursive definition 68
Translation of 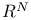 309 309
Triangle inequality 117
Trivial group 328
Trivial topology 77
Tube 168
Tube lemma 169
Tube lemma, generalized 172
Tychonoff theorem 232
Tychonoff theorem, countable 278
Tychonoff theorem, finite 167
Uncountability of 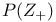 50 50
Uncountability of  50 50
Uncountability of R 176
Uncountability of transcendental numbers 52
Uncountable set 46
Uncountable well-ordered set 66 see
Uncountable well-ordered set, existence 73
Uniform boundedness principle 297
Uniform continuity theorem of calculus 146
Uniform continuity theorem, general 180
Uniform convergence 129
Uniform convergence on compact sets 282 see
Uniform convergence, Weierstrass M-test 134
Uniform limit theorem 130
Uniform limit theorem, converse fails 133
Uniform limit theorem, partial converse 172
Uniform metric 122 266 see
Uniform metric vs. sup metric 267
Uniform metric, completeness 266
Uniform structure 292
Uniform topology 122 266 see
Uniform topology vs. compact convergence topology 283
Uniform topology vs. pointwise convergence topology 283
Uniform topology vs. product topology 122
Uniform topology, compactness properties 181 277 279
Uniform topology, independence of metric 289
Uniformly bounded 278
union 5 12 38
Universal covering group 343
Universal covering space 341
Universal covering space, existence 397
Universal covering space, uniqueness 342 397
Universal extension property 216
Universal extension property vs. absolute retract 221
Universal neighborhood extension property 221
Upper bound 27
Urysohn lemma 207
Urysohn lemma for completely regular spaces 237
Urysohn lemma, strong form 215
Urysohn Metrization Theorem 217
Vacuously true 7
Value of a function 16
van Kampen theorem 349
Van Kampen theorem, special 348
Vanishing at infinity 279
Vector field 364
Vector field on  364 364
Vector field on 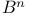 369 369
Vector field on 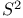 367 367
Vector field on  369 374 369 374
Vector field on Klein bottle 366
Vector field on torus 366
Vertices of a linear graph 304
Weaker topology 78
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



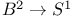
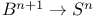
 onto logarithmic spiral
onto logarithmic spiral 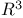 onto knotted x-axis
onto knotted x-axis 
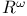
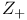
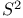 by
by 

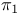 of
of 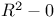
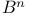
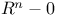
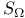
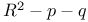 onto
onto  and eight
and eight