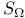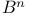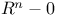|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Munkres J.R. — Topology: A First Course |
|
|
 |
| Предметный указатель |
Identity function 20
Image 16 17
Image set 15 16
Imbedding 105 see
Imbedding theorem for a 2-complex 310
Imbedding theorem for a compact manifold 223 314
Imbedding theorem for a completely regular space 237
Imbedding theorem for a linear graph 308
Imbedding theorem for a manifold 315
Imbedding theorem for an m-dimensional space 310 315
Imbedding theorem into 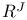 220 220
Immediate predecessor 25
Immediate successor 25
Index set 37
Indexed family of sets 37
Indexing function 37
Indiscrete topology 77
Induced homomorphism see “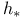 ” ”
Induction, Principle of 32
Induction, principle of, transfinite 67
Inductive definition see “Recursive definition”
Inductive set in a well-ordered set 67
Inductive set in R 32
Inessential map 357
Inessential map, induces zero homomorphism 358 371
Infinite broom 164
Infinite sequence 37
Infinite series 133
Infinite set 45 57
Initial point of a path 319
Injective function 19
Int A 95
Integers 32
Interior of a set 94
Intermediate value theorem of calculus 146
Intermediate value theorem, general 154
Intersection 6 12 38
Intersects 95
interval 25 84
Intervals in R, compactness 174
Intervals in R, connectedness 154
Intervals in R, topological dimension 304
Inverse function 19
Inverse image 17
Irrationality of  36 36
Irrationals, 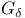 set 250 set 250
Irrationals, Baire 296
Irrationals, topologically complete 270
Isometric imbedding 132
Isometric imbedding into a complete space 268 271
Isometric imbedding surjectivity 182
Isometry 182
Isomorphism 105
J-tuple 38
Jordan curve theorem 383
Jordan separation theorem 375
K-fold covering 336
K-plane 309
Kuratowski 14-set problem 101
Larger topology 78
Largest element of an ordered set 27
Laws of algebra 33
Laws of exponents 35
Laws of inequalities 34
Least upper bound 27
Least upper bound property 27
Least upper bound property for R 31
Least upper bound property for well-ordered sets 67
Least upper bound property vs. greatest lower bound property 29
Lebesgue dimension 302
Lebesgue number 179
Lebesgue number lemma 179
Left inverse 21
Lens space 357
Lifting lemma for path homotopies 338
Lifting lemma for paths 337
Lifting lemma, general 342 390
Lifting of a map 336
Limit point 96
Limit point compact space 178
Limit point compactness of products 182
Limit point compactness vs. compactness 178 181 194
Limit point compactness vs. countable compactness 182
Lindelof condition 191 see
Lindelof condition for  192 192
Lindelof condition for 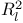 193 193
Lindelof condition for 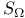 and and  194 194
Lindelof condition for products 193 195 235
Lindelof condition for subspaces 194 220
Lindelof condition vs. second-countability 191 194
Lindelof space 191
Linear continuum 31 152
Linear continuum, compact sets in 173
Linear continuum, connected sets in 152
Linear graph 304
Linear graph, imbedding in 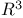 308 308
Linear graph, topological dimension 305
Linear order 24
lnvariance of domain 378 387
Local compactness 182 see
Local compactness of  and and  183 183
Local compactness of products 186
Local compactness, implies compactly generated 282
Local connectedness 161
Local connectedness of  and and  161 161
Local connectedness vs. connectedness in kleinen 163
Local connectedness vs. local path connectedness 162
Local homeomorphism 334
Local metrizability 220
Local metrizability vs. metrizability 220 260
Local path connectedness 161
Local simple connectedness 393 398
Locally compact Hausdorff space is Baire 296
Locally compact Hausdorff space, complete regularity 237
Locally compact Hausdorff space, imbedding in 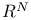 315 315
Locally compact Hausdorff space, one-point compactification 183
Locally compact Hausdorff space, regularity 205
Locally compact Hausdorff space, second-countability 261
Locally discrete collection 254
Locally finite collection 245
Locally finite indexed family 111 224
Locally finite indexed family vs. locally finite collection 246
Logical equivalence 8
Logical quantifiers 9
Long line 159
Long line, paracompactness 259
Loop 326
Lower bound 27
Lower limit topology 82 see
lub A 27
m-tuple 36
Manifold 223
Manifold, Hausdorff condition is needed 225
Manifold, imbeds in 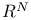 223 223
Manifold, imbeds in  314 315 314 315
Manifold, metrizability 224
Manifold, topological dimension 306 314 315
Mapping 16
Maximum principle 69
Maximum principle, applied 232 235
Maximum principle, intuitive proof 70
Maximum principle, proof 74
Maximum value theorem of calculus 146
Maximum value theorem, general 175
Mean convergence topology 284
Metric 117
Metric space 119
Metric space  121 121
Metric space  , uniform metric 266 , uniform metric 266
Metric space R 118
| Metric space, 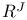 , uniform metric 122 , uniform metric 122
Metric space, 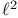 126 126
Metric space, Hausdorff condition 126
Metric space, normality 198
Metric space, paracompactness 256
Metric space, subspace 126
Metrizability of 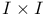 in dictionary order 195 in dictionary order 195
Metrizability of 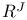 131 131
Metrizability of  121 121
Metrizability of  123 123
Metrizability of  in box topology 130 in box topology 130
Metrizability of $R_l} 195
Metrizability of 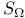 179 179
Metrizability of  131 131
Metrizability of manifolds 224
Metrizability of products 126 132
Metrizability of R 118
Metrizable space 119
Minimal uncountable well-ordered set 66 see
Multiplication operation 30
Nagata — Smirnov condition 245
Nagata — Smirnov metrization theorem, necessity 253
Nagata — Smirnov metrization theorem, sufficiency 249
Negation 9
Negative number 31
Neighborhood 96
Neighborhood retract 221
Nested sequence of sets 171
Net 187
Nonseparation theorems 378 382
Norm 120 ' '
Normal space 195 see
Normality 195
Normality of  202 202
Normality of adjunction space 221
Normality of closed subspace 205
Normality of coherent topology 216
Normality of compact Hausdorff space 198
Normality of metric space 198
Normality of paracompact space 255
Normality of product 197 201 202 205
Normality of regular Lindeloef space 205
Normality of subspace 197 201 205
Normality of well-ordered set 200
Normality vs. complete regularity 236
Normality vs. regularity 195 201 202 205
Nowhere-differentiable function 297
Number of elements in a set 40
Number of elements in a set, uniqueness 43
Odd integer 35
One 31
One-point compactification 183
One-to-one function 19
Open covering 164
Open interval 25 84
Open map 91 135
Open ray 86
Open refinement 251
Open set 76
Open set, relative to subspace 89
Operation, binary 30
Orbit space 357
Orbit space, fundamental group 357 see
Order of a collection 302
Order relation 24
Order topology 85 see
Order topology vs. subspace topology 90
Order topology, compact sets in 173 177
Order topology, Hausdorff condition 99
Order topology, normality 200
Order topology, regularity 205
Order topology, subbasis 91
Order type 25
Order-preserving function 25
Ordered field 31
Ordered pair 13
Paracompact space 225 255
Paracompact-manifold 261
Paracompactness 255
Paracompactness and partitions of unity 225
Paracompactness of  256 259 256 259
Paracompactness of 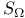 259 261 259 261
Paracompactness of compact Hausdorff space 255
Paracompactness of long line 259
Paracompactness of product 256 259
Paracompactness of R 255
Paracompactness of regular Lindeloef space 259
Paracompactness of subspace 256
Paracompactness vs. metrizability 256 260
Paracompactness vs. normality 255
Partial order 71
Partial order, axioms 72 187
Partial order, strict 69
Partition of a set 23
Partition of unity 222 225
Pasting lemma 108
Path 155
Path component 160
Path component vs. component 162
Path connectedness 155
Path connectedness of 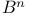 155 155
Path connectedness of 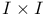 in dictionary order 156 in dictionary order 156
Path connectedness of 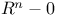 155 350 155 350
Path connectedness of  156 156
Path connectedness of deleted comb space 157
Path connectedness of long line 159
Path connectedness of topologist’s sine curve 158
Path connectedness vs. connectedness 156 158
Path homotopy 319
Path-homotopy class 320
Path-induced homomorphism 327
Peano curve 271
Peano space 274
Piecewise linear function 299
Plane in  309 309
Point of accumulation 96
Point-finite collection 247
Point-finite indexed family 224
Point-open topology 280
Point-open topology vs. compact convergence topology 283 290
Point-open topology vs. compact-open topology 286
Point-open topology, convergent sequences in 281
Pointwise bounded collection 278
Pointwise convergence topology 280 see
Positive integers 32
Positive number 31
Power set 11
Preimage 17
Principle of Induction 32
Principle of recursive definition 49 55
Principle of transfinite induction 67
Principle of transfinite recursive definition 68 '
Product space 86 113
Product space equals point-open topology 280
Product space vs. box topology 114
Product space vs. quotient topology 138 141 187 289
Product space vs. subspace topology 90 114
Product space vs. uniform topology, on 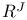 122 122
Product space, basis for 87 114
Product space, closures in 100 116
Product space, compactness 167 232 278
Product space, complete regularity 236
Product space, connectedness 150
Product space, convergent sequences in 116 281
Product space, countable dense subset 195
Product space, first-countability 191
Product space, fundamental group of 351
Product space, Hausdorff condition 99 115 197
Product space, limit point compactness 182
Product space, Lindeloef condition 193 195 235
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 ”
”
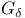 set
set 
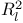
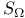 and
and 
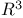
 and
and 
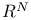

 , uniform metric
, uniform metric 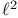
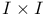 in dictionary order
in dictionary order