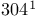Авторизация
Поиск по указателям
Reed M., Simon B. — Methods of Modern mathematical physics (vol. 4) Analysis of operators
Обсудите книгу на научном форуме Нашли опечатку?
Название: Methods of Modern mathematical physics (vol. 4) Analysis of operatorsАвторы: Reed M., Simon B. Аннотация: The book covers the theory about eigenvalues of Schrodinger operators. It is complete success in explaining clearly the basic concepts involved: perturbation theory (summability questions, fermi golden rule), min-max principle for discrete spectrum, Weyl theorem, HVZ theorem, the absence of singular continuous spectrum, ground state questions, periodic operators, semiclassic distribution of eigenvalues, compactness criteria.
Язык: Рубрика: Математика /Математическая Физика /Учебники /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1978Количество страниц: 396Добавлена в каталог: 24.04.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
32 Absolute value of an operator Absolutely continuous subspace Accretive operator Adjoint, Banach space Adjoint, Hilbert space Adjoint, unbounded operator Agmon potential 169 Agmon — Kato — Kuroda theorem 169 Algebraic multiplicity 9 Analytic family of type (b) 20 Analytic family, in the sense of Kato 14 Analytic family, type (A) 16 Analytic family, type (B) 20 Analytic Fredholm theorem Analytic function, vector-valued Analytic vector Anharmonic oscillator 20 32 41 64 84 Antilinear operator Approximate identity Approximate point spectrum 178 Aronszajn — Donoghue theory 140 Ascoli’s Theorem Associated string 129 Asymptotic series 26 Asymptotic series, order k strong 43 Asymptotic series, strong 40 Atomic model 304 Auger states 52 Autoionizing states 52 Axiomatic quantum field theory B.L.T. theorem Baire measure Balslev — Simon theorem 237 Banach space Barely connected 125 Bargmann’s bound 94 366 Basic period cell 303 Basis of a lattice 310 Bessel’s inequality Beurling — Deny criteria 209 Birman — Schwinger bound 98 Bochner integral Bochner — Schwartz theorem Borel set Borel summability method 44 Borel transform 44 Borel — Caratheodory theorem 328 Boson Fock space Bound state energies 79 Bound states 79 Bounded holomorphic semigroup Bounded operator Breit — Wigner resonance shape 53 Brillouin zone 311 Calogero’s bound 94 366 Canonical commutation relations Canonical form for compact operators Carleman’s theorem 39 Carlson’s theorem 236 Cauchy sequence Center of mass 197 Central potential 90 Characteristic equation 2 Characteristic function 221 Classical Weyl theorem 117 Closed graph theorem Closed operator Closed quadratic form Cluster decomposition 129 Commuting (unbounded) operators Compact operator “Relatively Compact operator, determinant 323 382 Compact operator, general theory 316—338 Compact operator, Hilbert — Schmidt Compact operator, ideal theory Compact operator, resolvent 244—260 Compact operator, trace class Compact sets 244 Compact support, distributions Compact support, functions Complex scaling see “Dilation analytic potentials” Complex thresholds 191 Conditionally negative definite 215 Conditionally positive definite 214 Contented set 271 Continuous functional calculus Contraction semigroup Convex function 104 Convex set Convolution, distributions Convolution, functions Core Coupling constant 11 Critical value 346 Cwikel — Lieb — Rosenbljum bound 101 D-thresholds 189 Darwin correction 81 Decomposable operator 281 Deficiency indices Degenerate eigenvalue 2 Density of states 313 Density of states measure 312 Determinant 323 382 Diagram, barely connected 125 Diagram, disconnected 125 Diagram, k-connected 129 Dilation analytic potentials 184 Dilation operators 56 183 Dirac operator Direct integral 280 358 Direct sum, of Banach spaces Direct sum, of Hilbert spaces Dirichlet Laplacian 263 Dirichlet problem Dirichlet — Neumann bracketing 262 Disconnected part 125 Disconnected string 129 Discrete spectrum 13 Discriminant 296 Distribution of eigenvalues, Dirichlet Laplacian 260 Distribution of eigenvalues, Schrodinger operators 86 Distributions, compact support Distributions, tempered Domain, analytic function Domain, form Domain, function Domain, unbounded operator Dominated Convergence Theorem Double well potential 34 Dual basis 305 Dual space Dunford functional calculus Dyson expansion Eigenvalue Eigenvalue, degenerate 2 Eigenvalue, discrete 13 Eigenvalue, multiplicity 9 316 Eigenvalue, nondegenerate 9 13 Eigenvalue, pseudo 48 Eigenvalue, simple 9 Eigenvalue, stable 29 Eigenvector Eigenvector, generalized 316 Eigenvector, pseudo- 48 Elliptic operator 346 Elliptic regularity energy levels 11 79 Energy operator see “Hamiltonian” Equicontinuous function Ergodic operator 202 Essentially self-adjoint Exceptional set § XI.6 Vol. Extension of an operator Fan’s inequality 383 Fermi energy 314 Fermi gas picture 356 Fermi Golden Rule 52 59 Fermi statistics Fermi surface 314 Fermion Fock space Feynman — Kac formula Fibers 280—281 Finite partition 208 First resolvent formula 1911 Fock space 53! FORM see also “Quadratic forms” Form, core Form, domain Form, domain, of operator Formal series 73 Fourier inversion theorem Fourier transform Friedrichs’ extension Functional calculus 245 Functions of rapid decrease Generalized eigenspace 9 Generalized eigenvector 316 Generalized function Generator group 268l Generator semigroup Geometric eigenspace 9 Geometric multiplicity 9 GGMT bound 94 Ghirardi — Rimini bound 367 Golden — Thompson inequality Graph 831 250 Graph limits 293 Green’s functions 592 263 Ground state 201 Ground state energy 201 Gyration, radius of 197 H-smooth on 163 H-smooth operator 142 Hadamard’s three line theorem Hahn — Banach theorem Hamiltonian Hamiltonian, free Hamiltonian, time dependent Hausdorff — Young inequality Helium Hamiltonian 80 Hermite functions Hermite functions, completeness Hermitian operator see “Symmetric operator” Hilbert space Hilbert — Schmidt operators Hilbert — Schmidt theorem Holder continuous 191 Holder’s Inequality Holder’s inequality, matrices 385 Holder’s inequality, operators Holomorphic semigroup Holomorphic semigroup, bounded Hughes-Eckart term 80 Hunziker’s theorem see “HVZ theorem” HVZ theorem 121 Hypercontractive semigroup Hyperfine structure in hydrogen 19 Ichinose’s lemma 183 Index of a string 129 Infinitely divisible 221 Infinitesimal generator, group Infinitesimal generator, semigroup Infinitesimally small form Infinitesimally small operator Interpolation theorems Inverse Fourier transform Iorio — O’Carroll theorem 154 Irreducible Jensen’s inequality (17) 104 Jordan content 271 Jordan normal form 10 Jost solution §XI.8 Vol. k-connected diagram 129 Kato — Agmon — Simon theorem 226 Kato — Rellich theorems 15 Kato — Simon theorem 193 Kato’s inequality 351 Kato’s projection theorem 22 Kato’s smoothness theorem 152 Kato’s theorem KLMN theorem Kohn’s theorem 301 Korteweg-de Vries equation 300 Kronig — Penny model 381 Lamb shift 81 86 Laplacian, Dirichlet 263 Laplacian, Neumann 263 Laplacian, on Lattice, ordered sense Lattice, subgroup sense 310 Lavine’s theorem 159 Levy — Khintchine formula 215 Levy — Khintchine Theorem 221 Lidskii’s theorem 328 Lieb — Thirring bound 368 Lipschitz 154 Local smoothness (operators) 163 Local Sobolev spaces 253 m-accretive Mathieu equation 298 Measurable functions, sets Measurable unbounded operator-valued function 283 Measurable, weakly and strongly 64 Measure Meromorphic Fredholm theorem 107 Min-max principle 76 Monotone Convergence Theorem Monotone convergence theorem, for nets Multiplicity of an eigenvalue 9 Multiplicity theorem Neumann Laplacian 263 Norm Norm resolvent sense, convergence in Norm, operator Normal coordinates 197 Normal operator One-electron model of solids 312 One-parameter unitary group Operator, adjoint, Banach space Operator, adjoint, Hilbert space Operator, adjoint, unbounded Operator, bounded Operator, compact Operator, compact resolvent 244 Operator, contraction Operator, decomposable 281 Operator, dilation 183 Operator, elliptic 346 Operator, energy (Hamiltonian) Operator, general theory Operator, Hamiltonian Operator, Hermetian see “Operator symmetric” Operator, Hilbert — Schmidt Operator, ideals Operator, infinitesimally form small Operator, infinitesimally small Operator, locally smooth 163 Operator, momentum
Реклама
-vector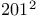 
inequalities 
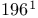
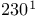
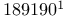
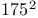
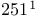
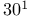
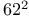
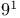
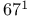
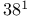
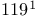
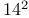
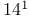
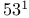
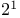
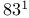
l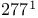
—
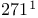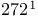
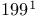(see also “Relatively compact”
—
—
—
—
—
—
—
—
|
|
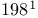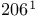
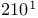
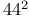
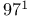
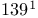
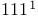
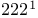
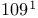
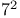
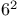
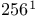
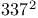
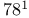
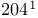
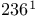
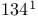
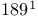
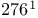
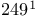
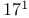
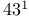
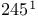
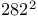
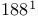
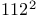
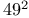
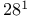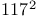
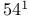
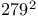
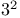
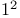
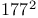
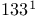
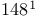
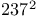
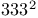
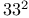
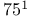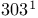
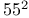
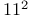
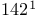
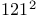
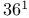
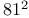
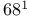
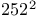
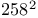
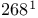
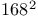
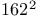
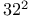
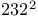
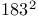
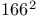
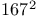
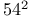
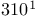
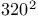
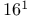
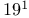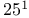
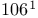
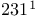
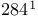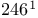
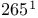
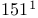
О проекте