Авторизация
Поиск по указателям
Arnold V.I., Khesin B.A. — Topological methods in hydrodynamics
Обсудите книгу на научном форуме Нашли опечатку?
Название: Topological methods in hydrodynamicsАвторы: Arnold V.I., Khesin B.A. Аннотация: Topological hydrodynamics is a young branch of mathematics studying topological features of flows with complicated trajectories, as well as their applications to fluid motions. It is situated at the crossroad of hyrdodynamical stability theory, Riemannian and symplectic geometry, magnetohydrodynamics, theory of Lie algebras and Lie groups, knot theory, and dynamical systems. Applications of this approach include topological classification of steady fluid flows, descriptions of the Korteweg-de Vries equation as a geodesic flow, and results on Riemannian geometry of diffeomorphism groups, explaining, in particular, why longterm dynamical weather forecasts are not reliable. Topological Methods in Hydrodynamics is the first monograph to treat topological, group-theoretic, and geometric problems of ideal hydrodynamics and magnetohydrodynamics for a unified point of view. The necessary preliminary notions both in hydrodynamics and pure mathematics are described with plenty of examples and figures. The book is accessible to graduate students as well as to both pure and applied mathematicians working in the fields of hydrodynamics, Lie groups, dynamical systems and differential geometry.
Язык: Рубрика: Математика /Математическая Физика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1998Количество страниц: 391Добавлена в каталог: 23.04.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
323 ABC flows 72—73 286—287 Action along a path 231 Action along a path, least action principle 1 16 Action-angle variables 100 308 Adjoint operator 4 Adjoint operator, orbit 8 Adjoint operator, representation 4 Aharonov — Bohm effect 199 Almost complex structure 330 Angular momentum, relative to the body 16 Angular momentum, relative to the space (or spatial) 16 Angular velocity 4 15 Angular velocity, relative to the body 15 Angular velocity, spatial 15 Anosov map 265 Antidynamo theorems 273—277 Antidynamo theorems, discrete 284—285 Antidynamo theorems, proofs of 281—284 Approximation, of generalized flows 238 Approximation, of vortex equation 56—59 Asymptotic, crossing number 152—155 Asymptotic, linking number 140—144 Attractor 64 66 Attractor, dimension of attractor 66 Attractor, general 279 Barotropic fluid 318 Beltrami fields 72 Beltrami fields, generalized 110 Bernoulli function 70 110 Bernoulli function, sequence 268 Bernoulli function, surface 111 Berry phase 58 199 Betti number 276 Bi-Hamiltonian system 309 Bi-invariant (pseudo-)metrics 252 Biot — Savart integral 146 Borromean rings 132(fig) 174(fig) 175 Brownian motion 134 Burgers equation 306 Calabi invariant 246—249 Calabi invariant, form 249 Calabi invariant, integral 249 Calugareanu formula 178 Cascade of solitori 168(fig) Casimir functions 47 48 312 Cat map 104(fig) 265(fig) 266 288 Characteristic path length 202 218 Chern — Simons functional 189 Circulations of vortices 57 Clebsch variables 324 Coadjoint representation 10—11 Coadjoint representation, invariants of 47—48 Coadjoint representation, orbit 11 Coboundary 304 316 Cocycle 304 316 Cohomology complex 316 Cohomology complex, group 316 Commutant subalgebra 35 257 Commutant subalgebra, subgroup 35 247 Commutator in Lie algebra 7 Commutator in Lie algebra, of vector fields 7 13 Compatible Poisson structures 309 Completely integrable system 308 Complex structure 330 Configuration space of fluid 32 Confocal quadrics 340 342 Conformal modulus 159 Conjugate point 224 Conjugate point, first 224 224(fig) 241 Conservation laws 43—44 55 62 Coriolis-type term 323 Coset of 1-forms Cosymmetry 114 Covariant derivative 119 202 Cowling theorem 274 Cross-helicity 55 Crossing number 152 Crossing number, asymptotic 152 153 155 Crossing number, average 153 Crossing number, of a knot 155 Crossing number, topological 154 Curvature tensor 200 Curvature, constant negative 197 293 Curvature, Ricci 200 Curvature, scalar 201 Curvature, sectional 200 Cut point 240—241 De Rham current 59 186 328 de Rham current, linking form 147—151 Degree, of a function 157 Degree, of a map 144 Degree, of a satellite link 155 Density form 320 Derivative, covariant 199 202 Derivative, exterior 32 Derivative, inner 32 Derivative, Lie 5—6 32—33 Derivative, Schwarzian 317 Derivative, variational 291 313 Diameter problem 226 Diffeomorphism, attainable 227—228 Diffeomorphism, Hamiltonian 242 Diffeomorphism, structurally stable 338 Diffeomorphism, symplectic 242 Diffeomorphism, volume-preserving 2 Diffeomorphism, volume-preserving, exact 14 35 Dirichlet integral 78 Dirichlet integral, problem 336 Dirichlet integral, variational problem 78—80 Discrete flow 234—235 Displacement energy 253 Divergence 125 Dual space of a Lie algebra 10 33—34 Dynamo equation 259 Dynamo equation, kinematic 259 Dynamo equation, self-consistent 262 Dynamo, discrete 261 Dynamo, dissipative 261 Dynamo, fast 260—261 Dynamo, in 264 Dynamo, kinematic 260 Dynamo, mean 270 Dynamo, nondissipative 261 Dynamo, rope 286 Dynamo, self-consistent 262 Dynamo, slow 260 Elliptic coordinates 340 342 Energy 2 Energy, 153 Energy, Hamiltonian function 37 Energy, minimization 75 Energy, of a curve 160 Energy, of a vector field 19 75 119 Energy, of MHD system 50 Energy, relaxation 129 Energy, second differential of 86 Energy, spectrum of a knot 162 Energy-Casimir method 95 Energy-momentum method 95—96 Enstrophy invariants 43 Entrophy 299—300 Equation in variations 88 Euler equation, of ideal hydrodynamics 20 Euler equation, of ideal hydrodynamics, aproximation of 56—59 Euler equation, of ideal hydrodynamics, generalized 37 Euler equation, of ideal hydrodynamics, on Riemannian manifolds 31 Euler equation, of ideal hydrodynamics, stationary 69—70 Euler equation, of rigid body 16—17 Euler theorem, first 16 Euler theorem, second 16 Euler — Helmholtz equation 20 56 Exact diffeomorphism 14 35 Existence and uniqueness theorems in hydrodynamics 24—25 Exponential instability 100 102 216 Extension of a Lie algebra 304 Exterior derivative 32 Extra symmetries 113 114 Extremal fields 76 80 120—121 Fast dynamo 260—261 Filament equation 332—333 First cut point 240—241 First cut point, local 241 First integral 43—44 308 Fluid, barotropic (or isentropic) 318 Fluid, compressible 322—323 Fluid, incompressible 2 Fluid, perfectly conducting 323 Fluid, viscous 63—67 Fluid, with a free boundary 323 Flux 130 159 Focal quadrics 343 Fokker — Planck equation 277—281 Force-free field 71 Form-potential 125 Form-residue 180 Frozen-in (or transported) field 22—23 49 Galerkin approximation 63 66 Gas dynamics 318 333 Gauss linking number 130 Gauss linking number, theorem (or formula) 143 Gauss — Bonnet theorem 296 Gauss — Codazzi formula 221 Gauss — de Rham linking form 147—151 Gaussian integral 186 Gelfand — Fuchs cocycle 304 317—318 Generalized flow (GF) 236—238 Genus of a knot 156 Geodesic 198 Geodesic, flow 293 Geodesic, submanifold 210 Geodesic, variation 201 Gibbs solution 278 Godbillon — Vey class 191—192 Group 1 Group, Lie group 1 Group, of diffeomorphisms 2 Group, of symplectomorphisms 242 Group, unimodular 97 206 Hamiltonian function 25 Hamiltonian function, field 25 27 Hamiltonian function, KdV structure 313—314 Hamiltonian vortex approximations 56—59 Hasimoto transformation 332 Heisenberg magnetic chain 333 Helicity 43 121 125 Helicity, invariance theorem 122 (see also “Hopf invariant”) Helicity, relative 167 Helicity, theorem 141 Helmholtz (or vortex) equation 20 38 47 56 99 Hermitian inner product 108 Hessian matrix 114 223 Hofer's metric 252 Holonomy, asymptotic 187 Holonomy, average 287 Holonomy, functional 190 Homeoidal density 341 Homoclinic point 267 268(fig) Homothetic quadrics 340 Homotopy formula 33 39 275 Hopf invariant of a field (or helicity) 43 121 125 Hopf invariant of a map 127 128(fig) 174 Hopf vector field 123 141 Horocycle 294 Horocyclic flows 294 Horseshoe 267 268(fig) Immersed knot 330—331 331 Incompressible fluid 2 Inertia operator 14 36—37 Infinite conductivity equation 324—325 Inner automorphism 3 Inner product, of a vector field with a form (or inner derivative) 12 32 Inner product, on vector fields 36 Inscribed angle theorem 338 Invariant of coadjoint action 47—48 Isentropic fluid see “Barotropic fluid” Isovorticed fields 80 Ivory theorem 341 Jacobi equation 201 Jacobi equation, identity 7 26 Jones — Witten functional complex 189 Jones — Witten functional complex, for a link 185 Jones — Witten functional complex, for a vector field 188 Kadomtsev — Petviashvili equation 323 Kahler geometry 326 KAM theory 101 133 KdV equation see “Korteweg — de Vries equation” KdV Hamiltonian structure, first 314 KdV Hamiltonian structure, second 313 Kinematic dynamo 260 kinetic energy 2 16 29 Kirchhoff equations 50 Kirillov — Kostant structure 25 329 Knot invariants 183 326 Knot invariants, Jones — Witten 185 Knot invariants, Vassiliev 183 Kolmogorov's conjectures 64 Korteweg — de Vries equation 305 Kronecker symbol 43 46 Lagrangian coordinates 49 Lagrangian coordinates, instability 216 Lagrangian coordinates, submanifold 253 Landau — Lifschitz equation 333—334 Laplace — Beltrami operator 123 275 Lefschetz formula 273 Lefschetz formula, number 273 Lefschetz formula, number, asymptotic 273 Left-invariant metric 14 19 196 Leibniz identity 26 40 Lenard scheme 310 310(fig) 314 Leray residue 180 Lichnerowicz cocycle 326 Lie algebra 4 7 32 Lie algebra, abstract Lie algebra 7 Lie algebra, dual space of 10 Lie algebra, of vector fields 32 Lie algebra, vector space of 4 Lie bracket of vector fields 32 Lie derivative 6 32 39 Lie group 1 7 32 Lie — Poisson structure (or bracket) 27 310 Lifting indices 37 322 Linear Poisson bracket see “Lie — Poisson bracket” Linearized vortex equation 99 Linearized vortex equation, Navier — Stokes equation 296 Link invariant, complex 184 Link invariant, Jones — Witten 185 Link invariant, Vassiliev 183 Link, essential 132 Link, satellite 155 Link, trivial 132 Linking form 147—151 Linking form, complex 184 Linking number 130 Linking number, asymptotic 140(fig) 141 144 171 Linking number, average 141 170—171 Linking number, for cascades 167 Linking number, holomorphic 181 Linking number, mutual (or multilinking number) 171—172 Linking number, of third order 175
Реклама
 |
|
О проекте
|
|
О проекте



 -plane equation
-plane equation 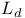 -norm
-norm  -energy
-energy