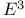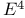|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| O'Neill B. Ч Elementary differential geometry |
|
|
 |
| ѕредметный указатель |
 , m, n 211Ч213 , m, n 211Ч213
1-segment 167
2-segment 169
Acceleration 54 68
Acceleration of a curve in a surface 196 324(Ex.
Acceleration, intrinsic 320
Adapted frame field 246Ч251
Algebraic area 289Ч290 386(Ex.
All-umbilic surface 257Ч259
Alternation rule 27 47 153
angle 44
Angle function 50(Ex. 12) 295(Ex.
Angle, coordinate 210
Angle, exterior 374
Angle, interior 374
Angle, oriented 291
Antipodal mapping 165(Ex. 5)
Antipodal points 182
Arc length 51Ч52 220(Ex.
Arc length function 51
Area 280Ч286
Area form 283Ч284 292 309(Ex.
Area-preserving mapping 295(Ex. 16)
Associated frame field 276Ч277
Asymptotic curves 226Ч227 230
Asymptotic directions 225Ч227
Attitude matrix 46 88
Basis formulas 251
Bending 268 275(Ex.
binomial 57 66 69
Boundary of a 2-segment 170
Boundary of a region 386
Bracket operation 81(Ex. 7) 195(Ex.
Bugle surface 241Ч242 244(Ex. 282Ч283 291
Canonical isomorphism 7Ч8 44 59
Canonical parametrization 238
Cartan, E. 42 92 96 303
Cartesian Plane 306
Cartesian product 187(Ex. 9)
Catenoid 236
Catenoid as minimal surface 236Ч238
Catenoid, Gauss mapping 296(Ex. 20)
Catenoid, Gaussian curvature 236 239
Catenoid, Gaussian curvature, total 287Ч288
Catenoid, local isometry onto 267Ч268
Catenoid, principal curvatures 236
Center of curvature 64(Ex. 6)
circle 59 62
Clairut parametrization 330Ч339
Closed surface in 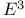 181(Ex. 10) 263n 181(Ex. 10) 263n
Codazzi equations 249 255
Compact surface 176Ч177 259Ч260
Complete surface see "Geodesically complete surface"
Composite function 1
Cone 140(Ex. 5) 231(Ex.
Conformal geometric structure 305Ч306 308(Ex. 312Ч313
Conformal mapping 268Ч271 310
Conformal patch 270(Ex. 7) 279(Ex.
Congruence of curves 116Ч123
Congruence of curves determined by curvature and torsion 117
Congruence of surfaces 189 297Ч303
Conjugate point 353Ч359
Connected surface 176
Connection equations on a surface 248 318
Connection equations on Euclidean space 86 248
Connection forms on a surface 248 272 277 306
Connection forms on Euclidean space 85Ч90
Conoid 233(Ex. 20)
Consistent formula for a mapping 165(Ex. 10)
Coordinate angle 210 220(Ex.
Coordinate expression 143 165(Ex.
Coordinate patch see "Patch"
Coordinate system 158(Ex. 9) 276Ч277
Covariant derivative formula 91(Ex. 5) 189Ч190 321
Covariant derivative on a patch 212 324Ч326
Covariant derivative, Euclidean 77Ч80 116(Ex. 189Ч190
Covariant derivative, intrinsic 318Ч326
Cross product 47Ч49 107 110
Cross-sectional curve 138
Curvature see also "Gaussian curvature"
Curvature of a curve in  65(Ex. 8) 122Ч123 65(Ex. 8) 122Ч123
Curvature of a curve in 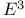 57 66 69 57 66 69
Curve 15
Curve in a surface 144Ч145
Curve plane 61
Curve segment 56(Ex. 10) 167
Curve, closed 169
Curve, coordinate expression for 144
Curve, one-one 20
Curve, periodic 20
Curve, regular 20
Curve, simple closed 151n
Curve, unparametrized 20Ч21
Cylinder 129 231(Ex.
Cylinder parametrization 137 141(Ex.
Cylinder, geodesies 275(Ex. 2) 352(Ex.
Cylindrical frame field 82Ч83
Cylindrical frame field, connection forms 89Ч90
Cylindrical frame field, dual 1-forms 96(Ex. 4)
Cylindrical helix 72Ч75
Darboux 81
Degree of a form 27 152
Degree of a mapping 386(Ex. 6)
Derivative map 35Ч40
Derivative map of a mapping of surfaces 160Ч161 166
Derivative map of a patch 149(Ex. 4)
Derivative map of an isometry 104
Derivative of Euclidean vector field 113
Diffeomorphism 38 40(Ex. 161
Differentiability 4 10 33 143 145Ч146
Differential 23Ч26
Differential form on  156 156
Differential form on 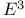 22Ч31 22Ч31
Differential form on a surface 152Ч157
Differential form, closed or exact 157(Ex. 2) 173Ч175
Differential form, pullback 163
Direction 196
Directional derivative 11Ч15 149
Directional derivative, computation of 12 25
Director curve 140
Distribution parameter 232(Ex. 14)
Domain 1
Dot product 42Ч44 46 82 210
Dot product, preserved by isometries 105
Dual 1-forms 91Ч92 248 306
Dupin curves 208(Ex. 5)
E, F, G 140(Ex. 2) 210 317(Ex.
Edge (curve) 170 373 377
Ellipsoid 142(Ex. 10)
Ellipsoid isometry group 365
Ellipsoid, Euclidean symmetries 303(Ex. 10)
Ellipsoid, Gaussian curvature 217Ч219 222(Ex.
Ellipsoid, umbilics 223(Ex. 23)
Elliptic hyperboloid 142(Ex. 10) 221Ч222
Elliptic paraboloid 142(Ex. 11) 220(Ex.
Enneper's surface 221(Ex. 12)
Euclid 335Ч336
Euclidean coordinate functions 9 15 24 33 53
Euclidean distance 43 49(Ex.
Euclidean geometry 112 304Ч305 308
Euclidean plane 5 305 335Ч336
Euclidean plane, local isometries 363Ч364
Euclidean space 3 5
Euclidean symmetry 302Ч303 365
Euclidean vector field 147
Euler Ч Poincare characteristic 378Ч380
Euler's formula 201
Evolute 75(Ex. 15)
Exterior angle 374
Exterior derivative 28Ч31 31Ч32 154Ч155
| Faces 377
Flat surface 207 231(Ex. 263(Ex.
Flat torus 316 317(Ex. 370(Ex.
Flat torus imbedding in 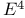 369 369
Focal point 362
FORM see "Differential form"
Frame 44
Frame field on 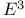 82 82
Frame field on a curve 120
Frame field on surface 251 306
Frame field, adapted 246
Frame field, natural 9
Frame field, principal 254
Frame field, transferred 272Ч273
Frame-homogeneous surface 366
frenet 81
Frenet apparatus 63(Ex. 1) 71
Frenet approximation 60Ч61 65(Ex.
Frenet formulas 58 67
Frenet frame field 57
Function 1Ч2
Function, one-to-one 2
Function, onto 2
Gauss 245 274 303
Gauss equation 249
Gauss mapping 194Ч195 289Ч291
Gauss Ч Bonnet formula 375Ч377 383Ч385 388(Ex.
Gauss Ч Bonnet theorem 380Ч383 387(Ex.
Gaussian curvature 203Ч207 310Ч312 see
Gaussian curvature in Jacobi equation 355
Gaussian curvature sign 204Ч205
Gaussian curvature, differentiability 206
Gaussian curvature, formulas, explicit 206 212 217 253 278
Gaussian curvature, formulas, implicit 205 252
Gaussian curvature, Gauss mapping and 289
Gaussian curvature, geodesic curvature an 339(Ex. 19)
Gaussian curvature, geodesies and 355Ч358
Gaussian curvature, holonomy and 325(Ex. 5)
Gaussian curvature, interval 275(Ex. 3)
Gaussian curvature, isometric invariance 273Ч275
Gaussian curvature, polar circles and 359Ч360
Gaussian curvature, polar discs and 361(Ex. 2)
Gaussian curvature, principal curvatures and 203
Gaussian curvature, shape operator and 203
Geodesic curvature 230(Ex. 7) 329Ч330 337(Ex.
Geodesic curvature, total 372Ч373
Geodesic polar mapping 340Ч341
Geodesic polar parametrization 341Ч344
Geodesically complete surface 263n 328Ч329
Geodesically complete surface, shortest geodesic segments in 348
Geodesies 228Ч232 326Ч363 see
Geodesies coordinate formulas 327 333
Geodesies on surfaces of nonpositive curvature 358 388(EX.
Geodesies, closed 232(Ex. 13)
Geodesies, existence and uniqueness 328
Geodesies, frame fields and 250(Ex. 1)
Geodesies, length-minimizing properties 339Ч359
Geodesies, preserved by (local) isometries 275(Ex. 1) 326 362Ч363
Geodesies, spreading of 353Ч354
Geographical patch 134Ч135
Geometric surface 305 308
Gradient 32(Ex. 8) 50(Ex.
Gradient as normal vector field 148 216
Group 103
Group, euclidean 103
Group, Euclidean symmetry 302(Ex. 7)
Group, isometry 365
Group, orthogonal 104
Halmos symbol 9
Handle 379 382
Hausdorff axiom 186(Ex. 5) 345n
Helicoid 141(Ex. 7)
Helicoid as ruled minimal surface 227 233(Ex.
Helicoid, Gauss mapping 296(Ex. 20)
Helicoid, local isometries 267 275Ч276
Helicoid, patch computations for 213Ч214
Helix 15Ч16 58Ч59 119
Hilbert's Lemma 261
Hilbert's theorem 263
Holonomy 323Ч325
Holonomy angle 323
Holonomy, Gaussian curvature and 325(Ex. 5)
Homogeneous surface 366Ч368 370
Homotopic to constant 175
Hopf 386 389
Hyperbolic paraboloid 143(Ex. 12) 220(Ex.
Hyperbolic plane 315Ч316
Hyperbolic plane of pseudo-radius r 317(Ex. 4)
Hyperbolic plane, geodesic completeness 351(Ex. 1)
Hyperbolic plane, geodesies 334Ч336
Hyperbolic plane, isometries 371(Ex. 14)
Hyperbolic plane, local isometries 364
Identity mapping 99
Image 1
Image curve 33
Immersed surface 187(Ex. 10) 219 368
Improper integral 285Ч286
Induced inner product 308 313
Initial velocity 21(Ex. 6)
Inner product 304 316Ч317
Integral curve 186Ч187
Integral of function 286 292(Ex.
Integration of differential forms 167Ч176 283Ч297
Integration of differential forms, 1-forms over 1-segments 167Ч169 172Ч173
Integration of differential forms, 1-forms over oriented regions 285 292(Ex.
Integration of differential forms, 2-forms over 2-segments 169Ч172 174(Ex. 297(Ex.
Interior angle 374
Intrinsic distance 264Ч265 269(Ex. 351
Intrinsic geometry 271 304
Inverse function 2
Inverse function theorem 39 161Ч162
Isometric imbedding 367Ч369
Isometric immersion 367Ч369
Isometric invariant 271 304
Isometric surfaces 265
Isometry group 365
Isometry of Euclidean space 98Ч111
Isometry of Euclidean space, decomposition theorem 101
Isometry of Euclidean space, derivative map 104
Isometry of Euclidean space, determined by frames 105
Isometry of surfaces 263Ч266 270Ч275 306
Isometry of surfaces, Euclidean isometries and 297Ч299
Isometry of surfaces, isometric immersions and 367
Isothermal coordinates 279(Ex. 2)
J (rotation operator) 309(Ex. 5)
Jacobi equation 355Ч357 361(Ex.
Jacobian 288 294(Ex. 296(Ex.
Jacobian matrix 37
Kronecker delta 23 45
Lagrange identity 209(Ex. 6)
Law of Cosines 370(Ex. 10)
Leibnizian property 13
Length of a curve segment see "Arc length"
Length of a vector 44
Levi Civita 322
Liebmann's Theorem 262
Line of curvature see "Principal curve"
Liouville parametrization 339(Ex. 18)
Local isometry 265Ч270 362Ч365
Local isometry determined by frames 363
Local isometry of constant curvature surfaces 364
Local isometry, criteria for 266 269(Ex. 270(Ex.
Local minimization of arc length 352Ч358
Manifold 184Ч187
Mapping of Euclidean spaces 32Ч41
Mapping of surfaces 158Ч166
Mean curvature 203 205Ч208 212 217 252
Mercator projection 271(Ex. 13)
Metric tensor 305
Minimal surface 207 275(Ex.
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте



 , m, n
, m, n