|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Olver F.W.J. Ч Asymptotics and Special Functions |
|
|
 |
| ѕредметный указатель |
Moore, W. K. 336
Moriguchi, H. 366
Morse, P. M. 501
Mott, N. F. 433
Muckenhoupt, B. 446
Muldoon, M. E. 338 434
Murnaghan, F. D. 544
Nakano, M. 517
National Bureau of Standards (N.B.S.) 64 168 277 282 321 526 543
National Physical Laboratory (N.P.L.) 541
Natterer, F 228
Navot, I. 321
Nayfeh, A. H. 433 434
Newton, R. G. 479
Ng, E. W. 64
Nicolaenko, B. 317
Ninham, B. W. 321
Nishimoto, T. 517
Norlund, N. E. 282
Nosova, L. N. 434
Oberhettinger, F. 278 321 361
Olver, F. W. J. 76 92 94 104 105 109 112 136 137 138 228 250 266 269 276 277 278 302 313 315 334 373 381 391 405 413 416 418 433 434 462 477 478 479 496 517 518
Order relations 4Ч11 29
Order relations, implied constants 5 7
Orthogonal polynomials 46Ч48 65 Jacobi Laguerre Legendre
Orthogonal polynomials, Christoffel Ч Darboux formula 48
Orthogonal polynomials, classical 48Ч52
Orthogonal polynomials, differential equations for 50 52
Orthogonal polynomials, generating functions 51 52
Orthogonal polynomials, Gram Ч Schmidt process 47
Orthogonal polynomials, normalization 46 47
Orthogonal polynomials, recurrence relations 47 50 52
Orthogonal polynomials, RodriguesТ formulas 49
Orthogonal polynomials, zeros 47
Orthonormal polynomials 46
Papperitz equation 157
Parabolic cylinder functions 206Ч207 (see also УWeberТs differential equationФ)
Parabolic cylinder functions of large order 207Ч208 228 334
Parabolic cylinder functions, integral representation 208
Parabolic cylinder functions, notation 207 228
Parabolic cylinder functions, relation to confluent hypergeometric functions 259
Path 29
Phase function 394 436 456
Phase principle 247Ч248
Phase principle, example 252Ч253
Phase shift 210 449Ч451 479
Phase-integral method 517
Pike, E. R. 228 478 518
Pittnauer, F. 30
Po1ya, G. 105
Pochhammer, L. 38
PochhammerТs notation 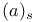 159 159
Poincare, H. 4 16 27 105
PoissonТs summation formula 306
Pollak, H. O. 309
Potential barrier 501 517Ч518
Prime numbers 63Ч64
Principal curves 487 504 518 521
Principal directions 509
Principal subdomains 504
Principle of the argument see УPhase principleФ
Progressive paths 222 266 367
Progressive paths, choice of 224Ч227 508
Proper degree 46
PrymТs function 45
PrymТs function, asymptotic expansion of 295
PrymТs function, GaussТs formula 40
Psi function 39
Rayleigh (Lord) 228 516
Redheffer, R. M. 31 123 128 146 247
Reflection coefficient 503 515
Region 7
Regular arc 29 147
Regular singularities see УSingularities of differential equationsФ
Reid, W. H. 368 518
Remainder term 2
Remainder term, optimum 522
Reudink, D. O. J. 251 321
Reversion of series 21 Ч22 313Ч315
Riccati equation 192
Rice, S. O. 361
Riedel, R. 361
Riekstina, V. 321
Riekstins, E. 29 30 317 361
Riemann equation 157
Riemann, B. 61 63 64 65 137 157 158
Riemann-Lebesgue lemma 73 99 104
Ritchie, R. H. 44
Ritt, J. R 9 29
Roberts, J. H. 94
Robin, L. 189 479
Rosser, J. B. 542 543
Royal Society (R.S.) 247 278 434
Rubin, R, J. 329
Sabatier, P. C 479
Saddle point 126 136
Saddle point, coinciding with pole 130 528
Saddle-point method 138
Saw-tooth function 279
Scattering theory 208 433 449
Schindler, S. 479
Schlesinger, L. 391
Schmidt, H. 30
Schoenfeld, L. 329
Schrodinger equation 449
Schwarzian derivative 191
SchwarzТs principle of symmetry 128
Scorer, R. S. 431
ScorerТs functions 332 430Ч432 434
Sectional continuity 73
Semiconvergent series 3
Shadow zones 223Ч224 417
Shadow zones, necessity of 236
Shanks, D. 544
Shepp, L. 309
Sibuya, Y. 277
Sine integrals 41Ч43
Sine integrals, asymptotic expansion of 67 320
Sine integrals, Laplace transform of 43
Singularities of differential equations 148 (see also УDifferential equations Irregular LG
Singularities of differential equations at infinity 153Ч154
Singularities of differential equations, connection formulas at 480Ч482
Singularities of differential equations, expansions at 148Ч150 153
| Singularities of differential equations, exponents 149 153 482
Singularities of differential equations, exponents differing by integer 150Ч152
Singularities of differential equations, indices 149
Singularities of differential equations, indicial equation 149
Singularities of differential equations, numerically satisfactory solutions at 154Ч155
Singularities of differential equations, rank 148 154
Singularities of differential equations, regular 148 153
Singularity, extraction of singular part 120
Skovgaard, H. 412 446
Slater, L. J. 189 277 278
Slowly convergent series 544
Snow, C 189
Soni, K. 361
Soni, R. P. 361
Spira, R. 294
Stationary phase see УMethod of stationary phaseФ
Stationary points 96
Staton, J. 44
Steepest descents see УMethod of steepest descentsФ
Steffensen, J. F. 69
Steinig, J. 278
Stenger, F. 278 361 391
Stieltjes transform 92
Stieltjes, T. J. 294 543
StirlingТs formula 88
StirlingТs series 293Ч295
Stokes lines 518 521
Stokes, G. G. 105 240 518 543
StokesТ phenomenon 240
StruveТs equation 274
StruveТs equation, numerically satisfactory solutions 276Ч277
StruveТs function 275Ч277 278
Successive approximations, method of 141
Summation by parts 296 540
Sums of powers of integers 283 284 292
Swanson, C. A. 412 446 478
Sweeney, D. W. 64
Szego, P. 434
Szego.G. 65 105 137 479
Tail of power series 7
Taylor coefficients, asymptotic expansion of 309Ч315 329Ч331
Tetragamma function 39
Thome, L. W. 230
Thorne, R. C 223 391
Thurber, J. K. 317
Titchmarsh, E. C. 26 54 65 161 306 498
Tolstov, G. P. 315
Total variation see УVariationФ
Transcendental equations, asymptotic solution of complex variables 14Ч16 29
Transcendental equations, asymptotic solution of real variables 11Ч14 29
Transition points 192 362 474
Transmission coefficient 503 515
Tricomi, F. O. 120 278
Trigamma function 39
Truncation error 2
Tumarkin, S. A. 434
Turning points 192 398
Turning points, multiple 363 474Ч478
Turning-point problems see УConnection formulasФ
Turning-point problems, asymptotic solutions for complex variables 416Ч419
Turning-point problems, asymptotic solutions for real variables 397Ч401 408Ч413 426Ч429
Turning-point problems, coefficients in asymptotic solutions 409Ч410 412Ч413 419
Turning-point problems, error bounds for asymptotic solutions 399Ч400 410 417Ч418
Turning-point problems, first approximation 397Ч400
Turning-point problems, formal series solutions 409 427Ч428
Turning-point problems, history 433 516Ч517
Turning-point problems, inhomogeneous equations 429Ч430 434
Turning-point problems, more general form 426Ч429 474Ч478
Turning-point problems, stationary values of solutions 408
Turning-point problems, zeros of solutions 405Ч408
Turrittin, H. L. 391 433
Ursell, F. 137 351 358 361
van der Corput, J. G. 27 29 68 105
van der Waerden, B. L. 361
van Wijngaarden, A. 544
Variation 27Ч29
Variation of negative power 225Ч227
Variation, convergence at singularity 200Ч202
Variational operator 27
Wainger, S. 446
Wasow, W. 278 391 433 517
Watson, G. N. 30 64 71 105 153 162 189 228 245 249 254 268 269 277 278 294 313 315 321 340 342 438 444 543 544
WatsonТs lemma for loop integrals 120
WatsonТs lemma, complex variables 112Ч113
WatsonТs lemma, error bounds for 89Ч90 105 114Ч116
WatsonТs lemma, estimation of remainder term 544
WatsonТs lemma, history 104
WatsonТs lemma, real variables 71
Wave penetration 501 513 517Ч518
Weber parabolic cylinder functions see УParabolic cylinder functionsФ
Weber, H. F. 103
WeberТs differential equation 147 206
WeberТs differential equation, connection formulas for solutions 516
WeberТs differential equation, zeros of solutions 214
WeberТs function 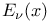 103 103
WeberТs function 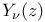 242 (see also УBessel functionsФ) 242 (see also УBessel functionsФ)
Weight functions 46 394 415 437 454
Wentzel, G. 228
Whittaker functions 260 (see also УConfluent hypergeometric functions WhittakerТs
Whittaker functions of large argument 260 266
Whittaker functions with large k 412 446Ч447
Whittaker functions with large m 260Ч261 278 368 401Ч402
Whittaker functions, zeros 452Ч453
Whittaker, E. T. 64 153 189 207 294 321
WhittakerТs equation 260
WhittakerТs equation, connection formulas for solutions 261 262 516
Widder, D. V. 68 105
Wilkins, J. E. 340
WKB approximation see УLG approximationФ
WKB or WKBJ method 228 517
Wong, R. 104 317
Wrench, J. W., Jr. 88 544
Wright, E. M. 321
wronskian 142
Wyman, M. 30 104 138 317 321
Wynn, P. 543 544
Zeros, asymptotic approximations from LG approximation 211Ч214 228
Zeros, asymptotic approximations in Case III 451Ч453
Zeros, asymptotic approximations in turning-point problems 405Ч408
Zeta function 61Ч64 65
Zeta function of integer argument 63 283
Zeta function, JensenТs formula 292
Zwaan, A. 517
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте