|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Gorenstein D., Lyons R., Solomon R. Ч Classification of the Finite Simple Groups (Vol. 1) |
|
|
 |
| ѕредметный указатель |
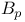 -property 24 28 40Ч41 62 123 127 -property 24 28 40Ч41 62 123 127
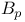 -property, partial 30 36 42 64 -property, partial 30 36 42 64
 59 62Ч63 67Ч68 76Ч77 83 59 62Ч63 67Ч68 76Ч77 83
 , ,  , ,  121 121
 , ,  , , 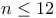 109 114 109 114
 , G* of Lie type, of large Lie rank 116 121 , G* of Lie type, of large Lie rank 116 121
 , G* of Lie type, of small Lie rank, of characteristic 2 95Ч96 115 , G* of Lie type, of small Lie rank, of characteristic 2 95Ч96 115
 , G* of Lie type, of small Lie rank, of characteristic 2 and Lie rank 1 95Ч96 , G* of Lie type, of small Lie rank, of characteristic 2 and Lie rank 1 95Ч96
 , G* of Lie type, of small Lie rank, of odd characteristic 110 112 113 , G* of Lie type, of small Lie rank, of odd characteristic 110 112 113
 , G* sporadic 109 , G* sporadic 109
 -balance 21 127Ч128 -balance 21 127Ч128
 -balance, analogue for near components 96 -balance, analogue for near components 96
 -balance, analogue for two primes 134 -balance, analogue for two primes 134
 -balance 127Ч128 -balance 127Ч128
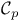 -groups 54 57 81 99Ч101 -groups 54 57 81 99Ч101
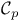 -groups as pumpups 101Ч102 -groups as pumpups 101Ч102
 -groups 95 100 -groups 95 100
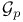 -groups 57Ч58 63 103 -groups 57Ч58 63 103
 -groups 5 12 -groups 5 12
 -groups, theory of 12 45 48 75Ч76 138Ч139 -groups, theory of 12 45 48 75Ч76 138Ч139
 -proper group 12 75Ч76 79 -proper group 12 75Ч76 79
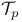 -groups 57 102Ч103 129 -groups 57 102Ч103 129
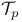 -groups as pumpups 101Ч103 -groups as pumpups 101Ч103
 37Ч38 52Ч53 55 58Ч59 61 82 86 92Ч93 105 131 37Ч38 52Ч53 55 58Ч59 61 82 86 92Ч93 105 131
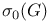 58Ч61 83 86 105Ч106 116 118 131 58Ч61 83 86 105Ч106 116 118 131
(B, N)-pair 34
(B, N)-pair, split 34
(B, N)-pair, split, recognition of rank 1 36Ч37 39 49 50 63 113 138
(B, N)-pair, split, recognition of rank 2 37 63 111 113 115 137Ч138
2-central involution 88
2-local p-rank 135
3/2-balanced functor 43 64Ч65
Algebraic automorphism 118 121
Alperin, J. 39 41
Amalgam method 5Ч6 26 39 41 43 60Ч61 105 131Ч133
Amalgam method, associated graph 132Ч133
Artin, E. 11
Aschbacher  -block 39 41 53 -block 39 41 53
Aschbacher, M. 18 30 37 39Ч43 45Ч48 50 53 89 99 125 129 130
Associated  -balanced functor 125 -balanced functor 125
Atlas of Finite Groups 45 50 139
Background references 47Ч50 140
Background results 59 63 79 87 104 118
Background results, listed 44Ч50
Balance, k-balance see УGroupФ (see also УSignalizer functorФ)
Bar convention 18 139
Baumann, B. 39 131
Bender method 30 38 43 60 62 104 110 123 134
Bender, H. 16Ч17 48Ч50 123
Blackburn, N. 46 47
Bombieri, E. 49
Borel, A. 25
Brauer Ч Suzuki theory of exceptional characters 38 135
Brauer, R. 51
Brauer, R., theory of blocks 38 46 50 62
Brauer, R., theory of blocks, defect groups of 2-rank at most 3 50
Building 34 73 138
Burnside, W. 29Ч30
Carter, R. 45 47
Centralizer of element of odd prime order p 35 41 42 51 54Ч56
Centralizer of element of prime order p 108
Centralizer of involution 11 27ff. 35 39 41Ч43 51 52 54 61Ч62
Centralizer of involution pattern 46 59 77 109Ч110
Centralizer of semisimple element 51 54Ч56
Character theory 31 46 50 60 62 104 108 135Ч137
Character theory, ordinary vs.modular 50
Chevalley groups see УGroups of Lie typeФ
Chevalley, C. 3
Chief factor, series 13Ч14
Classical groups 6ff. (see also УGroups of Lie typeФ)
Classification Grid 79 83 85 99Ч121
Classification Theorem see УTheoremsФ
Classification Theorem, Theorems  - - 104Ч106 104Ч106
Component 17 51 81
Component, solvable 51 67 109
Component, standard 53 91Ч92
Component, terminal 23 42 53 81 90Ч92 108
Composition factor, length, series 12
Composition factor, length, series, A-composition factor, length, series 13
Computer 35 45 68
Control of 2-locals 129
Control of fusion 87 122
Control of rank 1 or 2 fusion 91Ч92
Conway, J. 11
Core 20 (see also Уp'-coreФ)
Core, elimination 40 43 60 110Ч111
Covering group 16
Covering group, notation for 101
Covering group, universal 17 33
Curtis, C. 35
Das, K.M. 35 71
Delgado, A. 37
Dickson, L. 7
Dieudonne, J. 47
Double transitivity of Suzuki type 95Ч96
Enguehard, M. 49Ч50
Expository references 47 141Ч146
Feit, W. 46 47 48 107Ч108
Finkelstein, L. 35
Fischer, B. 11 39Ч40
Fischer, B., transpositions 11 39
Fitting length, series 19
Fitting subgroup 16
Fitting subgroup, generalized Fitting subgroup 17 123
Foote, R. 5 38 53 98
Frattini subgroup 18
Frobenius group 107
Frobenius, G. 29
Frohardt, D. 35
Fusion 29 60 62 63 104 120 122
Fusion, extremal conjugation 122
General local group theory 45Ч48
Generalized Fitting subgroup 17 123
Geometry associated with a finite group 35 73Ч74
Gilman, R. 35 39 41
Glauberman, G. 21 38 39 48Ч50 124 130
Goldschmidt, D. 39 43 49 125
Gomi, K. 37
Gorenstein, D. 29 38 39 41 46 47Ч50 99 124 126 127
Griess, R.L. 17 35 41 45
Group of Lie type see УGroups of Lie typeФ
Group order formulas 50 135Ч137
Group, almost simple 18
Group, alternating see УAlternating groupФ
Group, covering 16
Group, covering, notation for 101
Group, covering, universal 17 33
Group, DC-proper 12 21
Group, k-balanced 124Ч125 129
Group, k-balanced,  -balanced 125 129 -balanced 125 129
Group, k-balanced, locally balanced 128
Group, k-balanced, locally k-balanced,  -balanced 126 -balanced 126
Group, k-balanced, weakly /c-balanced, weakly locally /c-balanced 124 126
Group, nilpotent 15Ч16
Group, p-constrained 20
Group, p-solvable 24
Group, perfect 16
Group, quasisimple 16 53 81
Group, semisimple 16
Group, simple, table of 8Ч10
Group, solvable 13 16 73Ч74
Group, sporadic see УSporadic groupФ
Groups in 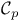 see У see У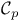 -groupsФ -groupsФ
Groups in  see У see У -groupsФ -groupsФ
Groups in 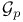 see У see У -groupsФ -groupsФ
Groups in  see У see У -groupsФ -groupsФ
Groups of 3/2-balanced type 120Ч121
Groups of characteristic 2-type 55 98 116Ч117
Groups of characteristic p-type 25 116Ч117
Groups of even type 36 53Ч55 57Ч59 81 87 98
| Groups of even type, groups of restricted even type 58Ч59 82 90 95 99 102 106
Groups of G*-type (G* a target group), groups of 2-amalgam G*-type,  115 115
Groups of G*-type (G* a target group), groups of 2-central G*-type,  of Lie type 111 of Lie type 111
Groups of G*-type (G* a target group), groups of 2-maximal G*-type mod cores,  111 111
Groups of G*-type (G* a target group), groups of 2-terminal G*-type,  110 110
Groups of G*-type (G* a target group), groups of doubly transitive G*-type,  , ,  , , 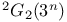 112 112
Groups of generic type 35Ч36 55Ч59 63Ч68 79 106 118Ч121
Groups of generic type, groups of  -generic type 57Ч59 61 -generic type 57Ч59 61
Groups of generic type, groups of generic even type 106
Groups of generic type, groups of generic odd type 106
Groups of generic type, groups of semisimple type 121
Groups of generic type, groups of semisimple type, groups of proper semisimple type 121
Groups of GF(2)-type 40Ч43
Groups of large sporadic type 37Ч38 61
Groups of Lie type 6ff. 32ff. 45Ч49
Groups of Lie type, as (B, N)-pair 34
Groups of Lie type, Borel subgroup of 33
Groups of Lie type, Bruhat decomposition of 34
Groups of Lie type, Cartan subgroup of 33
Groups of Lie type, Chevalley commutator formula 32
Groups of Lie type, Chevalley group 3
Groups of Lie type, Dynkin diagram of 32 71
Groups of Lie type, generation of 48 65Ч66
Groups of Lie type, Lie rank of twisted 32
Groups of Lie type, Lie rank of untwisted 32
Groups of Lie type, monomial subgroup of 34
Groups of Lie type, monomial subgroup of, reduced 34
Groups of Lie type, parabolic subgroup of 26 62
Groups of Lie type, rank 1, subgroup of 33 35 71
Groups of Lie type, Ree group 3 10 49 50
Groups of Lie type, root subgroup of 32
Groups of Lie type, root system 32
Groups of Lie type, root system, fundamental system 32
Groups of Lie type, Schur multiplier of 45 48
Groups of Lie type, semisimple element of 51
Groups of Lie type, Steinberg presentation of, relations 33
Groups of Lie type, Steinberg variation 7
Groups of Lie type, Suzuki group 3 10
Groups of Lie type, universal version of 33
Groups of Lie type, universal version of, and universal covering group 33
Groups of Lie type, untwisted see УChevalley groupФ
Groups of Lie type, Weyl group of 33 35
Groups of odd order, groups of  -uniqueness type 107 -uniqueness type 107
Groups of odd order, groups of odd order uniqueness type 107
Groups of odd order, groups of {p, q}-parabolic type 107Ч108
Groups of odd type 58Ч59 81 86
Groups of quasithin type 82 105 114Ч116
Groups of quasithin type, groups of 2-amalgam type 114Ч115
Groups of quasithin type, thin subcase 37 41
Groups of special type 36Ч38 58Ч61 79 103Ч106 106Ч108 110Ч118
Groups of special type, groups of 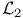 -special type 58Ч60 103Ч104 -special type 58Ч60 103Ч104
Groups of special type, groups of 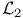 -special type, groups of -special type, groups of  -type 61Ч63 83 104 110Ч113 123 -type 61Ч63 83 104 110Ч113 123
Groups of special type, groups of 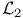 -special type, groups of -special type, groups of  -type, -type,  -subcase 61Ч63 -subcase 61Ч63
Groups of special type, groups of 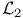 -special type, groups of -special type, groups of  -type 104 114 -type 104 114
Groups of special type, groups of 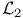 -special type, groups of -special type, groups of  -type, groups of 2-terminal -type, groups of 2-terminal  -type 114 -type 114
Groups of special type, groups of  -special type 58Ч60 105Ч106 -special type 58Ч60 105Ч106
Groups of special type, groups of  -special type, groups of -special type, groups of  -type 105 116Ч118 -type 105 116Ч118
Groups of special type, groups of  -special type, groups of -special type, groups of  -type 106 118 -type 106 118
Groups of special type, groups of  -special type, groups of -special type, groups of  -type, groups of p-terminal -type, groups of p-terminal  -type 118 -type 118
Groups of special type, groups of  -special type, groups of quasisymplectic -special type, groups of quasisymplectic  -type 117 -type 117
Groups of special type, groups of  -special type, groups of wide -special type, groups of wide  -type 116 133Ч135 -type 116 133Ч135
Groups of special type, groups of  -uniqueness type 107 -uniqueness type 107
Groups of special type, groups of odd order uniqueness type 107
Groups of special type, groups of special even type 105 106 114Ч118
Groups of special type, groups of special odd type 103Ч104 106Ч108 110Ч114
Groups of special type, groups of {p, q}-parabolic type 107Ч108
Groups with specified 2-structure, groups of 2-rank at most 2 36 135
Groups with specified 2-structure, groups of 2-rank at most 3 50
Groups with specified 2-structure, groups with semidihedral or wreathed Sylow 2-subgroups 76 136Ч137
Groups with specified 2-structure, groups with semidihedral or wreathed Sylow 2-subgroups, Brauer group order formula for regular groups 137
Groups with specified 2-structure, groups with semidihedral or wreathed Sylow 2-subgroups, characteristic power 136Ч137
Hall  -subgroup 46 -subgroup 46
Hall, M. 11
Hall, P. 16 25
Harada, K. 11 39 89
Hayashi, M. 37
Holt, D. 89
Hunt, D. 49
Huppert, B. 46 47
Identification of simple groups see УRecognition of simple groupsФ
Involution fusion pattern 46 60 62 109 110
Isaacs, I.M. 46 47
Isomorphism question 11
Janko, Z. 11 49
Klinger Ч Mason method 61 105 116Ч118
Klinger, K. 38 116Ч117
Layer 17 (see also Уp-layerФ)
Leech lattice 11 35
Linear group 18
Local subgroup 19 (see also Уp-local subgroupФ)
Local subgroup, general structure of 27Ч28
Lyons, R. 41 48Ч50 99
Mason, G. 37Ч38 41 116Ч117
Mathieu, E. 11
Maximal subgroup 60 62Ч63 123
McBride, P. 30 49 124
Meierfrankenfeld, U. 130
Modules, failure of factorization 26 130 133
Modules, quadratic 25Ч26 123 130
N-group 38Ч39 73
Near component 50 96Ч97 129Ч131 133
Near component, alternating 96Ч97 130
Near component, associated module of 96
Near component, linear 96Ч97 130 131
Near component, standard 98
Near component, type 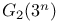 97 97
Neighbor 42 64Ч65 71 119
Neighbor, semisimple 64Ч65
Neighborhood 55Ч58 59 63Ч68 118Ч120
Neighborhood,  - 61 117 - 61 117
Neighborhood, base of 119
Neighborhood, example of 56Ч57 68Ч70
Neighborhood, level 66 121
Neighborhood, span of 66Ч67 121
Neighborhood, vertical 65 76Ч77 118Ч120
Niles, R. 39 50
Notation 139
Odlyzko, A. 49
Outer automorphisms 18
Overall strategy of proof 35Ч38 42Ч43
OТNan, M. 18 45 49 138
p'-core 19 (see also УCoreФ)
p'-core, elimination 23Ч24 37 61 120
p'-core, embedding of pТ-core of p-local subgroups 21 127Ч128
p-central p-element 101
p-component 20
p-component preuniqueness hypothesis 91Ч92
p-component preuniqueness subgroup see УUniqueness subgroupsФ
p-component uniqueness theorems 30Ч31 38 53 65 90Ч92 118
p-component, p-terminal 22ff. 63 108Ч109
p-component, p-terminal, pumping up to 23 108Ч109
p-component, solvable 64 109
p-layer 20
p-local subgroup 19
p-local subgroup, embedding of p'-core of 20Ч21 127Ч128
p-local subgroup, embedding of p-layer of 21Ч24 127
p-source 64
Parts of the series 4Ч5 59 77Ч78 80
Parts of the series, Part II 38 52Ч53
Parts of the series, Part III 36
Parts of the series, Part IV 37
Parts of the series, Part V 37 38
perfect central extension see УCovering groupФ
Permutation group 18 35
Permutation group, doubly transitive 74 112Ч113 138
Permutation group, doubly transitive of Suzuki type 95Ч96
Permutation group, doubly transitive, split (B,N)-pair of rank 1 95Ч96 112Ч113 138
Permutation group, highly transitive 11 35
Permutation group, of rank 3 11
Permutation group, representation as 31
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте



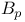 -property
-property 
 ,
, 
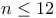
 -balance
-balance  -balance
-balance 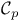 -groups
-groups  -groups
-groups 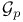 -groups
-groups  -groups
-groups 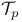 -groups
-groups 
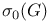
 -block
-block  -balanced functor
-balanced functor  -
-

 of Lie type
of Lie type 
 ,
, 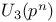 ,
, 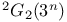
 -generic type
-generic type  -uniqueness type
-uniqueness type 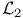 -special type
-special type  -type
-type  -subcase
-subcase  -type
-type  -type
-type  -type
-type  -subgroup
-subgroup 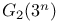
 -
-