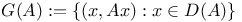|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Zeidler E. — Applied Functional Analysis: Applications to Mathematical Physics |
|
|
 |
| Предметный указатель |
 , inner product on the Sobolev space , inner product on the Sobolev space 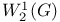 , , 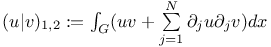 120 120
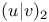 , inner product on the Lebesgue spaces , inner product on the Lebesgue spaces 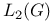 and and  , , 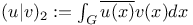 114 114
 , energetic inner product 273 , energetic inner product 273
 -automorphism 358 -automorphism 358
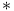 -Homomorphism 358 -Homomorphism 358
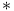 -isomorphism 358 -isomorphism 358
 , operator from the set S into the set Y, where , operator from the set S into the set Y, where 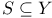 17 17
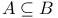 , the operator B is an extension of the operator A 260 , the operator B is an extension of the operator A 260
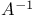 , inverse operator to A 17 , inverse operator to A 17
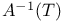 , preimage of the set T, , preimage of the set T, 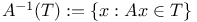 17 17
![$C^{k}[a, b]$](/math_tex/cd58d9158fbf4f4d721a3c4b7264057682.gif) , , 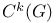 , ,  , ,  , , 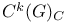 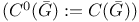 , spaces of smooth functions 96 116 , spaces of smooth functions 96 116
![$C^{\alpha}[a, b]$](/math_tex/a31066c19ba0d0e7af04e7c5416124d082.gif) , , ![$C^{k, \alpha}[a, b]$](/math_tex/2ef3fe6160ab361474d2c3817655983e82.gif) , ,  , , 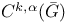 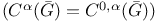 , spaces of Hoelder continuous functions 95ff , spaces of Hoelder continuous functions 95ff
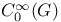 (or (or 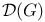 ), ),  , spaces of smooth functions 116 214 , spaces of smooth functions 116 214
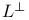 , orthogonal complement to the linear subspace L 165 , orthogonal complement to the linear subspace L 165
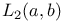 , , 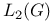 , , 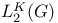 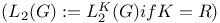 , spaces of integrable functions (Lebesgue spaces) 130 114 , spaces of integrable functions (Lebesgue spaces) 130 114
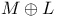 , orthogonal direct sum ( , orthogonal direct sum (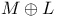 , where , where 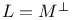 ) 165 ) 165
 , derivative of an operator function u = u(t) at time t 80 , derivative of an operator function u = u(t) at time t 80
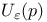 , ,  -neighborhood of the point p in a normed space, -neighborhood of the point p in a normed space,  15 15
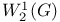 , , 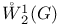 , Sobolev spaces 131 132 , Sobolev spaces 131 132
 , tensor product 224 , tensor product 224
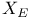 , energetic space 273 , energetic space 273
 , complexification of the linear space X 98 , complexification of the linear space X 98
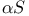 , the product , the product 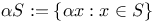 , , 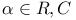 7 7
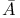 , closure of the linear operator A 415 , closure of the linear operator A 415
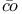 S, closed convex hull of the set S 47 S, closed convex hull of the set S 47
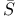 , closure of the set S 31 , closure of the set S 31
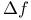 , Laplacian, , Laplacian, 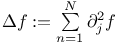 125 125
 , the delta distribution 161 , the delta distribution 161
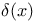 , the Dirac delta function 158 , the Dirac delta function 158
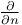 , derivative in the direction of the exterior normal 181 , derivative in the direction of the exterior normal 181
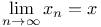 (or (or 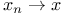 as as 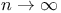 ), the sequence ), the sequence 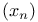 converges to the point x 9 converges to the point x 9
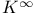 , , 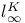 , , 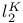 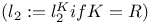 , spaces of sequences 95 177 , spaces of sequences 95 177
 , , 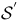 , spaces of distributions 160 219 , spaces of distributions 160 219
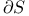 , boundary of the set S 31 , boundary of the set S 31
 , , 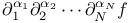 , where , where 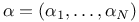 (the classical symbols are also used for the derivatives of generalized functions) 159 (the classical symbols are also used for the derivatives of generalized functions) 159
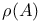 , resolvent set of the linear operator A 83 , resolvent set of the linear operator A 83
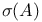 , spectrum of the linear operator A 83 , spectrum of the linear operator A 83
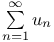 , infinite series in a Banach space 76 , infinite series in a Banach space 76
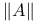 , norm of the linear operator A 70 , norm of the linear operator A 70
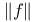 , norm of the functional f 75 , norm of the functional f 75
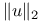 , norm on the Lebesgue spaces , norm on the Lebesgue spaces 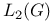 and and  , ,  114 114
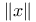 , norm of x 7 , norm of x 7
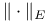 , energetic norm 273 , energetic norm 273
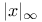 , special norm, , special norm,  11 11
 , the sum , the sum  159 159
(x|y), inner product 105
<x|y>, Euclidean inner product,  ( (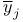 conjugate complex number to conjugate complex number to 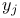 ) 109 ) 109
A posteriori error estimate 19
A priori error estimate 19
A priori estimates 64
A*, adjoint operator to the linear operator A 263
AB (or  ), the product of the operators A and B, (AB)(u):=A(Bu) 28 ), the product of the operators A and B, (AB)(u):=A(Bu) 28
Absolute continuity 437
Absolute integrability 435
Absolute temperature 352
Absolutely convergent 76
Abstract boundary-eigenvalue problem 255
Abstract boundary-value problem 254
Abstract Dirichlet problem 282
Abstract Fourier series 200
Abstract heat equation 255 306
Abstract Schroedinger equation 256 323
Abstract setting for quantum mechanics 327
Abstract setting of quantum statistics 348
Abstract wave equation 256 310
action 393
Addition theorems 305
Adjoint operator 263
Admissible paths 392
Admissible sequence 274
Algebraic approach to quantum statistics 357
Almost all 431
Almost everywhere 431
Annihilation operator 366
Anticommutation relations 367
Antilinear 169
Appolonius' identity 178
Arzela — Ascoli theorem 35
Asymptotically free 368
Balls 16 94
Banach algebra 76
Banach fixed-point theorem 18
Banach space 10
Barycenter 47
Barycentric subdivision 49
Basic equation of quantum statistics 351
Basis 42
Beauty of functional analysis 256
Bernstein polynomials 86
Bessel inequality 202
big bang 357
Bijective 17
Bilinear form 120
Black holes 340
Bolzano — Weierstrass theorem 34
Born approximation 403 404
Bose — Einstein statistics 355
Bosonic Fock space 364
Bosons 363
Bound states 372 394 396
Boundary 31
Boundary point 31
Boundary-eigenvalue problem 245 285 316
Boundary-value problem 125
Bounded 33
Bounded bilinear form 120
Bounded orbits 421
Bounded sequence 9
Brouwer fixed-point theorem 53
Brownian motion 381
C*-algebra 357
Calculus of variations 117 126
Cauchy sequence 10
Cayley transform 423
Characteristic equation 94
Charge density 180
chemical potential 352
Chronological operator 395
Classical function spaces 95 97
Classical Schwarz inequality 12
Closed 15
Closed balls 16
Closed convex hull 31
Closed linear subspace 30
Closure 31
Closure of an operator 414
co S, convex hull of the set S 31
Commutation relations 365
Commutative C*-algebra 358
Compact 90
Compact embedding 96 261
Compact operator 39
Compact set 33
Compactness 33
complete 10
Complete orthonormal system 200 209 210 223 232 247 374
Complete system of generalized eigenfunctions 346
Completeness relation 374
Completeness theorem 222
Complex linear space 4
Complex normed space 7
Complexification 98
Complexification of real Hilbert spaces 178
Composite states of elementary particles 227
Conservation of energy 336 404
Continuity 26
continuous 27
Continuous Dirac calculus 375
Continuous embedding 261
Continuous spectrum 409
| Contraction principle 19
Convergence 9
Convex 29
Convex hull 31
Convexity 29
Convolution 182
Coulomb force 180
Coulomb potential 420
Countable 84
Creation operators 364
Critical point 321
C[a, b], 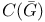 , spaces of continuous functions 14 116 , spaces of continuous functions 14 116
D(A) (or dom A), domain of definition of the operator A 17
Defect indices 423
Deflection of a string 157
Degenerate kernels 251
Degrees of freedom 229
Dense 84
density 84 189 222
Derivative 80
Diagonal sequence 36
diam S, diameter of the set S 47
Diameter 47
Dielectricity constant 420
Diffeomorphism 435
Differential operator 267
Diffusion 379 386
Diffusion equation 379
dim X, dimension of the linear space X 6
DIMENSION 6
Dirac  -distribution 161 -distribution 161
Dirac  -function 158 346 -function 158 346
Dirac calculus 222 373
Dirac function 375
Dirichlet principle 101 123 138
Dirichlet problem 125
Discrete Dirac calculus 374
Discrete spectrum 409
Disk 28
dispersion 328
Dispersion of the energy 352
Dispersion of the particle number 352
Distance 7 47
Distributions 160
Domain of definition 17
Dominated convergence 437
Dual maximum problem 169
Dual space 75
Duality map 169 279
Duality of quadratic variational problems 169
Duality theory 142
Dynamical systems 303
Dynamics 359
Dynamics of quantum systems 328
Dynamics of statistical systems 349
Dynamics of the harmonic oscillator 341
Dyson formula 395
Eigenfunction 247 340
Eigenoscillations 198
Eigenoscillations of the string 317
Eigensolution 230 241
Eigenspace 230
Eigenstate 329
Eigenvalue 83 230
Eigenvalue problem 283
Eigenvector 230
Elastic energy 256
Elasticity 145
electric field 180
Electric field of a charged point 180
Electrostatic potential 180
Electrostatics 183
Embedding theorems 262
Energetic extension 279
Energetic inner product 123 144 273
Energetic norm 144 273
Energetic space 144 154 273
Energy 309
Energy conservation 303 310 313
Energy of the harmonic oscillator 309
entropy 349 353
Equicontinuous 35
Equivalent norms 42 99
Error estimate 19 142
Error estimates via duality 142
Essential spectrum 84 372
Euclidean norm 12 109
Euclidean strategy in quantum physics 379
Euler — Lagrange equation 125
Expansion of our universe 340 357
Exponential function 78
ext S, exterior of the set S 31
Extension 261
Extension Principle 213
Exterior 31
Exterior point 31
Fatou lemma 110 438
Fermi — Dirac statistics 356
Fermionic Fock space 366
fermions 363
Feynman diagrams 400
Feynman formula 393
Feynman path integral 381 385 393
Feynman relation for transition amplitudes 397
Feynman — Kac formula 391
Finite  -net 38 -net 38
Finite elements 151
Finite multiplicity 230
Finite-dimensional Banach spaces 42
Finite-dimensional space 6
Fixed point 19
Fock space 363
Force density 156
Formal hamiltonian 339
Fourier coefficients 195 200
Fourier integral 198
Fourier method 315 316
Fourier series 195 203 241 247
Fourier transform 216 376 378 413
Fourier transform of tempered generalized functions 219
Fredholm alternative 237 245 249 284 287
Fredholm integral operator 95
Free Hamiltonian 371
Friedrichs extension 258 280
Friedrichs extension in complex Hilbert spaces 419
Friedrichs' mollification 186
Fubini's theorem 439
Function 17
Functional calculus 293 331
Functions of bounded variation 440
Functions of self-adjoint operators 293
Fundamental solution 179 182 183 186 413
Fundamental theorem of calculus 119
G(A), graph of the operator A, 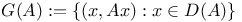 414 414
Gauss method of least squares 197
Gauss theorem 119
Gaussian functions 223
Gelfand — Kostyuchenko theorem 424
Gelfand — Levitan — Marchenko integral equation 410
General position 45
Generalized boundary values 135 138
Generalized derivative 129
Generalized diffusion equation 386
Generalized Dirichlet problem 138
Generalized eigenfunctions 343 372 377
Generalized eigenvectors 424
Generalized Fourier series 195
Generalized functions 156 160
Generalized functions in mathematical physics 179
Generalized initial-value problem 185
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 , inner product on the Sobolev space
, inner product on the Sobolev space 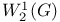 ,
, 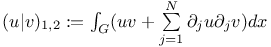
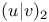 , inner product on the Lebesgue spaces
, inner product on the Lebesgue spaces 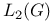 and
and  ,
, 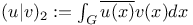
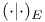 , energetic inner product
, energetic inner product 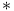 -automorphism
-automorphism  , operator from the set S into the set Y, where
, operator from the set S into the set Y, where 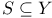
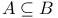 , the operator B is an extension of the operator A
, the operator B is an extension of the operator A 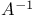 , inverse operator to A
, inverse operator to A 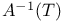 , preimage of the set T,
, preimage of the set T, 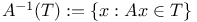
![$C^{k}[a, b]$](/math_tex/cd58d9158fbf4f4d721a3c4b7264057682.gif) ,
, 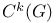 ,
,  ,
,  ,
, 
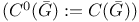 , spaces of smooth functions
, spaces of smooth functions ![$C^{\alpha}[a, b]$](/math_tex/a31066c19ba0d0e7af04e7c5416124d082.gif) ,
, ![$C^{k, \alpha}[a, b]$](/math_tex/2ef3fe6160ab361474d2c3817655983e82.gif) ,
,  ,
, 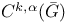
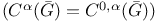 , spaces of Hoelder continuous functions
, spaces of Hoelder continuous functions 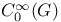 (or
(or 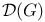 ),
),  , spaces of smooth functions
, spaces of smooth functions 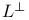 , orthogonal complement to the linear subspace L
, orthogonal complement to the linear subspace L 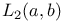 ,
, 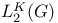
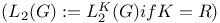 , spaces of integrable functions (Lebesgue spaces)
, spaces of integrable functions (Lebesgue spaces) 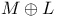 , orthogonal direct sum (
, orthogonal direct sum (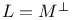 )
)  , derivative of an operator function u = u(t) at time t
, derivative of an operator function u = u(t) at time t 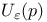 ,
,  -neighborhood of the point p in a normed space,
-neighborhood of the point p in a normed space, 
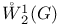 , Sobolev spaces
, Sobolev spaces  , tensor product
, tensor product 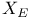 , energetic space
, energetic space  , complexification of the linear space X
, complexification of the linear space X 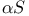 , the product
, the product 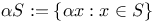 ,
, 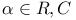
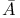 , closure of the linear operator A
, closure of the linear operator A 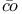 S, closed convex hull of the set S
S, closed convex hull of the set S 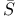 , closure of the set S
, closure of the set S 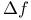 , Laplacian,
, Laplacian, 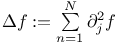
 , the delta distribution
, the delta distribution 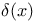 , the Dirac delta function
, the Dirac delta function 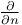 , derivative in the direction of the exterior normal
, derivative in the direction of the exterior normal 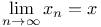 (or
(or 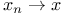 as
as 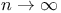 ), the sequence
), the sequence 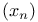 converges to the point x
converges to the point x 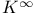 ,
, 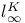 ,
, 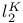
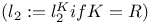 , spaces of sequences
, spaces of sequences  ,
, 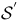 , spaces of distributions
, spaces of distributions 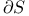 , boundary of the set S
, boundary of the set S  ,
, 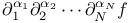 , where
, where 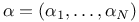 (the classical symbols are also used for the derivatives of generalized functions)
(the classical symbols are also used for the derivatives of generalized functions) 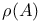 , resolvent set of the linear operator A
, resolvent set of the linear operator A 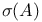 , spectrum of the linear operator A
, spectrum of the linear operator A 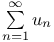 , infinite series in a Banach space
, infinite series in a Banach space 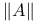 , norm of the linear operator A
, norm of the linear operator A 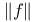 , norm of the functional f
, norm of the functional f 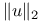 , norm on the Lebesgue spaces
, norm on the Lebesgue spaces 
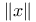 , norm of x
, norm of x 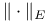 , energetic norm
, energetic norm 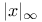 , special norm,
, special norm, 
 , the sum
, the sum 
 (
(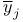 conjugate complex number to
conjugate complex number to 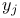 )
)  ), the product of the operators A and B, (AB)(u):=A(Bu)
), the product of the operators A and B, (AB)(u):=A(Bu)  , spaces of continuous functions
, spaces of continuous functions