|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Love R., Morris J., Wesolowsky G. Ч Facilities location |
|
|
 |
| ѕредметный указатель |
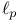 distance, definition 6 11 distance, definition 6 11
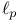 distance, models 23Ч27 86Ч89 113Ч117 distance, models 23Ч27 86Ч89 113Ч117
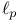 distance, properties 23 distance, properties 23
Absolute deviations 258
Adcock, R.J. 73
Addends 119
Aggregation of demand points 196Ч199 212Ч213
Aikens, C.H. 224
Aly, A.A. 73 169
Ambulance station location 175
Antipode 38 41
Area destinations 43Ч51
Armour, G.C. 254
Balinski, M.L. 95 223
Barber, G.M. 51
Baumol, W.J. 95 223
Bazaraa, M.S. 251 254
Beckenbach, E.F. 110
Belardo, S. 223
Bellman, R. 110
Benders decomposition method 203Ч212 224
Bergman,' L. 223
Bias, directional 257
Bilde, O. 223
Bindschedler, A.E. 31
Bjoerck, A. 31
Bos, H.D. 169
Bounds, bound (lower) for solving the quadratic assignment problem 233Ч236
Bounds, bound (lower) on objective function of location models 16Ч17 34Ч35 89 93 119 122 134 141 205 225
Bounds, bounds (upper) on distances in location models 131Ч132 182
Brady Ч Rosenthal Algorithm 119
Brady, S.D. 113 119 140
Branch-and-bound, solution of dynamic location problem 64Ч66
Branch-and-bound,solution of quadratic assignment problem 231Ч242
Broeckx, F. 254
Buffa, E.S. 254
Burkard, R.E. 254
Burness, R.C. 274
Cabot, A.V. 92 109
Calami, P.H. 93
Callahan, L.G. 229
Center-of-gravity 4Ч5 15 17
Chafe, P.M. 92
Chaiken, J.M. 178
Chained facilities 87
Chalmet, L.G. 169
Chandrasekaran, R. 115 117 139
Charalambous, C. 31 92 122 140
Charnes, A. 92
Chatelon, J.A. 136 140
Chen, R. 169
Christofides, N. 9 274
Church, R.L. 182 183 184 185 223
Circles, covering 118
Circles, great 268Ч271
Circles, market area 163
Circles, unit 257Ч258 265
Concavity of location-allocation problem 158
Conn, A.R. 93
Connors, M. 254
Constraints on distances in minimax location problems 131Ч132
Constraints on locations in rectangular distance location problems 83
Constraints, dual formulation of constrained problems 101Ч102 106Ч107
Continuous existing facilities 43Ч51 162Ч164
Continuous location-allocation 162Ч164
contours 131 269
Converse, A.O. 146
Convex sets, convex hull 15Ч16 88 118 153Ч157
Convex sets, convex polygon 16 115
Convex sets, convex set on sphere 39
Convexity (of functions), 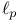 distance function 23Ч24 33 distance function 23Ч24 33
Convexity (of functions), definition 13 32 39
Convexity (of functions), empirical metrics 266Ч268
Convexity (of functions), Euclidean distance function 14 31
Convexity (of functions), hyperbolic approximation 25
Convexity (of functions), rectangular distance function 18
Convexity (of functions), spherical distance 39 73Ч74
Cooper, L. 73 169 273
Cornuejols, G. 223
Cost structures and capacity restrictions 193 195
CRAFT heuristic for floor layout problems 242Ч244 251
Criterion for locating at an existing facility location 14 24 40
Crowston, W.B. 254
Cutting plane procedure 177
Dahlquist, G. 31
Data considerations 187Ч188 202Ч203
Dearing, P.M. 135 140
Decomposition method to solve dual problem 107
Deviations, absolute and squared 258Ч259
Direction vectors, interpretations of dual solutions as 100
Directional bias 257Ч258
Discontinuities in the derivatives of the 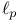 ,distance function 87 ,distance function 87
Distribution centers 143Ч145 200
Dohrn, P.J. 274
Domschke, W. 9
Donnay, J.D.H. 74
Dowling, P.D. 31 93 263
Drexl, A. 9
Drezner Ч Wesolowsky Algorithm 134
Drezner, Z. 31 35 43 69 73 133 134 140 152 169
Duality, minimax dual 129Ч131
Duality, multi-facility 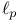 , dual 104Ч107 , dual 104Ч107
Duality, multi-facility Euclidean dual 102Ч104
Duality, single-facility Euclidean dual 95Ч101
Duality, solution methods 107
Duality, structural properties of locationllocation problem 158
DUALOC 189Ч193
Dynamic location 60Ч66
Eddison, R.T. 9
Edge descent method for solving multiacility problems 83Ч86
Efficient point 169
Efroymson, M.A. 223
Eilon, S. 9 274
Elshafei, A.N. 227 251 254
Elzinga Ч Heam Algorithm 118 123
Elzinga, J. 9 31 118 123 140
Empirical studies (of distance functions) 258Ч265
Erlenkotter, D. 73 190 193 223
Euclidean distances, dual models 95Ч104
Euclidean distances, location models 12Ч18 113Ч115 117125
Euclidean metric 257
Eyster, J.W. 31 92
Fire engine travel time 174 256
Fire station location 173Ч178
Fisher, M.L. 223
Floor layout-quadratic assignment problem, branch-and-bound solution method 231Ч242
Floor layout-quadratic assignment problem, Hall Quadratic Placement Algorithm 245Ч251
Floor layout-quadratic assignment problem, heuristic procedures--CRAFT and HC 63Ч66 242Ч244 251
Francis, R.L. 9 31 92 109 135 137 140 169
Gavett, J.W. 254
Gelders, L.F. 169
Geoffrion, A.M. 109 158 162 169 178 187 195 199 206 223 224
Gilmore, P.C. 254
Ginsburgh, V. 255
Goldstein, J.M. 9
Gomory, R.E. 223
Graphics, exclusion property 156
Graphics, inclusion property 153Ч155
Graphics, interactive computer graphics 118 133
Graves, G.W. 199 206 224 254
Great circle metrics 38 268Ч271
Haldane, J.B.S. 73
Hall Quadratic Placement Algorithm 245251
Hall, K.M. 245 254
Hamburger, M.J. 199 223
Handler, G.Y. 169
Hansen, P. 9 169 255 273
Hardy, G.H. 232
Harrald, J. 223
Hausner, J. 174
| Hearn, D.W. 9 31 118 123 136 140
Hendrick, T.E. 174
Heragu, S.S. 254
Hertz, D.B. 9
Heuristics for floor layout, quadratic assignment problems 242Ч244
Heuristics for set-covering problems 177Ч178
Heuristics for site-generating location-allocation problems 157Ч162
Hillier, F.S. 254
Hoelder's inequality 110
Hogan, K. 223
Homogeneity property of a norm 264
Hull, convex hull 15Ч16 118 153 157
Hull, rectangular hull 153Ч154 169Ч170
Hurter, A.P., Jr. 109 169
Hyperbolic approximation 24 31 87
Identity property of a metric 255
Identity property of a norm 264
Ignall, E.J. 178
Infinity, one-infinity norm 264Ч266
Instrument panel layout 229
Intercity road distances 262
Johnson, E.L. 202
Juel Ч Love Algorithm 86
Juel, H. 24 31 92 109 169
Katz, I.N. 31 73
Kaufman, L. 254
Kermack, K.A. 73
Khumawala, B.M. 190 223
Kirca, O. 254
Kleindorfer, G.B. 255
Kochenberger, G.A. 255
Kolen, A.J.W. 138 169
Kolesar Ч Walker Heuristic 177
Kotesar, P. 174 175 177
Kraemer, S.A. 109 140
Krarup, J. 223
Kuehn, A.A. 199 223
Kuenne, R.E. 169 274
Kuhn, H.W. 9 31 109
Kusiak, A. 254
Lagrange multipliers 99 110 121 246 247
Lagrangian function 110 246 247
Land, A.H. 254
Laporte, G. 274
Large region metrics 268Ч271
Larson, R.C. 178
Latitude 38 269
Lawlet, E.L. 254
Lawson Ч Charalambous Algorithm 122125
Lawson, C.L. 122 125 140
layout See Floor layout-quadratic assignment problem
Leamer, E.E. 169
Lee, S. 206
Linear facility location 51Ч60
Linear programming, minimax location, rectangular distances 126Ч131
Linear programming, minimum sum location, rectangular distances 80Ч83
Linear programming, one-infinity norm models 265Ч266
Linear programming, relaxation of distribution model 188Ч189
Linear programming, relaxation of set covering model 177
Littlewood, J.E. 232
Litwhiler, D.W. 73
Location-allocation, site-generating, continuous existing facilities 162Ч164
Location-allocation, site-generating, heuristics for solving 157Ч162
Location-allocation, site-generating, hull properties of 153Ч157 169 170
Location-allocation, site-generating, one-dimensional problem solved by dynamic programming 146Ч150
Location-allocation, site-generating, perturbation solution scheme 158
Location-allocation, site-generating, solved as m-median problem 152Ч156
Location-allocation, site-generating, structural properties 158
Location-allocation, site-generating, two-facility with Euclidean distances 150Ч152
Location-allocation, site-selecting, cost structures and capacity restrictions 193Ч196
Location-allocation, site-selecting, data considerations 187Ч188 202Ч203
Location-allocation, site-selecting, demand point aggregation issues 196Ч199 212Ч213
Location-allocation, site-selecting, DUALOC 189Ч193
Location-allocation, site-selecting, model representation and linear programming relaxation 188Ч189
Location-allocation, site-selecting, set-covering models for site selection 173Ч185
Location-allocation, site-selecting, single-stage, single-commodity distribution system design 186Ч199
Location-allocation, site-selecting, solution by Benders decomposition 203Ч212 224Ч226
Location-allocation, site-selecting, two-stage, multi-commodity distribution system design 199Ч212
longitude 38 269
Love, R.F. 24 31 73 78 92 93 97 109 140 169 254 256 258 263 273
Lowe, T.J. 136 169
m-median problem 155Ч156 188 223
MacKinnon, R.D. 51
Mairs, T.G. 202 256
Manne, A.S. 223
Marucheck, A.S. 169
Mavrides, L.P. 223
Maximal covering location 182Ч183
Maximin problem 114 133Ч135
McGinnis, L.F. 9
McGrew, M.C. 167
Mercator projection 269Ч271
Metrics 255Ч271. See also Travel distances
Miehle, W. 92
Minieka, E. 179 223
Minimax location, contours 131Ч132
Minimax location, convex programming approach 132
Minimax location, dual problem 129Ч131
Minimax location, Euclidean distances 117Ч125
Minimax location, maximin location 133Ч135
Minimax location, multi-facility 120Ч125
Minimax location, rectangular distances 125Ч131
Minimax location, set covering 178Ч181
Minimax location, upper bounds on distances and location constraints 131
Minkowski inequality 34 93
Modeling philosophy 99
Moore, J.M. 31
Morris, J.G. 31 73 92 93 97 140 169 223 256 258 273
Multi-facility location, 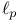 , distance minimum sum model 80Ч86 , distance minimum sum model 80Ч86
Multi-facility location, minimax model 120Ч132
Multi-facility location, properties and solution methods 80Ч90
Multi-facility location, rectangular distance minimum sum model 80Ч86
Nair, K.P.K. 115 117 139
Nemhauser, G.L. 223
Nobert, Y. 274
Nonlinear programming, formulation of minimax location problems 116Ч117 132
Nonlinear programming, solution of dual problem 100 107
Nonlinear programming, solution of multi-facility minimum sum problems 89
Norback, J.P. 73
Norm 264
Norm, one-infinity 264Ч266
Nugent, C.E. 254
O'Kelly, M.E. 169
Odoni, A.R. 178
One-infinity norm 264Ч266
Ostresh, L.M., Jr. 31 92 146 169
Pair exchanging heuristic See CRAFT heuristic for floor layout problems
Parameters, of distance functions 258Ч265
Pardalos, P.M. 169
Pearson, K. 73
Peeters, D. 9 273
Pelletier, P. 274
Perreur, J. 273
Perturbation function 158
Pierce, J.F. 254
Pintelon, L.M. 169
Planchart, A. 109
Plane, D.R. 174
Plyter, N.V. 254
Positivity property of a metric 255
Positivity property of a norm 264
Powers, R.F. 187
Pritsker, A.A.B. 92
Probabilistic destination location 66Ч69
Projection, Mercator 269Ч271
Pruzan, P.M. 223
Quadratic assignment problem See Floor layout-quadratic assignment problem
Quadratic equivalence 245Ч246
Quadratic placement problem 245Ч251
Quasilinearization 96Ч107 109
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте



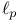 distance, definition
distance, definition