|
|
 |
| Авторизация |
|
|
|
| Поиск по указателям |
|
|
|
|
|
|
|
 |
|
|
|
| Love R., Morris J., Wesolowsky G. — Facilities location |
|
|
|
| Предметный указатель |
Quon, J.E. 92
Rao, M.R. 92
Ray, I'.L. 223
Rectangular destinations 43—51
Rectangular hull 153—157
Rectangular, bound on location models 31 34 93
Rectangular, distance models 18 43—51 80—86 125—132 152—157
Rectangular, metric 257
Rectilinear See Rectangular
Reduction, of branch-and-bound method, of solving floor layout problem 233—235
Restriction, of branch-and-bound method of solving floor layout problem 235—236
Reutzel, E.I'. 255
Revelle, C.S. 182 183 184 185 223
Rhumb line 269
Ritzman, L.P. 254
Road distances 259—262
Road network data 255
Robinson, S.M. 220
Rockafellar, R.T. 109
Roodman, G.M. 73
Rosen, J.B. 169
Rosenthal, R.E. 113 119 140
Rural road distances 259—262
Rural, J. 254
Schrage, L. 223
Schwartz inequality 35
Schwarz, L.B. 73
Scott, A.J. 179 180
Scriabin, M. 254
Separability of rectangular distance problem 18 80
Set-covering models 173—185
Sherali, A.D. 169 254
Shetty, C.M. 169
Shortest arc distance 38 270
Simple plant location problem 189—193 223
Single-facility location, 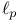 , distance minimum sum model 23—27
Single-facility location, dynamic model 60—66
Single-facility location, Euclidean distance minimum sum model 12—18
Single-facility location, linear facility 51—60
Single-facility location, minimax model 113 117—120
Single-facility location, one-infinity norm model 264—266
Single-facility location, point and area destinations 43—51
Single-facility location, probabilistic destinations 66—69
Single-facility location, properties and solution methods 11—27
Single-facility location, rectangular distance minimum sum model 18—23
Single-stage, single-commodity distribution system design 186—199
Soland, R.M. 169 274
Spfith, H. 139
Sphere, location on a sphere 38—43
Sphere, spherical circle 39
Sphere, spherical disc 39
Sphere, spherical distances 38—39
Spielberg, K. 202 223
Squared distances models, facility location 5 9 21
Squared distances models, quadratic placement 245—251
Stary, M.A. 92
Steinberg, L. 254
Stopping criterion for terminating iterative solution procedures 16—17 88—90
| Straight-line distances See Euclidean distances
Stratmann, K.H. 254
Sum of squares, goodness-of-fit criterion 259
Swain, R. 223
Thisse, J.F. 9 169 273
Toregas, C. 223
Tour-distances 271
Trade-off curves 179—181 193
Travel distances (mathematical models of), applications of 255—256
Travel distances (mathematical models of), empirical distance functions 256—258
Travel distances (mathematical models of), empirical metrics, convexity, optimal location 266—268
Travel distances (mathematical models of), empirical studies 258—265
Travel distances (mathematical models of), large region metrics 268—271
Travel distances (mathematical models of), modeling vehicle tour-distances 271
Travel distances (mathematical models of), weighted one-infinity norm 264—266
Tree, for branch-and-bound method, of solving dynamic location problem 64—66
Tree, for branch-and-bound method, of solving floor layout problem 238
Triangle inequality 34 255 264 266 275
Truscott, W.G. 73
Two-stage, multi-commodity distribution, system design 199—212
Unit circles 257—258 265
Urban road distances 259—262
Urquhart, M. 227
Van Roy, T.J. 73 223
Van Wassenhove, L.N. 169
Variations on the single-facility model, dynamic location 60—66
Variations on the single-facility model, linear facility 51—60
Variations on the single-facility model, point and area destinations 43—51
Variations on the single-facility model, probabilistic destinations 66—69
Variations on the single-facility model, spherical location 38—43
Varignon frame 8 27—28
Verdini, W.A. 31 92
Vergin, R.C. 254
Vijay, J. 140
Vollmann, T.E. 254
Wakefield, G.W. 202
Walker, W.E. 174 175 177 178
Wallace, W.A. 223
Ward, J.A. 169 223 264 273
Watson-Gandy, C.D.T. 9 274
Weber, A. 8
Weiszfeld iterative solution method 1415 25—26 40—41
Weiszfeld, E. 8 10 31 40 92 120 271
Wendell, R.E. 41 43 169 264 273
Werson, S.J. 92
Wesolowsky, G.O. 31 42 43 73 92 93 133 134 140
Westwood, J.B. 256
Whinston, A.B. 254
White, J.A. 9 31 92 273
Wierwille, W.W. 31 92
Wimmert, R.J. 254
Witzgall, C. 109 273
Wolfe, P. 95 223
Wong, J.Y. 254
Wyman, S.D. 229
Yeong, W.Y. 31 93
Yerex, L. 78
Young, D. 140
|
|
|
| Реклама |
|
|
|
|
|
О проекте