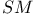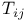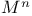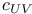Авторизация
Поиск по указателям
Frankel T. — The geometry of physics: an introduction
Обсудите книгу на научном форуме Нашли опечатку?
Название: The geometry of physics: an introductionАвтор: Frankel T. Аннотация: This book is intended to provide a working knowledge of those parts of exterior differential forms, differential geometry, algebraic and differential topology, Lie groups, vector bundles and Chern forms that are essential for a deeper understanding of both classical and modern physics and engineering. Included are discussions of analytical and fluid dynamics, electromagnetism, thermodynamics, the deformation tensors of elasticity, soap films, special and general relativity, the Dirac operator and spinors, and gauge fields, including Yang-Mills, the Aharonov-Bohm effect, Berry phase, and instanton winding numbers. Before discussing abstract notions of differential geometry, geometric intuition is developed through a rather extensive introduction to the study of surfaces in ordinary space; consequently, the book should also be of interest to mathematics students. This book will be useful to graduate and advanced undergraduate students of physics, engineering and mathematics. It can be used as a course text or for self study.
Язык: Рубрика: Физика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2001Количество страниц: 688Добавлена в каталог: 15.12.2013Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Space-time notation 141 Spacelike 193 Spatial metric 298 Spatial slice 316 Special 392 Special, linear group, Sl(n) 11 392 Special, orthogonal group SO(n) 392 Special, unitary group SU(n) 392 Sphere lifting theorem 605 Spin structure 515—518 Spinor, "representation" of SO(3) 497 Spinor, "representation" of the Lorentz group 509 Spinor, 2-component 497 Spinor, 2-component, left- and right-handed 513 Spinor, adjoint 532 Spinor, bundle 517 Spinor, connection 518—521 Spinor, cospinor 513 Spinor, Dirac or 4-component 513 Spinor, group Spin(3) 497 Stability 324 Stability, 2-component, subgroup 457 Stiefel manifold 459 616 Stiefel vector field 426 Stokes's theorem 111—114 Stokes's theorem for pseudoforms 117 Stokes's theorem, generalized 155 Stored energy of deformation 623—626 Stress forms, Cauchy 618 Stress forms, Cauchy, symmetry 620 Stress forms, first Piola — Kirchhoff 622 Stress forms, second Piola — Kirchhoff 623 Stress tensor 295 617 Stress-energy-momentum tensor 295 Structure constants 402 Structure constants in a bi-invariant metric 566 Structure group of a bundle 433 452 Structure group of a bundle, reduction 433 SU(N) 392 493—497 Subalgebra 411 Subgroup 411 Subgroup, isotropy = little = stability 457 Submanifold 26 Submanifold of 29 Submanifold of 4 8 Submanifold with transverse orientation 115 Submanifold, 1- and 2-sided 84 Submanifold, embedded 27 Submanifold, framed 115 Submanifold, immersed 169 Submersion 181 Summation convention 59 Support 107 Symmetries 527—531 Symplectic form 146 Symplectic manifold 146 Synge's formula 325 Synge's theorem 329 Tangent bundle 48 Tangent bundle, unit 51 Tangent space 7 25 Tangent vector 23 Tellegen's theorem 646 Tensor, analysis 298—303 Tensor, Cauchy — Green 82 Tensor, contravariant 59 Tensor, covariant 58 Tensor, deformation 82 625 Tensor, metric 58 Tensor, mixed 60 Tensor, mixed, linear transformation 61 Tensor, product 59 66 Tensor, product, representation 482 Tensor, rate of deformation 632 Tensor, transformation law 62 Tensor, two-point 622 Theorema egregium 231 Thermodynamics, first law 180 Thermodynamics, second law according to Caratheodory 181 Thermodynamics, second law according to Lord Kelvin 181 Thom's theorem 349 Timelike 193 Topological invariants 346 Topological quantization 468 Topological space 12 Topological space, compact 13 topology 12 Topology, induced or subspace 12 Torsion of a connection 245 Torsion of a connection, 2-form 249 Torsion of a space curve 196 Torus 16 Torus, maximal 393 Transformation group 456 Transition matrix 24 254 414 Transition matrix 417 Transition matrix 417 Transition matrix 417 Transition matrix 417 Transitive 456 Translation (left and right) 393 Transversal to a submanifold 34 Transverse orientation 115 Triangulation 346 Tunneling 558 Twisted product 415 Unitary group U(n) 392 Universe, static 292 Universe, stationary 291 Vacuum state 557 558 Vacuum state, tunneling 558 Variation of a map 153 Variation of action 154 Variation of Ricci tensor 306 Variation, external 523 Variation, first, of arc length 232 Variation, first, of area 221 322 Variation, internal 523 Variation, second, of arc length 324—332 Variational derivative 307 526 Variational equation 128 Variational principles of mechanics 275—281 Variational vector 128 153 272 Vector as differential operator 25 Vector, analysis 92 136—138 Vector, bundle 413—419 Vector, bundle-valued form 488 Vector, contravariant or tangent 23 Vector, coordinate 25 Vector, covariant = covector = 1-form 41 Vector, field 25 Vector, field, along a submanifold 269 Vector, field, flow (1-parameter group) generated by 32 33 Vector, field, integral curve of 31
Реклама
 |
|
О проекте
|
|
О проекте