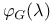|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Cvetkovic D., Doob M., Sachs H. — Spectra of graphs. Theory and application |
|
|
 |
| Предметный указатель |
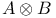 67 67
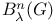 27 27
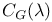 27 27
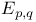 132 132
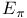 236/237 236/237
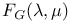 28 28
 65 65
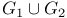 51 51 
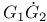 52 52
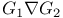 54 54
 44 44
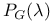 12 12
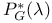 34 34
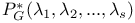 29 29
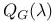 25 25
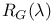 26 26
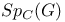 27 27
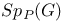 23 23
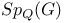 26 26
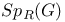 26 26
 27 27
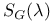 27 27
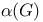 88 88
 -product 66 -product 66
 15 15
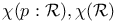 146 146
 232 232
 -product 66 -product 66
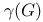 119 119
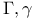 134 134
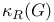 260 260
 -operator 252 257 -operator 252 257
 -product 66 -product 66
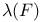 260 260
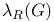 260 260
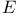 -eigenvalue 123 -eigenvalue 123
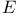 -polynomial 123 -polynomial 123
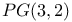 165 165
 -sum of graphs 66 76 -sum of graphs 66 76
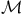 -combination 215 216 -combination 215 216
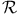 126; 141 126; 141
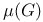 260 260
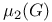 263 263
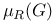 260 260
 -product 54 57 98 99 211 -product 54 57 98 99 211
 -electron 229 231 -electron 229 231
 -factor 111 -factor 111
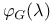 49 49
(0, 1, —l)-ineidenee matrix 16 265
(T', T'')-cover 130 133
(—1, 1, 0)-adjacency matrix 26 183 184 195 196 201
8-eage 266
a(G) 265
A-variation with repetitions 212
A.SCHBACHEE, M. 185
Adam, A. 223 224
Adjacency matrix 11*
Adjacent vertices 11*
Admittance, matrix of 27 37—41
Aigner, M. 180 183
Alphabet 131
Amis, A. T. 112
Anderson Jr., W. N. 27 266
Annulene 231
ARC 11*
Ash, R. B. 36
Association scheme 178 193 194 197 198 264
Aufbau — Prinzip 237
Automorphism group 119 121 134—155 161 194 231 269 270
Automorphism group, transitive 136 — 138 153 154
Automorphism of a graph 118*
Babai, L- 134 144 145 153—155
Baghavarao, D. 178
Baker, G- 256
Basis of a NEPS 66*
Bece, A. 36
Beineke, L. W. 170 269
benzene 244
Bhagawandas 103
BIBD 50 165/166 167 173 176 177 185 187 190
BIBD, symmetric see “Design symmetric”
Biggs, N. L. 7 134 138 194 198
Bingel, G. 97
Binomial coefficient graph of characteristic n 179 180
Biphenyl 244
Blackwell, D. 216
Block design, balanced incomplete 50 165 166 190 197
Block diagonal sum of a matrix 141
Block of a digraph 264
Boiling point 270
Boolean function of graphs 65 67 68—72 203—207
Bose, R. C 103 178 179 183 193 198
Bottema, 0. 259
Bridge 15 242
Brooks theorem 90 102
Brooks, R. L. 38
Brown, W. G. 190
Burnside, W. 148
Butadiene 244
Bvssemaker, F. C 185 197 266 268 269
Cameron, P. J. 177 178
Canonical form of a matrix 141 145
Cardinal product of graphs 66
Cartesian product of graphs 66
Catalan’s constant 247
Cauchy’s inequality 19
Cayley formula 217
Cayley graph 138 153 154 155
Cayley — Hamilton theorem 20 81
Chang, L. C 174
Chao, C.-Y. 144
CHARACTER 146
Chebyshev’s polynomial 73 78 218—220
Chelnokov, V. M, 224
Chen, W.K. 36
Chess board 214 245 247 251
Circuit 15*
Circuit, Hamiltonian 113
Circulant matrix 53 153
Clark, F. H. 36 60
Clatworthy, W. H. 195
Clebsch graph 184 185
Cliques, pair of bridged 170 171 186
Coalescence 158 159
Coates, C. L. 36 47
Cocktail-party graph 72 178 178 197 217
Code, error-correcting 75
Code, error-correcting, perfect 131 190
Coding theory 131
Codivisor 125 126 128 150
cof X 264
Coixatz, L. 13 36 78 84 128 156 160 232 256
Coloration, D-feasible 116 121 123 129
Complement of a graph 16*
Complete product 54 57 58
Complex, linear 118
Complexity of a graph 38 49 50 72 225
Component 15*
Component, strong 55
Composition of graphs 71
Configuration, coherent 194
Conjunction 66
Connection from i to j 47
Connectivity, algebraic 265
Connectivity, algebraic, edge-; vertex- 265 269
Connor, W. S. 195
Cook, C. R. 183
Coreljic, S. 268 269
Coulson — Bushbrooke pairing theorem 232
Coulson, C. A. 36 87 232
| Coulson’s bond-order matrix 238
Courant-Weyl inequalities 51 93
Cover, unbranched 118
CP(k) 177
Cube (graph) 225 266 269
Cube, rc-dimensional 178 179 186
Cutpoint 15* Cut set
Cvetkovic, D. M. 8 36 44 46 50 56 57 62—64 68 69 71 77 88 92 101 102 113 131 132 140 175 180 182 186 187 204 206—209 212 213 224 234—236 242—244 258 259 266 269
CYCLE 15*
d(F) 260
d-girth 81
Degree 14*
Degree matrix 16*
Delsahte, P. 183
Dembowski, P. 178
Density matrix 238
Desargues’ graph 266
Design, symmetric 166 167 176 185 187 190 227
Desoer, C. A. 36
Dewar, M. S. J. 241 259
diam (F) 260
Diameter 15 88 162—165 178 179 186 187 192—194 198 269
Digraph 11*
Digraph with weighted adjacencies 35 41 47
Dimer 245—251
Direct sum 64 57 158 162 163 167 186 195 211 219 262
Direct sum of matrices 141*
Dismantling of a graph 234
Distance 15*
Distance, matrix 268 264
Distance, polynomial 263 264 271
Divisor (front, rear) 116—133 149—152 190 198 257
Divisor (front, rear), generalized 118
Divisor (front, rear), proper 117
Djokovic, D. 2. 224
dodecahedron 225 269
Donath, W. E. 114
Doob, M. 8 69 78 107 162 163 165 169 175 176 185—187 223 260 261
Double-m-property 132
Dynamic mean of the valencies 46 217
EDGE 11*
Edge, directed 11*
Edge, multiple 11*
Eigenvalue, simple 18 135—140 162 166 204 270
Eigenvalue, simple, of a graph 12*
Eigenvector of a graph 12*
Elspas, B. 153 167 224
Erdos, P. 191
ethylene 244
Eulers theorem 243
Extended p-sum of graphs 66
Face 242
Factor, (= 1-factor) 239—246 249 251 258 259
Factor, generalized 111
Factor, linear 15 34 35 37 129
Factor, quadratic 129
Factor, regular of degree a 15 107 224 239
Feit, W. 193
Feobenius, F. G. 18
Fibonacci numbers 249 250
Fiedler, M. 27 39 265 266
Figure (elementary, basic) 32 87 88 95 96 240 243 244 259
Finck, H.-J. 57 94 98 102 103 113 133 163
Fisher, M. E. 254—256
Forest 16 37 90 258
Friendship theorem 193
Generating function for the number of walks 44 213 251
Geometry, projective 165
Gewirtz, A. 193
Gibbs, R. A. 187
girth 15 81 87 97 190—193 197 266 269
Godsil, C. D. 144 155
Goethals, J. M. 8 115 177 178 183
Graham, R. L. 264
Gramian matrix 199 — 202
Graovac, A. 241
Graph 11*
Graph,  -prime 98 99 100 207 223 -prime 98 99 100 207 223
Graph, bicomplete see “Graph complete
Graph, bipartite 15*
Graph, complete 13 16 72 90 113 162—164 185 196 206 207 211 217 221 223 262 264 269
Graph, complete bipartite 16 50 72 133 158 162—165 174 195 197 206 207 211
Graph, complete multipartite (k-partite, k-complete) 16 73 93 114 133 163 164 170 211 223
Graph, connected 15*
Graph, cyclically k-partite 82 83
Graph, directed 11*
Graph, disconnected 15*
Graph, distance-transitive 183 193 194 197 198
Graph, Eulerian 201
Graph, integral 266
Graph, k-colourable 15
Graph, k-degenerate 90
Graph, linear directed 15*
Graph, metrically regular 178 179 183 186 194 264
Graph, partial 14*
Graph, planar 242 245 256 266
Graph, plane 242 243 256
Graph, prime 182
Graph, properly coloured 15
Graph, regular 14*
Graph, schlicht 11*
Graph, self-complementary 97 98 114 269 270
Graph, semiregular 16 31 42 49 62 133 173—175 186 193
Graph, strong 196 203
Graph, strongly connected 15*
Graph, strongly connected, regular 103 115 140 163 174 179 185 190 193—197 203 226 227
Graph, symmetric 138 193—197 203 226 227
Graph, tetrahedral 180 183
Graph, tetrahedral, totally disconnected 16 99 113 162 196
Graph, tetrahedral, triangular 170 174 184
Graph, tetrahedral, weakly symmetric 138
Graphs, cospectral 24 125 134 153 155 156—161 163 166 170—179 183—187 197 266 267
Graphs, cospectral, isomorphic 12*
Graphs, cospectral, isospectral see “Graphs cospectral”
Grohmann, G. 57 98
Group, dihedral 155
Group, dihedral of automorphisms see “Automorphism group”
Group, dihedral of quaternions 153
Group, dihedral of symmetries of a molecule 146
Group, dihedral, orthogonal 143 144
Group, dihedral, unitary 144 145
Gunthard, Hs. H. 146
Gutman, I. 60 231 234—236 238 241 258 259 267
H(n) 170
Hadamard matrix 176 197
Haemebs, W. 19 115
Hakimi, S. L. 112
Hall, K. M. 115
Hamiltonian (operator) 228
Hamming distance 131
Harary, F. 11 36 44 46 112 187 266
Haynsworth, E. V. 20
Heawood’s graph 225
Heilbronner, E. 36 59 128 146 259
Hestbnes, M. D. 197
Heydemann, M. C. 187
Higman, D. G. 193—195 200
Hoffman — Singleton graph 165 197
Hoffman, A. J. 7 8 47 78 79 83 90—93 95 112—115 157 158 160 165 168 170 171 174—176 186 188 260—264
Holt, D. 138
Hosoya, H. 36 264
Howes, L. 92 93 113 262
Huckel matrix 231
Huckel theory 228—238 270
Huckel, E. 13 228 230 231
HUTSCHENREUTHER, H. 38 39
Hydrocarbon, acyclic 244
Hydrocarbon, acyclic, alternant 231 232
Hydrocarbon, acyclic, benzenoid 239 244
Hydrocarbon, acyclic, cata-fuzed 244
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



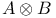
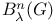
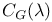
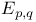
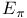
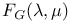


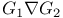

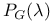
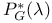
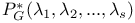
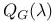
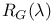
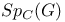
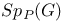
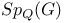
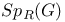

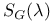
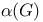
 -product
-product 
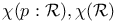

 -product
-product 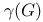
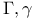
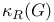
 -operator
-operator  -product
-product 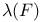
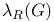
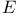 -eigenvalue
-eigenvalue 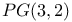
 -sum of graphs
-sum of graphs 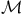 -combination
-combination 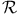
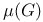
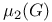
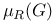
 -product
-product  -electron
-electron