|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Stewart G.W., Sun J. — Matrix perturbation theory |
|
|
 |
| Предметный указатель |
Idempotent matrix 28
Inertia of a matrix 196
Inner product 53 62
Invariant subspace 21 22
Invariant subspace, approximate (see Invariant subspace residual
Invariant subspace, backward perturbation 175 178—180
Invariant subspace, backward perturbation, optimal 176
Invariant subspace, characterization 220 227
Invariant subspace, complementary 222 225
Invariant subspace, complex conjugate, eigenvalues 29
Invariant subspace, definition 22
Invariant subspace, left 221 225
Invariant subspace, normalization 239 244
Invariant subspace, perturbation theory 229 236 240 244 254
Invariant subspace, perturbation theory,  theorem 253 258 259 theorem 253 258 259
Invariant subspace, perturbation theory, canonical angles 237
Invariant subspace, perturbation theory, first 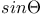 theorem 250 258 theorem 250 258
Invariant subspace, perturbation theory, Hermitian matrix 244
Invariant subspace, perturbation theory, second 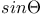 theorem 251 255 258 theorem 251 255 258
Invariant subspace, reduced form of matrix 221
Invariant subspace, reduction to block diagonal form 224
Invariant subspace, representation of matrix 22 220 231 235 237
Invariant subspace, representation of matrix, condition number 240
Invariant subspace, residual bound 174 206 229—236 246 249 254
Invariant subspace, residual bound, canonical angles 232
Invariant subspace, residual bound, nonorthonorinal basis 251
Invariant subspace, sensitivity 21 90 219
Invariant subspace, simple 220 221 226 227 231 235 238
Invariant subspace, simple, exist ence of complementary invariant subspace 225
Invariant subspace, spectral projection 114 225 240
Invariant subspace, spectral projection, canonical angles 226
Invariant subspace, spectral resolution 223 228 237 244
Invariant subspace, Sylvester’s equation (q.v.) 222
Inverse matrix, artificial ill-conditioning 122 124
Inverse matrix, asymptotic forms and derivatives 130—132
Inverse matrix, condition 17
Inverse matrix, condition number 119 127 133
Inverse matrix, condition number inverse matrix 102
Inverse matrix, condition number, 2—norm 121
Inverse matrix, condition number, optimal 133 135 193
Inverse matrix, condition number, relation to determinant 134
Inverse matrix, condition number, relation to eigenvalues 122 134 135
Inverse matrix, condition number, significant digits in inverse 120
Inverse matrix, distance from singularity 120 121 133
Inverse matrix, left and right inverses 134
Inverse matrix, perturbation theory 117—124
Inverse matrix, perturbation theory, linear system 124
Inverse matrix, perturbation theory, random perturbations 131
Inverse matrix, well-conditioned 120
Irreducible matrix 186 188
Jacobi, C. G. J. 209
Jacobian matrix 134
Jiang, E. 178 180
Jordan block 20 28 174 180 186 300
Jordan block, function of 73
Jordan block, powers of 28
Jordan canonical form 20 21 26 174 227 280
Jordan canonical form, associated invariant subspaces 21
Jordan canonical form, computation 27
Jordan canonical form, Drazin generalized inverse 113
Jordan canonical form, limitations 21 227
Jordan canonical form, principal vector 21
Jordan Wielandt matrix 32 34 35 259
Jordan, C. 26 34 35 45 289
Jordan, P. 63
Kalian, W. 27 45 46 133 151 178 180 209 211 217 218 244 258 259
Kato, T. 4 176 178 244
Konig, D. 88
Korganoff, A. 152
Krasnoselski, M. A. 98
Krein, M. G. 98
Kronecker, L. 289
Kroticcker product 30 228 258
Lancaster, P. 227
Laplace, P. S. 11
Lawson, C. L. 152 163
Least squares 10 11 101
Least squares, asymptotic forms and derivatives 162
Least squares, backward perturbation 160—163
Least squares, backward perturbation, Bjoerck’s theorem 162
Least squares, condition, condition number 156 163
Least squares, condition, reflected by solution 156 158 163
Least squares, condition, square of  158 163 158 163
Least squares, constrained 109 112
Least squares, cross-product matrix backward perturbation 161
Least squares, elliptic norm 109
Least squares, errors in the variables 163
Least squares, errors in the variables, bias 267
Least squares, expanded equations 107 161 163
Least squares, Gauss — Markov theorem 110
Least squares, Gauss. C. F. 108
Least squares, measurement error models 163
Least squares, normal equations 107
Least squares, numerical methods 152 163
Least squares, perturbation theory 156 160
Least squares, perturbation theory, Bjoerck’s theorem 158
Least squares, perturbation theory, errors in a column 163
Least squares, perturbation theory, perturbation in A 157
Least squares, perturbation theory, perturbation in b 156
Least squares, perturbation theory, perturbation of the residual vector 160
Least squares, perturbation theory, structured perturbation 158 163
Least squares, priority dispute between Gauss and Legendre 109
Least squares, reduced form 155
Least squares, regression diagnostics 163
Least squares, residual vector 107
Least squares, solution by pseudo-inverse 107
Least squares, statisticians’ notation 109
Legendre, A. M. 109
Levy, L. 186
Lidskii, V. D. 209 210
Linear functional 56
Linear system 101 114
Linear system, artificial ill-conditioning 128
Linear system, asymptotic forms and derivatives 130—132
Linear system, backward perturbation 128—130 133 135 136
Linear system, Bauer — Skeel 128 133
Linear system, Bauer — Skeel theorem 127
Linear system, component-wise bounds 125 126
Linear system, condition number 125 127
Linear system, Oettli Prager theorem 130
Linear system, perturbation theory 124 128 132
Linear system, perturbation theory from inverse matrix 124
Linear system, perturbation theory, perturbation in matrix 124
Linear system, perturbation theory, perturbation in the right-hand side 126 127
Linear system, perturbation theory, structured perturbation 127 128
Linear system, reflected by solution 126
Linear system, residual bound 128 130
Linear system, Rigal Cinches theorem 128
Linear system, structured 129
Linear system, structured perturbation 133
LINPACK 133
Littlewood, J. E. 88
Loewy, A. 27
LR algorithm 11
Lu, Q.-k 99
Mac Duffee, C. C. 4
Majorization 81 88 89
Marcus, M. 4
Matching distance (see Under eigenvalue) 167
Matrix function 20
Matrix function, exponential 73
Matrix function, Jordan block 73
Matrix function, Neumann series 73
Matrix function, power series 73
Matrix function, rational 29
Matrix function, spectral resolution 229
Matrix norm (see under norm) 64
Matrix pair (definite) 281—283 289
Matrix pair (definite), 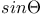 theorems 323 324 theorems 323 324
Matrix pair (definite), bounds for dif 319
| Matrix pair (definite), condition number 313 316 324
Matrix pair (definite), definition 282
Matrix pair (definite), diagonalizability 283
Matrix pair (definite), eigenangle 314
Matrix pair (definite), eigenangle, perturbation theory 324
Matrix pair (definite), eigenangle, variational characterization 314
Matrix pair (definite), eigenangles 324
Matrix pair (definite), eigenvector 318
Matrix pair (definite), failure of definition in real case 290
Matrix pair (definite), Fischer’s theorem 281
Matrix pair (definite), limitations of the theory 316
Matrix pair (definite), matching distance 324
Matrix pair (definite), perturbation of eigenspaces 317 322
Matrix pair (definite), perturbation of eigenvalues 314 317
Matrix pair (definite), perturbation of eigenvectors 322
Matrix pair (definite), positive definite B 281 282 289
Matrix pair (definite), projective metric 285—287 290 314
Matrix pair (definite), right and left eigenspaces 317
Matrix pair (definite), spectral resolution 318
Matrix pair (definite), spectral resolution normalized 318
Matrix pair (regular) 273 274
Matrix pair (regular), approximate eigenspace 306—309
Matrix pair (regular), condition number 294 300 303
Matrix pair (regular), continuity of eigenvalues 291
Matrix pair (regular), deflating subspace 311
Matrix pair (regular), diagonalizable pair 300 303
Matrix pair (regular), eigenspace 312
Matrix pair (regular), eigenspace, backward perturbation 309
Matrix pair (regular), eigenspace, characterizations 304
Matrix pair (regular), eigenspace, complementary 306
Matrix pair (regular), eigenspace, definition 303
Matrix pair (regular), eigenspace, eigenvectors 312
Matrix pair (regular), eigenspace, simple 277
Matrix pair (regular), first order approximation 292
Matrix pair (regular), first order error bounds 293
Matrix pair (regular), generalized Bauer Fike theorem 301
Matrix pair (regular), generalized Bauer-Fike, theorem 294 301 311
Matrix pair (regular), generalized Henrici Theorem 311
Matrix pair (regular), generalized Sehur form 276 289
Matrix pair (regular), generalized Sehur form, real pair 290
Matrix pair (regular), generalized, Hoffman Wielandt theorem 311
Matrix pair (regular), Gerschgorin theory 294 300
Matrix pair (regular), Gerschgorin theory, diagonal similarity 297
Matrix pair (regular), Gerschgorin theory, generalized Gerschgorin theorem 295
Matrix pair (regular), Gerschgorin theory, simplification of bounds 296
Matrix pair (regular), infinite eigenvalues 274
Matrix pair (regular), loft projective metric 288 290
Matrix pair (regular), normal pair 311
Matrix pair (regular), number of eigenvalues 275
Matrix pair (regular), perturbation of eigenspaces 309—311
Matrix pair (regular), perturbation of eigenvalues 311
Matrix pair, characteristic, equation 274
Matrix pair, eigenvalue 273
Matrix pair, eigenvalue problem 274
Matrix pair, eigenvalue, 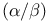 notation 273 notation 273
Matrix pair, eigenvector 273
Matrix pair, equivalence 276
Matrix pair, generalized Schur form 277
Matrix pair, Hermitian 281
Matrix pair, infinite eigenvalues 271 273
Matrix pair, limitations 288
Matrix pair, linear transformations of matrix pairs 275
Matrix pair, metrics 284 288
Matrix pair, nonsingular B 274
Matrix pair, numerical methods 274
Matrix pair, scaling problems 272
Matrix pair, singular 273 274 289
Matrix pair, systems of differential equations 289
Matrix pencil 209 271
Matrix pencil, rectangular 272
Matrix pencil, singular 271
Mcintosh, A. 194 227 228
Metric 53
Metric from a norm 62
Metric, pseudo-metric 62
Metrics for subspaces 50 90
Metrics for subspaces,  -metric 96 98 99 -metric 96 98 99
Metrics for subspaces, basis metric 95 98 99
Metrics for subspaces, completeness 99
Metrics for subspaces, distance from a vector to a subspace 90 99
Metrics for subspaces, failure to generate the gap topology 94
Metrics for subspaces, gap function 90 94 98 99
Metrics for subspaces, gap function, canonical angles 92
Metrics for subspaces, gap function, definition 91
Metrics for subspaces, gap function, equivalence of gap functions 91
Metrics for subspaces, gap function, equivalence of gap, projections 93
Metrics for subspaces, gap topology 91 93 94
Metrics for subspaces, projection metrics 93 94
Metrics for subspaces, Schaffer’s metric 99
Metrics for subspaces, unitarily invariant, metrics 94—98
Metrics for subspaces, unitary invariance 99
Meyer, C. 244
Milman, D. P. 98
Mine, H. 4
Minkowski, H. 59 61
Minkowski’s inequality 61
Mirsky, L. 71 72 194 209 210
Mirsky’s theorem 194 204 206 208 209
Moler, C. B. 290
Moore, E. H. 108 109
Moore-Penrose generalized inverse (see pseudo-inverse) 102
More, G. 135
Multiple eigenvalue 297 300
Multiple eigenvalue, perturbation of eigenvectors 312
Multiple eigenvalue, recovery of bounds for ordinary eigenvalue problem 294 297
Multiple eigenvalue, right projective metric 288 290
Multiple eigenvalue, spectral resolution 300 310
Multiple eigenvalue, spectral variation 302
Multiple eigenvalue, Weierstrass canonical form 280 290
Nashed, M. Z. 108
Nearly singular matrix 120
Neumann series 73
Nilpotent matrix 29
Nondefective matrix 21 28
Norm and spectral radius 73
Norm of a linear functional 56
Norm, 2-norm (q.v.) 3
Norm, absolute norm 52 72 76
Norm, combining norms 61
Norm, consistency 65 67 71
Norm, consistency, definition 66
Norm, consistency, failure 65
Norm, consistency, family of consistent norms 69
Norm, consistency, unitarily invariant norms 80
Norm, consistency, vector norm consistent with a matrix norm 66
Norm, convexity and norms 59 63
Norm, dual 57 59 67
Norm, dual of dual 58
Norm, elementary properties 51
Norm, elliptic 53 62 111
Norm, equivalence of norms 54 55 59 65 72
Norm, failure 64
Norm, Frobenius norm (q.v.) 65
Norm, generated by an inner product 62
Norm, generated by linear transformation 53
Norm, generated by positive definite matrix 53 62
Norm, Hilbert — Schmidt norm 210
Norm, Hoelder norms 51 57 60 61 64 69 121 134
Norm, infinite dimensional spaces 71
Norm, limits and norms 55 65
Norm, matrix  -norm 69 71 72 -norm 69 71 72
Norm, matrix 1—norm 69 71 72
Norm, matrix norm 49 64
Norm, operator norm 67 68 72
Norm, operator norm, consistency 67 68
Norm, polar 59
Norm, spectral radius and norms 67 72 73
Norm, subordinate norm (see norm operator
Norm, unitarily invariant (q.v.) 50
Norm, vector  -norm 51 55 69 -norm 51 55 69
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 theorem
theorem 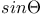 theorem
theorem 
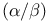 notation
notation  -metric
-metric  -norm
-norm