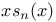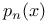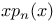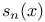|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Roman S. — The Umbral Calculus |
|
|
 |
| Предметный указатель |
Abel function 11 72 85
Abel operator 14
Abel polynomials 72—75
Abel polynomials, binomial identity 73
Abel polynomials, expansion theorem 74
Abel polynomials, generating function 72
Abel polynomials, inverse under umbral composition 75
Abel polynomials, transfer formula 72
Actuarial polynomials 123—125 140
Actuarial polynomials, generating function 123
Actuarial polynomials, recurrence relation 124
Actuarial polynomials, Sheffer identity 123
Actuarial polynomials, umbral composition 125
Adjoint, of linear operator on P 34
Appell cross sequence 140
Appell identity 27 see
Appell sequence 17 26—28 164
Appell sequence, Appell identity 27
Appell sequence, conjugate representation 27
Appell sequence, expansion of 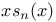 27 27
Appell sequence, expansion theorem 26
Appell sequence, generating function 27
Appell sequence, multiplication theorem 27
Appell sequence, polynomial expansion theorem 26
Associated sequence(s) 17 25—26 48 50—51 164
Associated sequence(s), action of operator h(t) on 26
Associated sequence(s), binomial identity 26
Associated sequence(s), conjugate representation 25
Associated sequence(s), expansion of 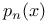 26 26
Associated sequence(s), expansion of 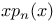 26 26
Associated sequence(s), generating function 25
Associated sequence(s), operator characterization of 25
Associated sequence(s), polynomial expansion theorem 25
Associated sequence(s), recurrence formulas 48
Associated sequence(s), relationship between 51
Associated sequence(s), transfer formulas 50
Automorphisms of P* 33—36 38
Backward difference functional 63
Bell polynomials 82—86
Bell polynomials, connection with Abel function 85
Bell polynomials, connection with exponential polynomials 85
Bell polynomials, connection with idempotent numbers 85
Bell polynomials, connection with Laguerre polynomials 86
Bell polynomials, connection with Lah numbers 86
Bell polynomials, connection with Stirling numbers 85
Bernoulli numbers 12 94 98—100
Bernoulli numbers of second kind 114
Bernoulli numbers, connection with harmonic series 99—100
Bernoulli numbers, connection with Stirling numbers 99
Bernoulli polynomials 12 93—100 105 117—118 129 135 140 151
Bernoulli polynomials of second kind 113—119
Bernoulli polynomials of second kind, connection with Bernoulli polynomials 117—118
Bernoulli polynomials of second kind, expansion theorem 116
Bernoulli polynomials of second kind, generating function 116
Bernoulli polynomials of second kind, Gregory's formula 117
Bernoulli polynomials of second kind, polynomial expansion theorem 117
Bernoulli polynomials of second kind, Sheffer identity 116
Bernoulli polynomials of second kind, symmetry of 119
Bernoulli polynomials, Appell identity 94
Bernoulli polynomials, complementary argument theorem 100
Bernoulli polynomials, connection with Bernoulli polynomials of second kind 117 118
Bernoulli polynomials, connection with Euler polynomials 105 135
Bernoulli polynomials, connection with lower factorial polynomials 93
Bernoulli polynomials, connection with Stirling polynomials 129
Bernoulli polynomials, Euler —Maclautin expansion 97
Bernoulli polynomials, expansion theorem 96
Bernoulli polynomials, generating function 94
Bernoulli polynomials, multinomial theorem 97
Bernoulli polynomials, polynomial expansion theorem 93
Bernoulli polynomials, recurrence formula 95
Bernoulli polynomials, transfer formula 96 100
Bessel polynomials 78—82
Bessel polynomials, binomial identity 79
Bessel polynomials, generating function 79
Bessel polynomials, polynomial expansion theorem 80
Bessel polynomials, recurrence formula 80
Bessel polynomials, transfer formula 78—79
Binomial convolution 160—161
Binomial identity 26 see
Binomial type, sequence of 26
Boole polynomials 127
Boole's summalion formula 104
Central difference series 68 see
Chebyshev polynomials 162 166 173
Complementary argument theorem for Bernoulli polynomials 100
Complementary argument theorem for Euler polynomials 106
Conjugate representation see also specific polynomial sequence
Conjugate representation of Appell sequences 27
Conjugate representation of associated sequences 25
Conjugate representation of Sheffer sequences 20
Connection constants problem 131—138
Cross sequences 140
Delta functional 10
Delta operator 13
Delta series 4
Delta series, genetic 82
Derivations on P* 34
Derivations on P*, chain rule for 46
Dobinski's formula 66
Duplication formulas 132 137—138
Duplication formulas for Hermite polynomials 137
Duplication formulas for Laguerre polynomials 137
Duplication formulas for Mittag — Leffler polynomials 138
Euler numbers 102
Euler polynomials 12 100—106 135—136 161
Euler polynomials, Appell identity 102
Euler polynomials, Boole's summation formula 104
Euler polynomials, complementary argument theorem 106
Euler polynomials, connection with Bernoulli polynomials 105 135—136
Euler polynomials, expansion theorem 104
Euler polynomials, generating function 102
Euler polynomials, multiplication theorem 105
Euler polynomials, Newlon’s expansion of 101
Euler polynomials, recurrence formula 103
Evaluation functional 7 11 163
Expansion theorem for Appell sequences 26
Expansion theorem for associated sequences 25
Expansion theorem for Sheffer sequences 18 164
Exponential polynomials 63—67 85
Exponential polynomials, binomial identity 64
Exponential polynomials, connection with actuarial polynomials 123
Exponential polynomials, connection with Laguerre polynomials 134
Exponential polynomials, connection with Poisson — Charlief polynomials 122
Exponential polynomials, Dobinski's formula 66
Exponential polynomials, expansion theorem 65
Exponential polynomials, generating function 64
Exponential polynomials, recurrence formula 64
f 3 7 12
Falling factorial polynomials see Lower factorial polynomials
Fibonacci numbers 149
Formal power series 3—4
Forward difference functional 11 56
Forward difference operator 14
Gaussian coeffcient see q-Umbral calculus
Gegenbauer polynomials 162 166 173
Generalized Appell type 19 164
Generating function see also specific polynomial sequence
Generating function for Appell sequences 27
Generating function for associated sequences 25
Generating function for Sheffer sequences 18—19
Generic associated sequence 82—84 see
Generic associated sequence, binomial identity 83
Generic associated sequence, conjugate representation 82
Generic associated sequence, generating function 83
Generic associated sequence, recurrence formula 83—84
Gould polynomials 67—72 149
Gould polynomials, binomial identity 69
| Gould polynomials, expansion theorem 70
Gould polynomials, generating function 68
Gould polynomials, recurrence formula 69
Gould polynomials, Stirling's formula 70
Gould polynomials, Vandermonde's convolution formula 69
Gregory's formula 59 117
Heine's theorem see q-Umbral calculus
Hermite polynomials 87—93 135 137 140 150 158
Hermite polynomials, Appell identity 88
Hermite polynomials, classical Rodrigues formula 89
Hermite polynomials, duplication formula 137
Hermite polynomials, generating function 88
Hermite polynomials, multiplication theorem 93
Hermite polynomials, polynomial expansion theorem 89—90
Hermite polynomials, q-Hermite polynomials see q-Umbral calculus
Hermite polynomials, recurrence formula 89
Hermite polynomials, recurrence relation for 158
Hermite polynomials, squared Hermite polynomials 128
Hermite polynomials, urnbrai composition of 92
Hermite polynomials, Weierstrass operator 88
Idempotent numbers 85
Integration by parts 118
Inverse relations 147—156
Invertible functional 10
Invertible operator 13
Krawtchouk polynomials 126
Lagrange inversion formula 138—140
Laguerre polynomials 11 108—113 120 133—134 137 140 151 158—159
Laguerre polynomials, classical Rodrigues formula 109
Laguerre polynomials, connection with exponential polynomials 134
Laguerre polynomials, connection with Poisson — Charlier polynomials 120
Laguerre polynomials, duplication formula 137
Laguerre polynomials, generating function 110
Laguerre polynomials, Lab numbers 109
Laguerre polynomials, polynomial expansion theorem 112
Laguerre polynomials, recurrence formula 110
Laguerre polynomials, recurrence relation for 158—159
Laguerre polynomials, second order differential equation for 110—111
Laguerre polynomials, Sheffer identity 110
Laguerre polynomials, transfer formula 109
Laguerre polynomials, umbral composition of 112—113
Lah numbers 86 109
Linear operator, continuous 33
Lower factorial polynomials 56—63
Lower factorial polynomials, binomial identity 57
Lower factorial polynomials, conjugate representation 61—62
Lower factorial polynomials, expansion theorem 58
Lower factorial polynomials, generating function 57
Lower factorial polynomials, Gregory's formula 59
Lower factorial polynomials, Newton's forward difference interpolation formula 58
Lower factorial polynomials, polynomial expansion theorem 59
Lower factorial polynomials, recurrence formula 56 58
Lower factorial polynomials, Vandermonde convolution formula 57
Mahler polynomials 129—130
Meixner polynomials of first kind 125—126
Meixner polynomials of second kind 126
Mittag — Leffler polynomials 75—77 138
Mittag — Leffler polynomials, binomial identity 76
Mittag — Leffler polynomials, duplication formula 138
Mittag — Leffler polynomials, expansion theorem 77
Mittag — Leffler polynomials, generating function 76
Mittag — Leffler polynomials, recurrence formula 77
Mott polynomials 130
Multiplication theorem 27 93 97—98 105
Multiplication theorem for Bernoulli polynomials 97—98
Multiplication theorem for Euler polynomials 105
Multiplication theorem for Hermite polynomials 93
Narumi polynomials 127
Newton's forward difference polynomials 58
Operational formulas 144—147
Orthogonal polynomials, three term recurrence relation for 156 159—160
P 6
Peters polynomials 128
Pidduck polynomials 126—127
Pincherele derivative 49
Poisson dislribution 119
Poisson — Chadier polynomials 119—123 136 140
Poisson — Chadier polynomials, connection with Laguerre polynomials 120
Poisson — Chadier polynomials, generating function 120
Poisson — Chadier polynomials, recurrence formula 121
Poisson — Chadier polynomials, Sheffer identity 121
Poisson — Chadier polynomials, umbral composition of 122
Polynomial expansion theorem see also specific polynomial sequence
Polynomial expansion theorem for Appell sequences 26
Polynomial expansion theorem for associated sequences 25
Polynomial expansion theorem for Sheffer sequences 18 164
Poweroid 19
q-binomial coefficient see q-Umbral calculus
q-derivative see q-Umbral calculus
q-Hermite polynomials see q-Umbral calculus
q-Leibniz formula see q-Umbral calculus
q-Umbral calculus 174- 182
q-Umbral calculus, Gaussian coefficients 175
q-Umbral calculus, Heine's theorem 179
q-Umbral calculus, q-binomial coefficienls 175—177
q-Umbral calculus, q-derivative 175
q-Umbral calculus, q-Hermite polynomials 180—181
q-Umbral calculus, q-Hermite polynomials, generating function 180
q-Umbral calculus, q-Hermite polynomials, inverse under urnbrai composition 180
q-Umbral calculus, q-Hermite polynomials, polynomial expansion theorem 18 i
q-Umbral calculus, q-Leibniz formula 176
Recurrence formulas for associated sequences 48
Recurrence formulas for Sheffef sequences 50 166
Rising factorial polynomials 63
Sheffef identily 21 see
Sheffef sequence 17—24 164—165
Sheffef sequence, action of operator of form h(t) on 21
Sheffef sequence, characterization by Sheffer identity 21 165
Sheffef sequence, conjugate representation of 19—20 164
Sheffef sequence, expansion of 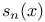 24 24
Sheffef sequence, expansion of 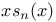 24 24
Sheffef sequence, expansion theorem 18 164
Sheffef sequence, generating function 18—19 164
Sheffef sequence, operalor characterization of 20 165
Sheffef sequence, performance with respect to multiplication in urnbrai algebra 23
Sheffef sequence, polynomial expansion theory 18 164
Sheffef sequence, recurrence formulas 50
Sheffef shift 49- 50
Sheffer operators 42—44
Sheffer operators, adjoint of 42
Sheffer operators, group of 43
Signless Stirling numbers of first kind 63
Squared Hermite polynomials 128
Steffensen sequences 140—143
Stirling numbers of first kind 57—59 61—62 66—67 85 99 115 129 134
Stirling numbers of first kind, connection with Stirling polynomials 129
Stirling numbers of first kind, signless 63
Stirling numbers of second kind 59—60 62—64 66—67 85 99 129 144
Stirling numbers of second kind, connection with Stirling polynomials 129
Stirling polynomials 128—129
Stirling polynomials, connection with Bernoulli polynomials 129
Stirling polynomials, connection with Stirling numbers 129
Stirling polynomials, generating function 128
Stirling's formula 70
Transfer formulas 50 166
Transfer operator 165
Translation operator 14
Umbral algebra 7
Umbral composition 41
Umbral operator 37—42 see
Umbral operator, adjoint of 38
Umbral operator, group of 40
Umbral shift(s) 45—49 165
Umbral shift(s), adjoint of 46
Umbral shift(s), formula for 48
Umbral shift(s), relationship between 47
Vandermonde convolution formula 57 69
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте