|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Bertlmann R.A. Ч Anomalies in Quantum Field Theory |
|
|
 |
| ѕредметный указатель |
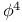 theory 142Ч149 theory 142Ч149
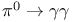 decay 233Ч238 decay 233Ч238
't Hooft Ч Veltman integral formula 207 222
't Hooft Ч Veltman regularization 207Ч210 220Ч223
Action principle 492
Action, active coordinate transformations 470 472Ч474
Action, adjoint derivative 51Ч53 294
Adjoint map 94
Adjoint operator 412
Adjoint representation 94 161
Adler Ч bardeen theorem 205
Aharonov Ч Bohm effect 306Ч311
Aharonov Ч Bohm effect, fibre bundle description 309
Aharonov Ч Bohm effect, phase shift 308
Aharonov Ч Bohm effect, quantum mechanics 307
Anomalous divergence 188 214 219 253
Anomaly 176 188 193Ч195 212Ч214 296Ч297 338 353 354 365 366 378 410 426
Anomaly and phase 194Ч195 440
Anomaly cancellation 245Ч247
Anomaly for L-currents 190
Anomaly formula 341
Anomaly shift 517
Anomaly, 2-dimensional 214Ч227 260Ч261 276 283 286
Anomaly, ABJ 188 197Ч214
Anomaly, Bardeen 241 243 297 351 436 446
Anomaly, consistent 243 272 401
Anomaly, covariant 243 266 270 271 297 393Ч394 397Ч400 405
Anomaly, dispersion relations 216Ч220
Anomaly, IR behaviour 230Ч231
Anomaly, non-Abelian 241Ч244 265Ч272 297 338 379 382 435Ч436 445Ч446
Anomaly, nonlocal extensions 406Ч407
Anomaly, normalization 369
Anomaly, regularization independence 261Ч263
Anomaly, singlet 238Ч241 259 296 379 381 400 411
Anomaly, UV behaviour 231Ч232
Anti-BRS transformation 175
Antiderivation rule 43 333
Associated bundle 103
Atiyah Ч Singer index theorem 305 319 410Ч411 426Ч427 443 534 541
Atlas 31
Axial current 178
Axial current, non-Abelian 183
Axial gauge 169
Background field 371
Bardeen Ч Zumino counterterm 515
Bardeen Ч Zumino polynomial 392Ч393 396Ч400 404Ч405 519 541
Base space 95
beta function 388
Betti numbers 69Ч71
Bianchi identity 165 295 354 463 480
Bianchi identity, first 482
Boundary 11
Boundary operator 62
Bounded operator 412
BRS algebra 376 520Ч524
BRS algebra for nontrivial fibre bundles 528Ч529
BRS algebra, shifted 524Ч525
BRS operator 172Ч175 345 350 356 379 523
BRS operator, shifted 524
BRS operator, total 521
BRS transformation 172Ч175 345Ч346 357 358 366 521
Cartan structure equation 114Ч116 463 479
Cartan's exterior algebra 42
Cartan's homotopy formula 334
Cauchy's integral formula 217
Chain complex 62
Chain terms 352 354 369 537
Chain terms, solutions 382Ч383 386 389
Chains 61
Charts 31
Chern character 425
Chern number 305 319 327
Chern Ч Simons form 296 314 327Ч328 335Ч337 354 367 372 377 379 444 516 525 527
Chern Ч Simons form, gauge transformation 336Ч337
Chern Ч Simons form, variation 339 341
Chern Ч Simons term 240
Chern Ч Simons theory 379
Chiral transformations 181 250Ч251 256 267
Chiral transformations, Jacobian 280Ч286
Chirality split 268
Christoffel connection 460Ч461
Christoffel connection, transformation 469 471 474
Christoffel symbol 460
Closed set 10
Closure 10
Coboundary 67 364 378
Coboundary operator 363
Cochain 363
Cocycle 67 362 364 378
Cocycle condition 361 364
Coderivative see "Adjoint derivative"
Cohomology 363Ч365 378Ч379
Cokernel 413
Commutation relations functional 391
Compactification 13 17
Compactness 12
Compatibility condition 112 117 304 317 442 443
Conformal group 456
Conformal transformation 456
Conformally flat manifolds 456Ч458
Connectedness 18
Connection 105Ч113 304 309 317
Connection 1-form 109Ч112 442 460
Connection, global 443
Conservation laws 177Ч185 228
Consistency conditions for gravitational anomalies 512Ч515 522 529
Consistent current 392
Consistent current, gauge transformation 392 396 402Ч403
Continuous map 13
Contractible space 329
Contravariant vector 459
Coordinate transformations 458Ч460 468Ч474
Coordinate transformations, infinitesimal 470Ч471
Coordinate transformations, matrix 469
Cosmological constant 493
Cotangent bundle 104
Cotangent space 39Ч40
Cotangent space, basis 39 373
Covariant anomaly condition 394
Covariant current 392Ч393 397 404
Covariant current, gauge transformation 393 404
Covariant derivative 113 162 163 181 343 376 461 467Ч468 479 496
Covariant vector 459
Covering 12
Current 1-form 296 395
Current 1-form on Sp  401 401
Current operator 210Ч214
Curvature 113Ч117 304 310 463Ч465 479 484
Curvature 2-form 114 443
Curvature, transformation 469 471 473
Cutkosky rule 225
CYCLE 63
d'Alembert operator 420
De Rham cohomology 67Ч73
de Rham cohomology, group 67
de Rham cohomology, inner product 70
de Rham complex 68
de Rham's theorem 69
Derivative in Sp  391 391
Descent equations 341 354 369 372 376 377 385 387 525Ч527 530
Determinant bundle 448Ч449
Determinant fermion 439
Determinant of a differential operator 137Ч139
Determinant of the Dirac operator 277Ч286
Determinant of the metric 453
Determinant of the Weyl operator 428
Determinant, gauge invariance 429Ч430 439
Determinant, phase 439Ч440
Determinant, regularized 277 280Ч286
Diffeomorphism group 469
| Diffeomorphisms 13 532
Differential forms 40Ч58 84
Differential forms on Sp  348 348
Differential forms, anticommutator 56Ч58
Differential forms, closed 54
Differential forms, coclosed 54
Differential forms, coexact 54
Differential forms, commutator 56Ч58
Differential forms, exact 54
Differential forms, harmonic 54 71Ч72
Differential forms, inner product 51
Differential forms, integration of 45Ч46
Differential forms, trace 56
Differential map 73 89
Dilatation vector 475
Dirac equation 154 178 229
Dirac equation, energy spectrum 229
Dirac field, free 154 155
Dirac field, interacting 155Ч156
Dirac genus 534
Dirac monopole 297Ч306 380 426
Dirac monopole, fibre bundle description 303
Dirac monopole, field strength 302
Dirac monopole, gauge potentials 299 301 302
Dirac monopole, gauge transformation 300Ч302
Dirac monopole, magnetic charge quantization 301
Dirac monopole, winding number 305
Dirac operator 249 255 265 497
Dirac operator, construction on 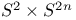 441Ч443 441Ч443
Dirac operator, eigenvalue equation 409
Dirac operator, spectrum 431
Dirac operator, zero modes 409
Dirac sea 227Ч233
Dispersion relations 216Ч220
Dual algebra *Lie G 358
Dual current form 296 395
Dual p-form 49
Duality 68
Einstein anomaly 509Ч510 533 536
Einstein anomaly, 2-dimensional 537
Einstein equation 492Ч493
Einstein equation, with matter 493
Einstein equation, without matter 492
Einstein ghost 521
Einstein invariance 504Ч505 519
Einstein tensor 493
Einstein transformations 474 504 518Ч519
Einstein transformations, generator 511
Einstein Ч Hilbert action 491
Electrodynamics 287
Electrodynamics, action 292
Electrodynamics, current conservation 292
Electrodynamics, field strength 178
Electrodynamics, field strength tensor 288
Electrodynamics, gauge potential 156Ч159 290
Electrodynamics, Lagrangian 177
Elliptic differential operators 417Ч420 422
Embedding 454
Energy-momentum tensor 493Ч495 508 519
Energy-momentum tensor, antisymmetric 509
Energy-momentum tensor, covariant 519
Energy-momentum tensor, covariant conservation 493 505
Energy-momentum tensor, symmetric 493 503 504
Energy-momentum tensor, traceless 507
Equivalence principle 458
Euclidean space 250
Euclidean space, 2-dimensional 261
Euler characteristic 69Ч71
Exponential map 90
Extended gauge group element 374
Exterior covariant derivative 113
Exterior derivative 42 373 443
Exterior derivative in Sp  348 360 348 360
Exterior derivative in Sp 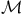 518 518
Exterior derivative on  374 374
Face 59
Faddeev Ч Popov determinant 167
Faddeev Ч Popov ghosts 92 168Ч170 344 350 356 379
Faddeev Ч Popov operator, differential 345
Faddeev Ч Popov operator, integrated 345
Family index theorem 436
Fermionic action 497
Fermionic Lagrangian 500Ч502
Feynman gauge 159
Feynman parameter integral 206 221
Feynman propagator for fermions 154Ч155
Feynman propagator of the photon 158Ч159
Feynman propagator, scalar 137
Feynman's path integral 124Ч129
Feynman's path integral, formula 126
Feynman's path integral, Hamilton formalism 126Ч129
Fibre bundles 95Ч117 348
Fibre bundles, reconstruction 99Ч101
Fibres 95 304 316
Field strength 116Ч117
Flow 78Ч81 90 93 105
Flow, infinitesimal 79
Frame bundle 104
Fredholm alternative 415
Fredholm operators 413Ч414 420
Fredholm operators, direct sum 415
Fredholm operators, product 416
Fujikawa's method 255Ч259
Fujikawa's method, regularization 257Ч259 263 268 270 432Ч435
Fujikawa's uncertainty principle 263Ч265
Function of an operator 274
Functional derivative 132Ч135 347
Fundamental vector field 105 360
Fundamental vector field, commutator 401
Gamma function 278
Gauge condition 166
Gauge fixing 158 167 169
Gauge group 159Ч161
Gauge group space 347 374 447
Gauge operator 343Ч344 353 356 360
Gauge operator, commutation relations 343 353
Gauge operator, integrated 344
Gauge potential 110Ч113 162
Gauge transformations 112 161 270 374
Gauge transformations, axial 179 181
Gauge transformations, infinitesimal 164
Gauge transformations, left-handed 180 182
Gauge transformations, right-handed 180 182
Gauge transformations, vector 178 181
Gauge variation 344
Gauss theorem 467
Gaussian cut-off 257 434
Gaussian integration 137Ч139 259 435
Gaussian integration for Grassmann variables 151Ч154
Gell-Mann matrices 160
Generating functional 140 142 144 154 155 169 191Ч197 251 342
Graded algebra 173
Grassmann algebra 42 149Ч154
Grassmann differentiation 150
Grassmann integration 150 256 264 433
Grassmann measure 256 267
Gravitational anomaly formula 527 531
Gravitational anomaly, axial 542Ч543
Gravitational anomaly, covariant 518Ч520 540
Gravitational anomaly, normalization 536
Gravitational anomaly, total 522
Green functions 185Ч190
Green functions for interacting fields 144Ч147
Green functions, connected 147Ч149
Green functions, free 139Ч141
Gribov ambiguity 171Ч172
Group parameter space 372
harmonic oscillator 121
Heat equation 274Ч275
Heat kernel 274Ч276 423Ч425
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте



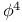 theory
theory 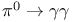 decay
decay