Jensen C.U., Lenzing H. — Model Theoretic Algebra with particular emphasis on Fields, Rings, Modules :: Электронная библиотека попечительского совета мехмата МГУ

Главная Ex Libris Книги Журналы Статьи Серии Каталог Wanted Загрузка ХудЛит Справка Поиск по индексам Поиск Форум

Авторизация

Поиск по указателям

Jensen C.U., Lenzing H. — Model Theoretic Algebra with particular emphasis on Fields, Rings, Modules

Обсудите книгу на научном форуме

Нашли опечатку?
Выделите ее мышкой и нажмите Ctrl+Enter

Название: Model Theoretic Algebra with particular emphasis on Fields, Rings, Modules

Авторы: Jensen C.U., Lenzing H.

Аннотация:

It is the purpose of these notes to present some subjects from ring theory, field theory and module theory from a model theoretic point of view, basically, by making a semantic (first order) analysis of the corresponding algebraic concepts. Many non-trivial questions hereby arise, which may be of independent interest.

Язык:

Рубрика: Математика/

Серия: Сделано в холле

Статус предметного указателя: Готов указатель с номерами страниц

ed2k: ed2k stats

Год издания: 1989

Количество страниц: 447

Добавлена в каталог: 30.10.2011

Операции: Положить на полку | Скопировать ссылку для форума | Скопировать ID

Предметный указатель

Module, $\Sigma$ -pure-injective      161
Module, 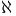${\aleph}$ -compact      144 146 155 141
Module, 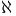${\aleph}$ -generated      136
Module, 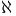${\aleph}$ -injective      141
Module, 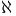${\aleph}$ -presented      136
Module, 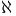${\aleph}$ -projective      152
Module, 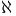${\aleph}$ -pure-injective      141
Module, ${\aleph}_{0}$ -compact      157
Module, (m,n)-presented      228
Module, absolutely pure      377
Module, algebraically compact      125 128 130—131 144 154 157 165 171 126 375
Module, coherent      237
Module, compact      158
Module, cyclically presented      97
Module, finitely generated      227
Module, finitely generated free      227
Module, finitely presented      93—94 96—98 107—108 112 228 234 368
Module, flat      96—97 106 109 115—119 122—123 229—232 234—235 244 368
Module, FP-injective      98—99 108 119 153 239 245 577
Module, free      367
Module, free of infinite rank      232—233 235—236
Module, graded      300
Module, group-graded      300
Module, injective      119—123 368
Module, Kronecker      207—208
Module, linearly compact      289
Module, local      238 241
Module, locally coherent      237
Module, locally finite      244
Module, of finite n -presentation      264
Module, of length t      226
Module, projective      122 227 231 234—235 367
Module, projective dimension of      234—235
Module, Pruefer      208
Module, pure-injective      125 128 126 375
Module, pure-projective      234—235 374
Module, semiprimary      281
Module, semisimple      244
Module, simple      226—227 237 244
Module, torsion-free      109 116—117 119
Module, uniform      184 238 241
Module, weak dimension of      231 233
Monk, L. G.      91 100
Morphism, of affine schemes      314
Morphism, of functors      380
Morphism, of varieties      310
Nagata, J.      272 284
Nakayama's lemma      323
Nazarova, L.      340
Nazarova-Roiter's theorem      340
Neukirch, J.      77
Neumann, B. H.      100
Nilpotent      404
Nilradical      405
Nishimura, J.      254
Noether-Ostrowski irreducibility theorem      6
Noetherian module      405
Noetherian ring      98 113 115—118 120—123 237—240 245 370 405
Noetherian scheme      314
Non-Archimedean field      41 48 64
Non-essential map      272
Norm residue symbol      29
Northcott, D. G.      117
Number field      28 31 62 66 69
Number field, integers in      119
Oberst, U.      176
Okoh, F.      194
Olsson, J. B.      85
Open subscheme      314 345
Openness, of finite global dimension      347
Openness, of finite projective dimension      345
Openness, of self-injective dimension      346
Orbit space      317
Order      318—319
Ordered field      8—9 20
Ordered field, rank of      44—47
Ore condition      248
Ore domain      248
Osofsky, B. L.      282 301
Path algebra      405
Path category      405
Peano arithmetic      63
Peano field      61—63 65
Peano field, rigid      63
Peano ring      61—65 68 74 219 261 288 406
Peano ring, non-Noetherian      65
Peano ring, rigid      63
Perfect ring      124 244 406
Pfister, A.      26
PI-degree      273—274
Pierce, R. S.      261
Place extension theorem      305 309 330 344 347
Polynomial algebra      192
Polynomial identity ring      273
Polynomial, absolutely irreducible      6
Ponomarev, V. A.      169 207
Popescu, N.      198 379
Positive diagram      308
Positive primitive formula      92 96 99—100 114
Positive quantifier-free formula      307
Positive-primitive definable subgroup      92
Pourchet, Y.      28
Power series algebra over afield      265
Power series field      30—31
Power series field $K(({X}_{1}))(({X}_{2}))...(({X}_{n}))$       50
Power series field $K(({X}_{1},...,{X}_{n}))$       31 50
Power series fields      23
Power series fields, in infinitely many indeterminates      59
Power series fields, in infinitely many variables      60
Preinjective Kronecker module      208
Preprojective Kronecker module      194
Prest, M.      93 158 163 219
Prestel, A.      306
Prime ideal      4O6
Prime ring      248 40S
Primitive ring      268 406
Principal ideal domain      115 250 4O6
Principal open sets      311
Principal open subscheme      314
Procesi, C.      273
Projective cover      406
Projective cover of module      251
Projective dimension      123—124 234—235 344—345
Projective dimension of module      234—235
Projective dimension of ultraproduct      234
Projective dimension, axiomatizability of finite      235 369
Projective envelope      187
Projective module      122 227 231 234—235 367
Projective object in abelian category      381
Projective resolution      368
Proper polynomial identity      273
Pruefer domain      72 108—109 111 249—250 279 407
Pruefer group      238—239
Pruefer module      205 208 245
Pseu do-convergence in valued field      288
Puiseux field      23 69
Puiseux's theorem      23 62
Pure submodule      96 105 109 123 164 240 242 244 256 374
Pure-cact sequence      96 98 155 234 291 300 374
Pure-global dimension      155 190 194 291—293 300 375
Pure-global dimension, of free algebra      301
Pure-global dimension, of free Boolean algebra      291
Pure-global dimension, of Kronecker algebra      299
Pure-global dimension, of polynomial algebra      301
Pure-injective dimension      155 375
Pure-injective envelope      128
Pure-injective module      125 128 126 375
Pure-injective resolution      375
Pure-projective dimension      291—292 374
Pure-projective module      234—235 374

Pure-projective resolution      374
Pure-semisimple ring      164—165 168 170 181—182 218 222 292 407
Pythagoras number      24—28 70—71
Pythagorean field      34—35 47
Quantifier elimination for modules      99
Quasi-algebraically closed field      19
quiver      407
Quiver, representation of      407
Quotient category      198
Rank of ordered field      44—47
Rank, of a flat module      244
Rank, of lattice      318
Rank, of order      318
Rational function field      47
Raynaud, M.      50
Real closed field      9 21—23 26—28 30—31 41 43 45—49 58—59
Real closed fields non-isomorphic      22
Real closure      9
Reduced product, algebraically compact      192
Reduced product, of 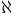$\aleph$ -injective modules      152
Reduced ring      310 407
Reduction modulo the radical      187 189 216
Regular local ring      50—51 262 265 278 288 293 378
Regular map      310
Regular representation of algebra      316
Regular, action of algebraic group      312
Regular, Kronecker module      208
Reiner, I.      324
Relatively algebraically closed field      4
Rentschler, R.      278 353
Representable functor      313 386
Representation, of quiver      407
Representation-finite ring      292 329 331—332 338 165 408
Representative functor      380
Resolution, flat      372
Resolution, injective      368
Resolution, projective      368
Resolution, pure-injective      375
Resolution, pure-projective      374
Ribenboim, P.      19 21 24—26 28 30 63
Riedtmann, C.      341
Rigid algebraic structure      63
Rigid field      63
Ring, 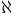$\aleph$ -coherent      142 152
Ring, $\Sigma$ -algebraically compact      281
Ring, algebraically compact      281 285 287—288 323
Ring, Artinian      226 232 234—237 241 245
Ring, Bezout      249 279
Ring, coherent      97 99 106 108 122 124 229 231—232 236—237 253 255j 258—259 264—265 278—279 377^
Ring, commutative Noetherian      115—118 120—122
Ring, complete local      56
Ring, countable      157 235—236 239
Ring, Dedekind      98 109 115 168 170 182 190 201 216 218 232 250—251 265 291
Ring, F-semiperfect      127 247—249 252
Ring, henselian      50—58 60
Ring, hereditary Noetherian      232
Ring, hereditary ring      214 254
Ring, Jacobson semi-simple      247
Ring, left perfect      251
Ring, local      111 118 378
Ring, Noetherian      98 113 115 123 237—240 245 370
Ring, of continuous functions      271
Ring, of finite width      232—233 245
Ring, Peano      61 288
Ring, perfect      124 244
Ring, prime      248
Ring, primitive      268
Ring, Pruefer      249
Ring, pure-semisimple      164—165 170 181—182 218 222 292
Ring, reduced      310
Ring, regular local      50—51 262 265 278 288 293 378
Ring, representation-finite      292 331 165
Ring, self-injective      195 197 285
Ring, semihereditary      253
Ring, semilocal      123
Ring, semiperfect      249 252
Ring, semiprimary      213 236 239 252
Ring, semiprime      273
Ring, uniformly coherent      72 229—232 236—237 254—256 259
Ring, valuation      249 320
Ring, von Neumann regular      127 160 169 181 183 195 232 239 247—248 254 261 265 268 270 282 285 291
Ring, with Ore condition      248
Ring, with polynomial identity      273
Ring, with sufficiently many a.c.i.modules      182
Ringel, C. M.      208—209 219 336 340
Robinson, J.      61
Robinson, R.      35 66—67 70
Robson, J. C.      198 278 353
Roiter, A. V.      166 340—341
Roquette, P.      75
Rosenberg, A.      169 263
Roth's theorem      49
Rothmaler, P.      163
Rowen, L. H.      273
Rucker, R. V. B.      172
S-closed field      87
S-group      82
S-radical of group      83
S-semisimple group      82—83
Sabbagh's theorem      158 180
Sabbagh, G.      105—106 109 122—123 158 220 259
Sai, Y.      165
Sally, J. D.      254
Salmeron, L.      341
Samuel, P.      51
Saturated structure      12
Scheme, affine      313
Scheme, Noetherian      314
Schiilting, H. W.      250
Schilling, O. F. G.      47 288 290
Schneider, T.      49
Schofield's theorem      313
Schofield, A. H.      263 313 345 347
Self-injective dimension      172 346
Self-injective ring      195 197 285
Semi continuous function      313
Semi hereditary ring      253 408
Semicontinuity, of fibre dimension      313
Semicontinuity, of orbit dimension      313
Semifir      257 408
Semilocal ring      123
Semiperfect ring      249 252 408
Semiprimary ring      213 236 239 252 260 281
Semiprime ring      273
Semisimple Artinian ring      408
Semisimple module      244 4O8
Semisimple ring      408
Separable closure      18
Separable, Mac Lane      17
Separably closed field      17
Sequence, almost-split      332
Sequence, exact      402
Sequence, pure-exact      155 234 374
Serre subcategory      197
Serre, J. -P.      29
Shannon, R. T.      244
Sharpe, D. W.      121 159 283 398
Shelah, S.      12 329
Simple artinian ring      408
Simple group      78
Simple module      226—227 237 244 408
Simple ring      408
Simson, D.      152 155 168 183 356
Slomson, A. B.      1 5 12 16 20 159
Smal0, S.      340
Small category      384
Small submodule      409
Small, L.      263
Snider, R. L.      268
Socle      244

1 2 3 4

О проекте