|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Petersen K.E. — Ergodic theory |
|
|
 |
| Предметный указатель |
Strong topology (on group of m 71
Sub - Markov operator 120
Subadditive sequence 240
Submartingale 91 103
Submartingale Convergence Theorem 104 106
Subshift of finite type 153 162 273
Subskeleton 286
Supermartingale 103 105 106
Supremum (of a family of factors) 185
Swanson, L. 221 224
Symbolic cascades 153
sz 185
Szemer di - Furstenberg Theorem 150 (see also Ergodic Szemer di - Furstenberg Theorem 150 (see also Ergodic Szemer di Theorem) di Theorem)
Szemer di Theorem 159 163 di Theorem 159 163
Szemer di, E. 162 di, E. 162
Tchebychef, P. L. 158
Thomas, R. K. 61 279
Three-series theorem 94
Time mean 42 44
Toeplitz sequence 209
Topological dynamics 133 150
Topological entropy 264 265
Topological entropy, Bowen definition of 266 267
Topological entropy, of a symbolic cascade 266
Topological ergodicity 151 152
Topological strong mixing 151
Topological weak mixing 151
Topological weak mixing without strong mixing 210 212 214
Total function 189 191 202 208
Totoki, Haruo 9
Tower 197 200 Kakutani
Transition matrix 36 51 52 59
Transitivity, metric 42
Transitivity, regional 152
Translation of the torus (ergodicity of) 51
Translation on a compact group 153 (see also Group rotation rotation)
Trigonometric polynomial 148
Turan, Paul 162
Ulam. S. M. 72 99
Uniform distribution mod 1 156
Uniform function 189 190 202 208 210 329
Uniform mixing 64
Uniformly almost periodic 136 137 154
| Uniquely ergodic 42 138 152 186 187 217
Unitary operator determined by a m.p.t. 24 43
Upcrossing lemma 104
Van der Waerden, Bartel L. 162
van der Waerden’s theorem 162 167 171 172
Varadarajan, V - S. 161
Varadhan, S. 133
Varga, Richard 7
Variational Principle 264 268 269 273
Veech, William A. 160 161
Very weakly Bernoulli 278 279
Ville, Jean 91
Virtual group 55
von Neumann - Kakutani adding machine 209 211
von Neumann, John 3 17 23 55 64 65 80 99 139 188
von Neumann’s Theorems on Lebesgue spaces 17 71
Walters, Peter 267
Wandering point 151
Wandering set 38 41 126
Weak law of large numbers 285
Weak mixing 57 58 64 65 152
Weak mixing, and density 0 73
Weak mixing, and eigenfunctions 64 72
Weak mixing, characterizations of 65 180
Weak mixing, of higher degree 151 163 179
Weak mixing, of roots and powers 72
Weak mixing, topological 151 152 180 210 212 214
Weak mixing, without strong mixing 71 210 212 214 217 219 220
Weak topology (on group of m 71 73
Weak-type ( , , 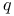 ) inequality 91 ) inequality 91
Weak-type (1,1) inequality 113 115 119 Maximal
Weakly Bernoulli 276 278 279
Weakly wandering set 41
Weiss, Benjamin 151 164 173 187 253 279 280
Weiss, Guido 107
Weyl, Hermann 50 149 156 158
White, H. E., Jr 72
Wiener, Norbert 27 75 76 87 88 91 102 108 114
Wright, Fred B. 39 46
Yosida. K saku 25 27 76 91 saku 25 27 76 91
Zermelo, E. 35
Zero entropy 244
Zimmer, Robert J. 55
Zygmund, A. 107
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 di - Furstenberg Theorem
di - Furstenberg Theorem  ,
,  ) inequality
) inequality  saku
saku