Авторизация
Поиск по указателям
Hughes B.D. — Random walks and random enviroments (Vol. 1. Random walks)
Обсудите книгу на научном форуме Нашли опечатку?
Название: Random walks and random enviroments (Vol. 1. Random walks)Автор: Hughes B.D. Аннотация: Volume 1 can be read without reference to Volume 2. In Volume 1, I expect of the reader only a modest facility in classical analysis, including the theory of functions of a complex variable up to contour integration. Those elements of probability theory which are needed are introduced in Chapter 1 of Volume 1, so that although an acquaintance with elementary
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1995Количество страниц: 631Добавлена в каталог: 13.10.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Gamma function, duplication formula 570 Gamma function, properties 570—571 Gamma function, reflection formula 570 Gamma function, relatives of 571—573 Gap dimension 17 Gauss' transformation of elliptic integrals 576 Gaussian density as limiting density 193 Gaussian density, defined 37 189 Gaussian density, only two nonzero cumulants 374 Generalized double diffusion 225 Generalized master equation, derived from projection operators 293—295 Generalized master equation, introduced 291—293 Generalized master equation, Klafter and Silbey derivation 304 Generalized master equation, related to continuous-time random walk 295—299 Generalized Mean Value Theorem 198 383 Generating function, first-passage time distribution 120 Generating function, introduced 117 Generating function, number of self-avoiding polygons 474 495 Generating function, number of self-avoiding trails 495 Generating function, number of self-avoiding walks 466 474 495 Generating function, recovery of original sequence 117—119 Generating function, site occupancy probability 120 Gram — Charlier expansions 65 71 82 104 Grand partition function for self-avoiding walk 467 Graph 19 Group without relations 23 Growth constant for lattice animals 503 Guest site 304 Hamiltonian walks 507 Hammersley's criterion 446 Hammersley's Theorem for polygons 440 Hammersley's Theorem on self-avoiding walks with prescribed end points 444 Hankel inversion theorem 67 72 578 Harmonic dimension and random walks 335 Harmonic dimension of a continuum 234 Harmonic dimension of Sierpinski lattice 30 Harmonic dimension, defined by lattice vibrations 29 Hausdorff measure of a set 9 Hausdorff — Besicovitch dimension 8 Heaviside's unit step function 90 Heun function 614 Hexagonal close-packed lattice, Green function for 610 Hexagonal close-packed lattice, return probability 153 Hexagonal lattice see "Honeycomb lattice" high-temperature expansion 537 Holomorphic function 524 569 Homogeneous function 527 Homogeneous lattice self-avoiding walk 424 Homogeneous lattice walk, defined 121 332 Homogeneous lattice walk, implications for 333—335 Homogeneous lattice walk, implications for 332 Homogeneous lattice walk, implications for 342 Homogeneous lattice walk, implications on generating functions 121 Homogeneous lattice walk, lemma on strong transience 127 Honeycomb lattice as a non-Bravais lattice 22 Honeycomb lattice, connective constant predicted 481 Honeycomb lattice, equivalent to brick wall 180 Honeycomb lattice, Green function evaluated 603 Host site 304 Husimi trees 25 Hypercubic lattice, defined 21 Hypercubic lattice, return probability 153 Hypercubic lattice, structure function for 139 Hypergeometric function, defined 574 Hypergeometric function, differential equation for 575 Hypergeometric function, generalized 581 Hypergeometric function, linear transformation 574 Hypergeometric function, quadratic transformation 574 Hyperscaling inequalities 535 Hyperscaling relation for n-vector model 529 Hyperscaling relation for self-avoiding walk 453 Hyperscaling relation, evidence for in self-avoiding walk 484 Independence of events 31 Independence of random variables 34 Infimum (inf) 9 423 Infinitely divisible 208 Infra-red bound 449 Integrated density of states 28 422 Internal energy per site 526 Internal states, random walk with 177 Irreversible traps, example of defective sites 165 Irreversible traps, randomly distributed see "Rosenstock model" Ising model as special case of n-vector model 525 Isotropic walks 59 Jensen's inequality, stated and proved 45—46 Jensen's inequality, used 373 Joint probability, density function 34 Joint probability, distribution function 34 Kasteleyn's walk problem 507—508 Kesten's Pattern Theorem 504—505 Killing rate 123 Killing time 124 Kinetic walk models 419 Koch curve 13 Kohlrausch relaxation function 264 Kolmogorov's equation 209 Kronecker — Weyl Equidistribution Theorem 84 L-lattice 493 Lace expansion for lattice animals 503 Lace expansion for lattice trees 504 Lace expansion for self-avoiding walk 426 449 Lace expansion for weakly self-avoiding walk 396 Lacunary, Fourier series 218 220 Lacunary, power series 218 Landau symbols xix Landen's transformation (of elliptic integrals) 576 Langevin function 103 Laplace transform 193 Laplace transform and moments 44 Laplace transform, defined 43 Laplace transform, inversion formula 204 Laplace Transform, Shift Theorem 291 Laser speckle 81 Lattice animals 502 Lattice Green function, evaluated 599—615 Lattice Green function, history of three-dimensional 604 Lattice Green function, introduced 132 Lattice trees 504 Lattice walk see also "Polya walk" Lattice walk, asymptotic analysis of structure function 595—599 Lattice walk, biased 158—160 Lattice walk, conditions for transience or recurrence 144 Lattice walk, dimensionality effects 145—154 Lattice walk, evolution equations 131 Lattice walk, historical notes 111 Lattice walk, reversible 130 Lattice walk, simple one-dimensional 140—142 Lattice walk, structure function see "Structure function" Lattice, Bethe 22 113 Lattice, Body-centred cubic 140 Lattice, Bravais 22 Lattice, Covering 496 507 Lattice, diamond 153 179 Lattice, directed 20 Lattice, Face-centred cubic 140 Lattice, fractal 25 Lattice, hypercubic 21 111 117 132 136 139 Lattice, introduced 19 Lattice, linear chain 21 Lattice, periodic 21 Lattice, self-similar 25 Lattice, Sierpinski 25 314 335 359 390 515 Lattice, simple cubic 21 Lattice, square 20 21 Lattice, terminology 19 Lattice, truncated tetrahedron 27 Lattice, vectors 21 Law of Large Numbers 401 Law of Large Numbers, strong 402 Law of large numbers, weak 45 402 Lawler's Theorem 130 Leaper, asymptotic behaviour 288 Leaper, defined 286 Leaper, model for turbulent dispersion 289 Lebesgue — Stieltjes integrals 33 Lemma of Dvoretzky and Erdos 332 342 Lemma of Hughes et al. 214 Lemma on strong transience 127 Lemma on subadditive sequences 423 Lemma on the asymptotic form of finite sums 332 Lemma, loop and chain 536 Lemma, Sarma's 536 Levy density see "Stable density" Levy, Paul 199 Lexicographic ordering 441 Lifetime in presence of traps 374 Linear chain, defined 21 Linear chain, Polya walk on 140 Linear independence of angular frequencies 84 Local fractal dimension 421 Local time 366 393 Localization problem 34 Loop and chain lemma 536 Loop erasure 431 Loop-erased self-avoiding walk 505 Lorenz equations 5 Magnetization in n-vector model 524 Manhattan lattice 493 Marginal densities 34 Marginal distributions 35 Markov chain for random walk on finite lattice 161 Markov chain, little used in this work 48 Markov chain, taboo probabilities 168 Markov chain, terminology 122 Markovian, nature of master equation 291 Markovian, process 3 412 Martingale, not introduced in this work 48 Mass dimension 15 Master equation with random coefficients 299—300 Master equation, introduced 289—291 Matrix see also "Tensor" Matrix, notation for walk with internal states 177 Matrix, use of boldface symbol 117 Maximum displacement of random walk 331 Maxwell — Boltzmann distribution 229 390 Mean 36 Mean field theory for n-vector model, critical temperature predicted 533 Mean field theory for n-vector model, derived 530—533 Mean field theory for n-vector model, exponents predicted, 533—534 Mean field theory for n-vector model, exponents predicted, 534 Mean motion 85 Mean value 36 Mean value theorem 198 383 Mean-square 36 Measure theory, avoided 46 Mellin convolution theorem 591 Mellin inversion theorem 588 Mellin transform and asymptotic expansions 588—592 Mellin transform, definition and properties 586—587 Memory kernel 292 Meromorphic function 569 Method of steepest descent 98 Meyer model 414 Modified Bessel function, first kind 579 Modified Bessel function, third kind 580 Moment of inertia tensor 97 Moments 36 Monodisperse 417 Monomer activity 467 Monte Carlo method for self-avoiding walks or polygons, algorithms 464—468 Monte Carlo method for self-avoiding walks or polygons, history 464 Monte Carlo method for self-avoiding walks or polygons, limitations 464 Monte Carlo method for self-avoiding walks or polygons, sources of data 464 Mosquitos 80 Multifractal 16 Mutually exclusive events 31 n-vector model, critical exponents, 527 n-vector model, critical exponents, 527 n-vector model, critical exponents, 525 n-vector model, critical exponents, 525 n-vector model, critical temperature 524 n-vector model, free energy 523 n-vector model, introduced 523 n-vector model, magnetization 524 n-vector model, scaling theory 525—527 n-vector model, spontaneous magnetization 524 n-vector model, susceptibility 525 Nearest-neighbour 19 Neighbour-avoiding walk 497 networks 19 Nienhuis' loop model, defined 542 Nienhuis' loop model, exact results for honeycomb lattice 544 Nienhuis' loop model, relation to self-avoiding polygons 543 Non-Markovian process 3 Nondifferentiable function, Riemann's 220 Nondifferentiable function, Weierstrass' 219 Noninteracting walkers 129 154 Normal density see "Gaussian density" Number of distinct sites visited, introduced 329 Number of distinct sites visited, mean and fractal dimension of random walk paths 210 Number of distinct sites visited, mean, Dvoretzky — Erdos analysis 331—333 Number of distinct sites visited, mean, for d 339 Number of distinct sites visited, mean, for d = 1 336—338 Number of distinct sites visited, mean, for d = 2 338—339 Number of distinct sites visited, mean, for strongly transient walks 340—341 Number of distinct sites visited, mean, generating function analysis 333—336 Number of distinct sites visited, mean, when mean-square displacement per step is 339—340 Number of distinct sites visited, variance for d = 2 357 Number of distinct sites visited, variance for d = 3 353—357 Number of distinct sites visited, variance, Dvoretzky — Erdoes inequalities 341—343 Number of distinct sites visited, variance, when asymptotically linear 344—346 Number of polygons per lattice site 440 o() Landau symbol xix O() Landau symbol, defined xix O() Landau symbol, notational clash 542 O(N) symmetry 542 Ornstein — Zernike theory for 534 Pair correlation function as 538 Pair correlation function at critical temperature 529 Pair correlation function, defined 528 Pair correlation function, scaling theory for 529—530 Partition function 331 390 523 Partition function, reduced 392 Partitions, theory of applied to spiral self-avoiding walk 510 Partitions, theory of applied to unfolding walks 438 Partitions, theory of need for provokes pessimism 418 Pausing-time density see "Waiting-time density" Peano curve 6 Pearson's walk after many steps 70—71 Pearson's walk, applications, crystallography 85 Pearson's walk, applications, laser speckle 81 Pearson's walk, applications, migration 80 Pearson's walk, applications, Monte Carlo integration 85 Pearson's walk, applications, spherical model 85 Pearson's walk, applications, vibrations 83 Pearson's walk, applications, wave superposition 80 Pearson's walk, closed-form solutions 68—70 Pearson's walk, equivalent to wave superposition 80 Pearson's walk, integral solutions 66—67 Pearson's walk, model of laser speckle 81 Pearson's walk, model of migration 80 Pearson's walk, numerical solutions 71—73 Pearson's walk, variable step-length 73—75 Pearson's walk, vibrations of unequal frequency 83—85 Pearson's walk, winding angle 76—79 Pearson, Karl 53 Penrose tiling 484 Percolation theory, as canonical model of random system 3 Periodic boundary conditions 28 161 163 537 Periodic lattice 21
Реклама
 |
|
О проекте
|
|
О проекте






 ,
,  ,
,  ,
, 
 ,
, 
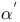
 ,
, 
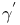
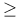 3
3 
 generates self-avoiding walks
generates self-avoiding walks