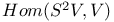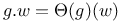|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Bertram W. — The Geometry of Jordan and Lie Structures (Lecture Notes in Mathematics) |
|
|
 |
| Предметный указатель |
 - JTS T in the form - JTS T in the form 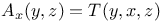 VII.2.4 VII.2.4
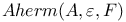 - space of A-skew-Hermitian matrices I.6 - space of A-skew-Hermitian matrices I.6
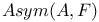 - space of A-skew-symmetric matrices I.6 - space of A-skew-symmetric matrices I.6
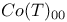 - set of - set of  with g (0) = 0, with g (0) = 0, 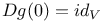 VIII.2 VIII.2
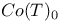 - set of - set of  with g (0) = 0 VIII.2 with g (0) = 0 VIII.2
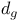 - denominator of a conformal transformation g VIII.1 - denominator of a conformal transformation g VIII.1
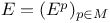 - Euler operator on M VII.1 - Euler operator on M VII.1
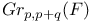 - Grassmannian variety VIII.4 - Grassmannian variety VIII.4
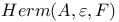 - space of Hermitian matrices I.6 - space of Hermitian matrices I.6
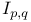 - diagonal matrix with signature (p, q) I.6 - diagonal matrix with signature (p, q) I.6
 - vector field extension of - vector field extension of 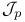 VI.1 VII.1 VI.1 VII.1
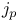 - affine extension of - affine extension of 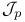 VI. 1 VI. 1
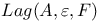 - variety of Lagrangian subspaces VIII.4 - variety of Lagrangian subspaces VIII.4
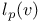 - vector field extension of a tangent vector v I.2 I.A - vector field extension of a tangent vector v I.2 I.A
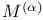 - -  -modification of the space belonging to a JTS T X.1 -modification of the space belonging to a JTS T X.1
 - twisted complexification (or "Hermitification") of M III.4.3 - twisted complexification (or "Hermitification") of M III.4.3
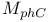 - twisted para-complexification of M III.4.3 - twisted para-complexification of M III.4.3
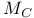 - straight complexification of M III.4.3 - straight complexification of M III.4.3
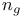 - numerator of a conformal transformation VIII.1 - numerator of a conformal transformation VIII.1
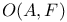 - orthogonal group w.r.t. A I.6 - orthogonal group w.r.t. A I.6
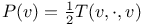 - "quadratic representation" of a JTS T VIII.2 - "quadratic representation" of a JTS T VIII.2
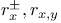 - "multiplication by - "multiplication by  w.r.t. a point (x, y)" VI.3 w.r.t. a point (x, y)" VI.3
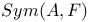 - space of symmetric matrices I.6 - space of symmetric matrices I.6
 - Symmetry w.r.t. a point x in a symmetric space I.3 - Symmetry w.r.t. a point x in a symmetric space I.3
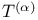 - -  -modification of a JTS T III.4 -modification of a JTS T III.4
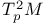 - second order tangent space I.B - second order tangent space I.B
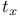 - translation by a vector x I.A - translation by a vector x I.A
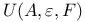 - A-unitary group I.6 - A-unitary group I.6
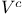 - conformal completion of V VIII.3 - conformal completion of V VIII.3
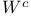 - conformal completion of W VIII.3 - conformal completion of W VIII.3
 - Cayley transform XI.2 - Cayley transform XI.2
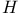 - skew field of quaternions I.6 - skew field of quaternions I.6
 - quadratic vector field - quadratic vector field 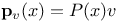 VIII.2 VIII.2
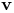 - constant vector field I.A.1 - constant vector field I.A.1
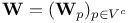 - structure bundle VIII.3 - structure bundle VIII.3
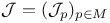 - almost complex structure or polarization on M III.1 - almost complex structure or polarization on M III.1
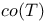 - conformal Lie algebra of a JTS T VII.1 - conformal Lie algebra of a JTS T VII.1
 - conformal Lie algebra of a Jordan algebra V VII.1 - conformal Lie algebra of a Jordan algebra V VII.1
 - invariance algebra of - invariance algebra of  VI.A VI.A
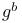 - inner conformal Lie algebra VII.1 - inner conformal Lie algebra VII.1
 - Lie algebra of P VIII.3 - Lie algebra of P VIII.3
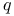 - -1-eigenspace in an involutive Lie algebra; LTS I.1 - -1-eigenspace in an involutive Lie algebra; LTS I.1
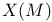 - Lie algebra of vector fields on M I.A.1 - Lie algebra of vector fields on M I.A.1
 - multiplication map of a symmetric space I.4 - multiplication map of a symmetric space I.4
 - affine connection I.B - affine connection I.B
 - open symmetric orbit in a vector space II.1 - open symmetric orbit in a vector space II.1
 - involution of Co (T) and of - involution of Co (T) and of 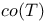 VII.2 VIII.2 VII.2 VIII.2
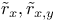 - exponential of a twisted polarization VI.2 - exponential of a twisted polarization VI.2
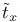 - exponential of the quadratic vector field - exponential of the quadratic vector field 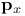 VIII.2 VIII.2
Affine connection I.B
Affine locally symmetric space I.2
Affine map I.B
Affinization VIII.3
Almost complex structure III.1
Associative algebra II.3
Associative form V.4
B (x, y) - Bergman operator VIII.2
Bergman operator VIII.2
Borel imbedding VII.3 X.1
c-dual I.1
Canonical connection I.2
Cartan involution X.6
Casual diffeomorphism IX.2.3
Casual group IX.2
Casual intervals XI.3
Casual Makarevic space XI.3
Casual structure IX.2
Casual symmetric space XI.3
Causal, compactly-, non-compactly- XI.3
Cayley transform I.6 XI.2
Cayley type spaces XI.3
Circled space VI.1
Classical group I.6
Co (T) - conformal group of a JTS T VIII.1
Co (V) - conformal group of a Jordan algebra V VIII.2
Co' (T) - set of  with with 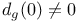 VIII.2 VIII.2
Complexification diagram III.4
Complexification, straight III.1
Complexification, twisted III.2
Cone, regular- IX.2.3
Cone, symmetric V.5
Conformal compactification VIII.3
Conformal completion VIII.3
Conformal group VIII.1
Conformal Lie algebra VII.1
Conformal map (T-, G-) VIII.1
Conformally equivalent XI.5
Conjugation, complex III.1
Connected pair III.3
Curvature tensor I.2 I.B
Denominator VIII.1
density X.6
Der (V, A) - derivation algebra of a bilinear map 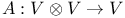 II.1 II.1
Displacement group I.3
Elliptic realization of a cone XI.3
Elliptic tripotent X.5
Equivariant map I.1
Euclidean Jordan algebra V.5
Euler operator I.A VII.1
Exp - exponential map of a connection or of a symmetric space I.B
exp - exponential map of a Lie group I.5
Exponential map I.B X.4
Extension of a tangent map I.A
Extension of a vector field I.A
F - symmetric matrix I.6
Faithful JTS VII.2
First kind XI.1
Fundamental formula II.2 VIII.C
Fundamental theorem IX.1 IX.2
G (M) - group of displacements I.3
Geodesic symmetry I.3
Global polarized space VIII.3
Global space X.2
Graded Lie algebra III.3
Graph VIII.4
Grassmannian VIII.4
Harish-Chandra imbedding VII.2 X.2
| Helwig-space II.4 XI.4
Hermitian (para-)complexification III.4
Hermitian JTS III.2
Hermitian symmetric space V.5
Homomorphism of symmetric spaces I.2 I.4
Hyperbolic functions on a JTS X.4
Hyperbolic realization of a cone XI.3
Hyperbolic space I.6
Hyperbolic tripotent X.5
Integrability VI.A
Interval (causal) XI.3
Invertible element XI.1
J - complex structure or polarization on a LTS III.1 III.3
j - Jordan inverse II.2
J - standard symplectic matrix I.6
jet VIII.2
Jordan algebra II.2
Jordan coordinates VII.2
Jordan extension III.4
Jordan inverse II.2
Jordan pair III.3
Jordan triple system (JTS) III.2
Jordan — Lie functor III.4
L (v) - operator of left multiplication by v II.1
Lagrangian I.6
Lie functor I.1
Lie group I.1
Lie triple algebra II.1
Lie triple system (LTS) I.1
Linear relation IX.2
Liouville theorem IX.1
Makarevic space XI.1
Modification (of a JTS) III.4
Multiplication map I.4
Non-degenerate JTS V.4
Numerator VIII.1
Orbit, open symmetric- II.1 X.1
Orbit, symmetric- II.1
P - stabilizer of 0 in Co (T) VIII.3
P' - intersection of Co (T) with the affine group of V VIII.3
Para-conjugation III.3
Para-Hermitian symmetric space III.4
Para-real form III.3
Parabolic realization of a cone XI.3
Paracomplex structure III.3
Peirce decomposition X.5
Polarisation III.3
Polarized LTS, JTS III.3
Positive JTS V.5
Power associative II.2 X.A
Powers in a Jordan algebra II.2
Powers in a symmetric space I.5
Prehomogeneous symmetric space II.1
Prehomogeneous vector space II.1
Projective (T-) VII.2 VIII.
Pseudo-Hermitian symmetric space V.4
Pseudo-metric V.1 X.6
Q - quadratic map of a symmetric space I.5
Quadratic map I.5
Quadratic prehomogenous symmetric space II. 2
Quadratic representation II.2
Quasi-inverse VIII.2
R - curvature tensor, Lie triple product I.1 I.2
Real Cayley transform I.6 XI.2
Real form III.1
Relative invariant V.4
Representation of a symmetric space I.5
Representation, polynomial II.2
Ric - Ricci-tensor V.1
Ricci form V.1
Ruled space VI.2
Semisimple JTS V.4
Semisimple LTS V.1
Semisimple symmetric space V.1
Siegel space I.6
Simple JTS V.4
Simple LTS V.1
Sphere I.6 IV.1
Standard imbedding I.1
Str (T) - structure group of a JTS T VIII.1
Str (V) - structure group of a Jordan algebra V II.3
Straight complexification III.1
Structure algebra II.2 VII.2
Structure bundle VIII.3
Structure group II.2 VIII.
Structure monoid VIII.1
Structure tensor III.2
Structure variety III.4
Svar (T) - structure variety of a JTS T III.4
Symmetric cone V.5
Symmetric Lie algebra I.1
Symmetric pair I.1
Symmetric R-space V.5 X.6
Symmetric space with twist III.4
Symmetric space, (pseudo-)Hermitian V.4
Symmetric space, (pseudo-)Riemannian V.1
Symmetric space, (straight) complex I.1 III.1
Symmetric space, algebraic I.4
Symmetric space, para-Hermitian V.4
Symmetric space, polarized III.3
Symmetric space, topological I.4
Symmetric space, twisted complex III. 2
Symmetric submanifold II.4
T - structure tensor, Jordan triple product III.2 III.3 III.4
Trace form V.2 V.4
Tripotent X.5
Tube domain XI.2
Twisted complex structure III.1
Twisted complexification III.4
Twisted para-complexification III.4
Twisted polarization III.3
Underlying Jordan pair IV.2
V' - set of invertible elements in a Jordan algebra V II.2
Vector field extension I.A
W - subspace 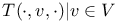 of of 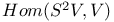 VII.2 VII.2
W - vector space V with Str (T)-action by 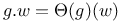 II.2 II.2
[X, Y, Z] - Lie triple product I.1
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 - JTS T in the form
- JTS T in the form 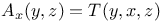
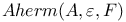 - space of A-skew-Hermitian matrices
- space of A-skew-Hermitian matrices 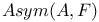 - space of A-skew-symmetric matrices
- space of A-skew-symmetric matrices 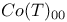 - set of
- set of  with g (0) = 0,
with g (0) = 0, 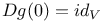
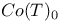 - set of
- set of 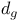 - denominator of a conformal transformation g
- denominator of a conformal transformation g 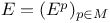 - Euler operator on M
- Euler operator on M 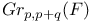 - Grassmannian variety
- Grassmannian variety  - space of Hermitian matrices
- space of Hermitian matrices 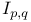 - diagonal matrix with signature (p, q)
- diagonal matrix with signature (p, q)  - vector field extension of
- vector field extension of 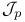
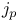 - affine extension of
- affine extension of 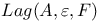 - variety of Lagrangian subspaces
- variety of Lagrangian subspaces 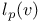 - vector field extension of a tangent vector v
- vector field extension of a tangent vector v 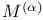 -
-  -modification of the space belonging to a JTS T
-modification of the space belonging to a JTS T  - twisted complexification (or "Hermitification") of M
- twisted complexification (or "Hermitification") of M 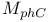 - twisted para-complexification of M
- twisted para-complexification of M 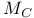 - straight complexification of M
- straight complexification of M 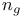 - numerator of a conformal transformation
- numerator of a conformal transformation 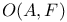 - orthogonal group w.r.t. A
- orthogonal group w.r.t. A 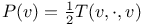 - "quadratic representation" of a JTS T
- "quadratic representation" of a JTS T 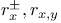 - "multiplication by
- "multiplication by  w.r.t. a point (x, y)"
w.r.t. a point (x, y)" 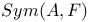 - space of symmetric matrices
- space of symmetric matrices  - Symmetry w.r.t. a point x in a symmetric space
- Symmetry w.r.t. a point x in a symmetric space 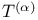 -
- 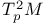 - second order tangent space
- second order tangent space 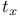 - translation by a vector x
- translation by a vector x 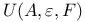 - A-unitary group
- A-unitary group 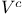 - conformal completion of V
- conformal completion of V 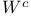 - conformal completion of W
- conformal completion of W  - Cayley transform
- Cayley transform 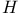 - skew field of quaternions
- skew field of quaternions  - quadratic vector field
- quadratic vector field 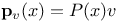
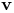 - constant vector field
- constant vector field 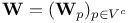 - structure bundle
- structure bundle 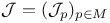 - almost complex structure or polarization on M
- almost complex structure or polarization on M 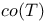 - conformal Lie algebra of a JTS T
- conformal Lie algebra of a JTS T  - conformal Lie algebra of a Jordan algebra V
- conformal Lie algebra of a Jordan algebra V  - invariance algebra of
- invariance algebra of 
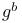 - inner conformal Lie algebra
- inner conformal Lie algebra  - Lie algebra of P
- Lie algebra of P 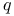 - -1-eigenspace in an involutive Lie algebra; LTS
- -1-eigenspace in an involutive Lie algebra; LTS 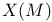 - Lie algebra of vector fields on M
- Lie algebra of vector fields on M  - multiplication map of a symmetric space
- multiplication map of a symmetric space  - affine connection
- affine connection  - open symmetric orbit in a vector space
- open symmetric orbit in a vector space  - involution of Co (T) and of
- involution of Co (T) and of 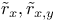 - exponential of a twisted polarization
- exponential of a twisted polarization 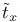 - exponential of the quadratic vector field
- exponential of the quadratic vector field 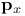
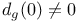
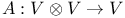
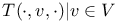 of
of