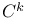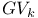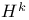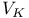|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Almgren F.J. — Plateau's Problem: An Invitation to Varifold Geometry |
|
|
 |
| Предметный указатель |
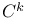 21 21
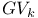 51 51
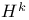 15 15
 43 43
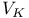 43 43
Analytic sets 18
Area 16 18
Calculus of variations 54
Covectors 18—21
Critical point 54
Current 37
Current, integral 5 39
Current, rectifiable 37
Diameter of regular varifold 70
Disk 2
Disk with handles 3
Disk with spines 42—43
Distance see "Metric"
Douglas, J. 2 3 71
Dual vector space 18-21
f 45
Federer, H. 5 67 71
Flat chains with coefficients in a group 5
Fleming, W.H. 4 5 69 71
Force diagram method 62
Intersection of varifolds see "Varifold operations"
Isoperimetric inequalities 65
Lebesgue measure 18
Length 16 18
Lipschitz condition 14—15 21
M 46
Mass 50
Metric 22 see
Metric, distance 22
Minimal surface 64
Moebius band 5—7
Moebius band, triple 5—7
Monotonicity for regular vari 69—70
Multiplicities 3 39—41 47
P 59
Partial ordering of varifolds see "Varifold operations"
Plateau, J. 1 71
| Plateau’s construction problem 2
Plateau’s existence problem 2
Plateau’s problem 1
Projective space 22
Rectifiable set 30—33
Rectifiable set, oriented 32—33
Reifenberg, E.R. 4 69 71 72
S 55
Singular set 13
Soap bubbles 1—2 57 60 65
Soap films 1—2 7-—13 56—57 60 65
Soap films, not touching all of boundary wire 7—9 65 68
Stationary point 54
Stationary varifold see "Varifold"
Stokes’ Theorem 39
Sum of varifolds see "Varifold operations"
Support, of differential form 21
Support, of varifold 43
Surface tension 9
Suslin sets 18
t 58
Union of varifolds see "Varifold operations"
V 50
Variation 55
Varifold metrics 45 46
Varifold operations, intersection 44
Varifold operations, partial ordering 44
Varifold operations, sum 44
Varifold operations, union 44
Varifold pairs 59
Varifold pairs, regular 59
Varifold pairs, stationary 59
Varifold pairs, stationary, touching all of boundary 67
Varifold, axioms for 38 43
Varifold, definition 43
Varifold, elementary 50-51
Varifold, geometric 51
Varifold, integral 1 38 47
Varifold, rectifiable 38
Varifold, stationary 55 57
w 47
X 53
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте