Авторизация
Поиск по указателям
Wong K. — Asymptotic Approximations of Integrals
Обсудите книгу на научном форуме Нашли опечатку?
Название: Asymptotic Approximations of IntegralsАвтор: Wong K. Аннотация: Asymptotic methods are frequently used in many branches of both pure and applied mathematics, and this classic text remains the most up-to-date book dealing with one important aspect of this area, namely, asymptotic approximations of integrals. In Asymptotic Approximations of Integrals, all results are proved rigorously, and many of the approximation formulas are accompanied by error bounds. A thorough discussion on multidimensional integrals is given, and references are provided. Asymptotic Approximations of Integrals contains the "distributional method," which is not available elsewhere. Most of the examples in this text come from concrete applications.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2001Количество страниц: 543Добавлена в каталог: 13.12.2009Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Abel limit 198 Abel summability 198 Abscissas 222 Airy function 46 51 90 133 370 Airy function, asymptotic expansion of 47 93 Airy function, differential equation for 91 Airy function, generalized 386 Airy function, integral of 46 91 Airy function, of negative argument 220 Airy function, relation to Bessel functions 220 Airy integral (see “Airy function”) Amplitude function 429 Anger function 418 Anger function 418 Anger’s function 82 Asymptotic of Poincare type 5 11 Asymptotic of power series form 11 Asymptotic, compound 11 Asymptotic, equalities 2 Asymptotic, expansions 5 Asymptotic, failure of 18 Asymptotic, formulas (see “Asymptotic equalities”) Asymptotic, generalized 10 Asymptotic, sequences 10 Asymptotic, uniform 353 356 Asymptotics 1 Asymptotics, paradox in 146 Barnes’ lemma 48 229 355 Bernoulli numbers 2 32 Bernoulli polynomials 32 Bernoulli polynomials, generalized 47 Bessel function 24 45 Bessel function of large argument 24—26 112—116 134 Bessel function of large order 98 100 102—103 Bessel function of the second kind 236 Bessel function, asymptotic expansion of 227 Bessel function, asymptotic formulas 205 206 Bessel function, integral representation of 24 97 112 134 417 Bessel function, modified 50 225 Bessel function, relation to Hankel functions 97 211 227 Bessel function, Schlaefli’s integral representation for 97 Bessel function, series representation 231 Bessel transforms 221 Binet — Cauchy product formula 510 Catastrophy theory 388 Cauchy limit 500 Cauchy principal value 313 Caustics 392 Central limit theorem 141 Complementary error function 42 218 Complementary error function, asymptotic expansion of 42 Completeness of 248 Confluent hypergeometric function 46 Confluent hypergeometric function, asymptotic expansion of 46 Convergence in 247 265 Convergence in 261 267 Convolutions 254 Convolutions differentiation of 255 Convolutions of distributions 241 255 Cosine integral 513 Critical points 281 424 478 Critical points of the first kind 426 Critical points of the second kind 426 442 478 Critical points of the third kind 426 448 Cubic transformation of Chester,Friedman & Ursell 356 367 Curvature of an arc 510 Darboux approximant 117 Darboux’s method 116 145 146 Debye’s method 84 87 Delta function (see “Dirac delta function”) Derivatives (of distributions) 249 266 Derivatives (of distributions) of convolutions (see “Convolutions”) Derivatives (of distributions) of Fourier transforms 274 276 Diffeomorphisms 479 Differentiation under an integral sign 327 Dirac delta function 244 266 Dirac delta function, derivative of 252 283 Dirichlet — Mehler integral 420 Dispersive wave theory 83 Distributional approach 293 351 Distributional/Weak limit 247 Distributional/Weak limit in 247 Distributional/Weak limit in 262 Distributions 243 265 Distributions, 268 Distributions, 270 272 Distributions, 258 263 272 Distributions, concentrated on a set 280 282 Distributions, convergence of (see “Convergence”) Distributions, convolution of (see “Convolution”) Distributions, derivative of (see “Derivatives”) Distributions, equal in an open set 245 Distributions, in 265 Distributions, multiplication by a 248 Distributions, regular 243 Distributions, singular 243 Distributions, support of 245 Distributions, surface 241 280 Distributions, translation of 249 Distributions, vanishing of 247 Distributions, zero 244 Divided differences 223 Dominant (exponentially increasing) 94 Double integrals 176 423 Edgeworth series 141 146 Elementary symmetric functions in theory of equations 385 Elliptic function (of Weierstrass) 391 Elliptic integrals of the first kind 345 Error function (see “Complementary error function”) Error test 38 Euler — Maclaurin summation formula 32 Euler’s limit formula 51 Exponential integral 14 29 Exponential integral, asymptotic expansion of 15 30 Exponentially small 6 355 Fourier integral 15 195 198 210 221 Fourier integral, asymptotic expansion of 16 199 Fourier integral, error bound for 201 Fourier transform of 287 Fourier transform of translated 244 285 Fractional integral 326 Fractional integral, asymptotic expansion of 328 330 Fractional integral, error bound for 332 Fresnel integral 218 332 362 411 Fresnel Integral, Mellin transform of 219 Functional, continuous linear 242 Functional, continuous linear on 243 265 Functional, continuous linear on 262 267 Furier transforms 147 221 274 276 323 Furier transforms of 278 279 Furier transforms of 277 Furier transforms of a tempered distribution 276 Furier transforms, n-dimensional 274 276 478 500 Furier transforms, n-dimensional, asymptotic expansion of 603 Furier transforms, n-dimensional, inverse 287 Furier transforms, near the origin 321 323 362 Furier transforms, Parseval identity for 287 Gamma function 60 (see also “Incomplete Gamma function”) Gamma function, asymptotic expansion of 62 110 135 Gamma function, asymptotic expansion of ratio 47 Gamma function, asymptotic formula for large imaginary argument 154 Gamma function, residues of 154 Gamma function, singularities of 321 Gauss — Laguerre quadrature formulae 222 Generalized functions 240 (see also “Distributions”) Geometric optics 388 Group velocity 83 Guass sum 203 Guassian quadrature 221 Hankel function 94 Hankel function, asymptotic expansion of 134 Hankel function, integral representation of 94 Hankel function, Mellin transform of 171 Hankel transform 147 203 210 502 Hankel transform, asymptotic expansion of 204—207 Hankel transform, error bound for 207—209 Harmonic numbers 1 38 Hayman’s admissible functions 123 Hayman’s admissible functions, distributional derivative of 262 Hayman’s formula 4 122 145 Heaviside function 252 282 Hessian matrix 478 Hessian of a function 436 Hilbert transform 187 312 Hilbert transform, asymptotic expansion of 314—316 Hills 87 Hironaka, theorem of 492 Incomplete Gamma function, asymptotic expansion of 43 Incomplete Gamma function, complementary 28 43 108 232 365 Incomplete Gamma function, uniform asymptotic expansion of 365 366 Klein — Gordon equation 84 144 Kontorovich — Lebedev transform 324 Lagrange resolvents for zeros of quartics 391 Lagrange’s inversion formula 61 64 93 Laguerre polynomials 222 372 396 421 Laguerre polynomials, uniform asymptotic expansion of 381 402 Laplace transforms 30 69 147 321 Laplace transforms, inverse 30 229 Laplace transforms, near the origin 167—171 322 Laplace-type integrals 424 459 467 494 Laplace-type integrals, asymptotic expansions of 461 467 495 498 Laplace’s approximation (or Laplace’s formula) 3 56 Laplace’s approximation (or Laplace’s formula), n-dimensional 494 508 516 Laplace’s approximation (or Laplace’s formula), two-dimensional 459 Laplace’s method 55 Laurent series 270 Laurent series for 273 Laurent series for 272 Lebesgue constants 1 2 40—42 Lebesgue constants, associated with polynomial interpolation 54 Legendre ftinction of the first kind 406 422 Legendre ftinction of the second kind 47 Legendre ftinction, uniform asymptotic expansion of 408 Legendre polynomials 141 146 420 Legendre polynomials uniform asymptotic expansion of 420 Legendre polynomials, asymptotic expansion of 141 Liouville — Green approximation 4 Locally absolutely continuous function 260 Logarithmic integral 43 Logarithmic integral, asymptotic expansion of 43 Logarithmic singularity 66 144 146 186 Maclaurin coefficients, asymptotic behavior of 116—127 145 Maclaurin expansion of 91 Mellin transform technique 147 351 Mellin transforms 147 Mellin transforms (relation with) bilateral Laplace transform 149 Mellin transforms, analytic continuation 153 Mellin transforms, convolution integral 147 151 333 352 Mellin transforms, generalized 337 Mellin transforms, inversion formula 147 151 Mellin transforms, Parseval formula (or Parseval identity) 148 189 Mellin transforms, properties of 149 Mellin transforms, table of 192—194 Mellin — Barnes type integrals 228 Method of steepest descents 84 144 145 Morse’s Lemma 479 Multiple integrals 177 477 Multiple Integrals, resolution of 280 Nesting property 281 Neutralizers 246 Open covering 246 Oscillatory kernels 216 Parabolic cylinder function 236 364 Parseval formula (see “Mellin transform & Fourier transform”) Partition of unity 246 Pearcey integral 388 395 Pearcey integral, uniform asymptotic expansion of 416 417 Perron’s method 103 145 406 Phase function 76 phase velocity 83 Phragm 6 Phragmen — Lindeloef theorem 166 Plemelj formula 313 Pochhammer notation 47 Positive definite functions 281 Principle of Stationary Phase 76 144 240 Quadrature formulae for Bessel transforms 226 Quadrature formulae for Fourier transforms 222 Quadrature formulae, Gauaa — Laguerre 222 Quartic transformation 392 Rapidly decreasing functions 261 266 Recessive (exponentially decreasing) 94 Regularization 267 Regularization at infinity 260 261 Regularization in finite interval 259 Regularization of a function 258 Regularization of an integral 258 336 Regularization, method of 333 352 Regularization, nonuniqueness 272 Resolution of singularities (see “Singularities”) Riemann-Lebesgue lemma, generalized 200 Riemann-Liouville fractional integral (see “Fractional integral”) Robinson’s conjecture 120 Saddle point 86 432 Saddle point of order m 87 Saddle point, many coalescing 383 Saddle point, near a pole 356 Saddle point, near an endpoint 360 Saddle point, relevant 89 Saddle point, two coalescing 366 Saddle-point method 103 Schlaefii’s integral 97 Schroedinger spectral kernel 230 Scorer’s function 419 Second fundamental form of differential, geometry 511 Signature of a matrix 480 Sine integral 513 Singularities, resolution of 492 Splitting lemma 491 512 Stationary phase (see “Principle of stationary phase”) Stationary phase approximation 77 240 Stationary phase approximation for double integrals 431 Stationary phase approximation, n-dimensional analogue 478 Stationary points 77 424 478 Stationary points, boundary 440 484 Stationary points, curve of 452 Stationary points, degenerate 435 491 Stationary points, non-degenerate 487 Steepest ascent 87 Steepest descent 86 87 Stiltjes transform 147 295 304 351 Stiltjes transform, asymptotic expansion of 186 Stiltjes transform, error bounds for 302—304 309 Stiltjes transform, generalized 185 Stirling formula 1 51 62 Stirling numbers 143 Stirling numbers, asymptotic expansion of 143 Stirling series 51 Stokes line 94 Stokes’ phenomenon 94 Summability method 195 216 Support of a distribution 245 Support of a function 242 Surface distribution 241 280 Swallowtail integral 388 389 Swallowtail integral, uniform asymptotic expansion of 395 Tangential contact, points of 487 Tauberian theorem (see “Laplace transforms”) Tauberian theorem for 230 Taylor series for 273
Реклама
 |
|
О проекте
|
|
О проекте



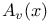
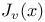

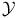


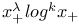
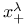

 -function
-function