Авторизация
Поиск по указателям
Arnold V.I. — Ordinary Differential Equations
Обсудите книгу на научном форуме Нашли опечатку?
Название: Ordinary Differential EquationsАвтор: Arnold V.I. Аннотация: Although there is no lack of other books on this subject, even with the same title, the appearance of this new one is fully justified on at least two grounds: its approach makes full use of modern mathematical concepts and terminology of considerable sophistication and abstraction, going well beyond the traditional presentation of the subject; and, at the same time, the resulting enhancement of mathematical abstractness is counterbalanced by a constant appeal to geometrical and physical considerations, presented in the main text and in numerous problems and exercises.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Издание: 1st editionГод издания: 1998Количество страниц: 280Добавлена в каталог: 10.12.2009Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
57 Admissible coordinate system(s) 34 Admissible coordinate system(s), class of 35 Analytic manifold 235 Andronov, A.A. 94n Asymptotic stability 156 201n Atlas(es) 235 Atlas(es), equivalent 235 Atlas(es), examples of 236—238 Auto-oscillations 92 Auto-oscillatory regime 92 Base of a tangent bundle 246 Beats 184 Bessel's equation 193 Brieskorn, E. 242n Bundle space 243n Cardano's Formula 32 Cauchy sequence 98n Center 127 133 Characteristic equation 116 Characteristic frequencies 175 Chebotarev, N.G. 160n Chebyshev polynomial 175 Chinn, W.G. 256n Clairauf s theorem 271 Clairaut equation 67 Class 6 Commutator 75 Comparison theorem 17 Complex amplitude 182 Complex conjugate operator 122 Complexification of a space 120 Complexification of an operator 120 Complexified equation 129 Configuration space 79 Conservative system, with one degree of freedom 79 Contraction mapping(s) 211 220 Contraction mapping(s), applications of 222 Contraction mapping(s), fixed point of 212 Contraction mapping(s), theorem 212 Convex domain 78n Coriolis force 66 Covariant functoriality 248 Critical point 81n Critical value 81n Curve(s), derivative of 122 Curve(s), index of 255 Curve(s), leaving a point 33 241 Curve(s), tangent 34 Curve(s), velocity vector of 33 34 Curve(s), velocity vector of, components of 33 Curve(s), with complex values 122 Decomplexification of a space 119 Decomplexification of an operator 120 Degree of a mapping 266 Degree of a mapping, at a regular point 264 Derivative in the direction of a field 73 Derivative in the direction of a vector 73 Derivative of a curve 122 Derivative of a mapping 37 Determinacy 1 Determinant of a matrix 111 Determinant of an operator 111 Determinant of the exponential of an operator 113 Determinant vs. trace 112 Diagonal operator 102 116 Diagonal operator, exponential of 102 Diffeomorphism 6 Diffeomorphism of a manifold 241 Differentiability 1 20n Differentiability and Lipschitz conditions 217 Differentiability theorem 53 Differentiability theorem for equation of order n 62 Differentiability theorem for nonautonomous case 57 Differentiable function 6 Differentiable manifold(s) 6 234—243 Differentiable manifold(s) of class 235 Differentiable manifold(s), compact subset of 238 Differentiable manifold(s), connected 239 Differentiable manifold(s), countability condition for 236 Differentiable manifold(s), curve on 241 Differentiable manifold(s), diffeomorphic 241 Differentiable manifold(s), diffeomorphism of 241 Differentiable manifold(s), differentiable mapping of 241 Differentiable manifold(s), differentiable structure on 235 Differentiable manifold(s), disconnected 239 Differentiable manifold(s), disconnected, connected components of 239 Differentiable manifold(s), examples of 233 243 Differentiable manifold(s), finite-dimensional 6 Differentiable manifold(s), open subset of 238 Differentiable manifold(s), parallelizable 246 Differentiable manifold(s), parallelized 246 Differentiable manifold(s), separability condition for 235 Differentiable manifold(s), simply connected 242n Differentiable manifold(s), tangent bundle of see "Tangent bundle" Differentiable manifold(s), tangent space to 244 Differentiable manifold(s), vector field on 248 Differentiable manifold(s), vector tangent to 243 Differentiable mapping 6 241 Differentiable mapping, derivative of 33 37 Differentiable structure 35 234 235 Differential algebra 32n Differential equation(s), basic theorem on 48 Differential equation(s), determined by a vector field 11 Differential equation(s), direct product of 24 Differential equation(s), direction field of 12 Differential equation(s), higher-order, systems of 170 Differential equation(s), integral curve of 12 31 Differential equation(s), integration of 32 Differential equation(s), linear see "Linear differential equations" Differential equation(s), nonautonomous 28—33 56—59 Differential equation(s), normal form of 67 Differential equation(s), of order n 59 171 Differential equation(s), of order n, solution of 59 Differential equation(s), on manifolds 233—268 Differential equation(s), phase curve of 12 Differential equation(s), phase space of 12 Differential equation(s), phase space of, extended 12 Differential equation(s), solution of see "Solutions of a differential equation" Differential equation(s), solved with respect to the highest derivative 67 Differential equation(s), system of 62 67 Differential equation(s), with separable variables 29 Differential equation(s), with variable coefficients 30 Differential operator, linear homogeneous 74 Differentiations as mappings 74 Direct product of differential equations 24 Direct product of sets 5 Direction field 12 Directional derivative 73 Dirichlet's s cell principle 164 Divergence 198 Eigenbasis 102 Eigenvalues of a linear differential equation, distinct 137—139 Eigenvalues of a linear differential equation, distinct real 115—119 Eigenvalues of a linear differential equation, multiple 167—176 Eigenvalues of a linear differential equation, purely imaginary 160—167 Elliptical rotations 133 Emde, F. 193n Energy, conservation of 77 81 Energy, kinetic 80 Energy, level curves of 81 Energy, level curves of, critical 90 Energy, level curves of, noncritical 87 Energy, potential 80 Energy, total 80 Equation of variations 64 186 223 224 Equilibrium position 5 12 95 Equilibrium position, asymptotic stability of 156 Equilibrium position, Lyapunov stability of 155 Euler characteristic 262 267 Euler formula 108 Euler line 110 222 Euler's theorem on polyhedra 262 Everywhere dense set 163n Existence theorem 50 Existence theorem for equation of order n 61 Existence theorem for nonautonomous case 57 Exponential of a complex number 108 Exponential of a complex operator 122 Exponential of a diagonal operator 102 Exponential of a nilpotent operator 103 Exponential of an operator 97 100 107 167 Exponential of an operator, determinant of 113 Exponential, group property of 104 Extended phase space 5 12 Extension of a solution 53 Extension of a solution, backward 53 Extension of a solution, for nonautonomous case 58 Extension of a solution, forward 53 Extension of a solution, of equation of order n 62 Extension of a solution, up to a subset 53 Fibonacci sequence 119 Filippov, A.F. 32n Finite-dimensionality 1 First integral 75 First integral, local 77 First integral, time-dependent 78 Fixed point of a flow 5 Fixed point of a mapping 201 211 Fixed point of a mapping, asymptotically stable 201n Fixed point of a mapping, stable, in Lyapunov's sense 201 Fixed point theorem 257 Focus 127 Focus, stable 127 Focus, unstable 127 Forced oscillations 183 Free oscillations 183 functors 121 Fundamental theorem of algebra 256 Galileo's law 9 Golubev, V.V. 227n Graph of a mapping 5 Hadamard's lemma 85 Half-life 16 Hamilton's equations 63 77 198 Hamiltonian system 203 Hedgehog theorem 247 Hessian matrix 262 Homeomorphism 141 Homogeneous coordinates 233 Hypergeometric equation 193 Imaginary plane 121 Index of a singular point 258 266 Index of an oriented closed curve 255 263 Index of an oriented closed curve, properties of 255—256 Infinitely differentiable functions, linearly independent 176 Infinitely differentiable functions, space of 176 Infinitesimal generator 107 Initial condition 12 30 Integral curve 5 12 31 Integral part 164n Invariant subspaces 131 Inverse function theorem 39 Involutory operator 121 Jacobi's identity 75 Jacobian matrix 38 Jahnke, E. 193n Jordan block 167 Jordan block, nilpotent 168 181 Kamke, E. 32n Kepler potential 83 Khaikin, S.E. 94n Konstantinov, N.N. 2 Kurosh, A.G. 160n Lavrentev, M.A. 160n Level set 76 Levine, H.I. 265n Lie algebra 75 Limit cycle 47 71 Limit cycle, stable 93 Limit cycle, unstable 93 Linear differential equations, basic theorem on 106 Linear differential equations, complexified 129 Linear differential equations, definition of 96 97 Linear differential equations, general solution of 138 Linear differential equations, homogeneous, with constant coefficients 97 Linear differential equations, homogeneous, with variable coefficients 188 Linear differential equations, in the plane 132 Linear differential equations, nonautonomous see "Nonautonomous linear equation" Linear differential equations, nonhomogeneous 179 Linear differential equations, nonhomogeneous, solution of 179 Linear differential equations, space of solutions of 177 Linear differential equations, space of solutions of, invariance of, under shifts 178 Linear differential equations, with complex phase space 124 Linear differential equations, with complex phase space, basic theorem on 124 Linear differential equations, with complex phase space, solution of 124 Linear differential equations, with periodic coefficients 199—208 Linear differential equations, with periodic coefficients, stability of solutions of 201 Linear operator(s), complex conjugate of 122 Linear operator(s), determinant of 111 Linear operator(s), exponential of 97 100 Linear operator(s), invariant subspaces of 131 Linear operator(s), norm of 98 Linear operator(s), space of 98 Linear operator(s), trace of 112 Linear systems 95—210 Linear systems, differential equivalence of 143 Linear systems, linear equivalence of 142 Linear systems, singular points of, classification of 139—154 Linear systems, topological equivalence of 143—145 Linearization 95 Linearization, invariance of 95 Linearized Equation 96 Liouville's formula 114 Liouville's theorem 195 Liouville's theorem, stronger version of 198 Lipschitz condition 21 Lipschitz condition, with constant L 217 Lissajous figures 175 Local family of transformations 57 Local phase flow 51 Loesch, F. 193n Logarithmic spiral 126 Lyapunov function 146 Lyapunov function, construction of 147 Lyapunov stability 155 201n Manifold structure see "Differentiable structure" Map(s) 234 Map(s), compatible 235 Map(s), image on a 234 Mathieu's equation 193 203n Matrix of a linear system 97 Meiman, N.N. 160n Method of complex amplitudes 182 Method of Euler lines 109 Method of small parameters 64 Metric 98n Metric space 98n Metric space, complete 98n Metric space, convergence in 98n Milnor spheres 242 Moebius strip 246 Morse's lemma 85 Mulchenko, Z.M. 16n Nalimov, V.V. 16n Natural frequencies 175 Newton's equation(s) 63 80 Newton's equation(s), extension of solutions of 86 Nilpotent operator 102 Nilpotent operator, exponential of 103
Реклама
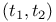-advance mapping 
|
|
О проекте