|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Barut A.O., Raczka R. — Theory of Group Representations and Applications |
|
|
 |
| Предметный указатель |
Peter, F. 71 172 175 426
Petiau, G. 631 635
Pirron, C. 418
Plancherel equality 421 422 427 430 431 432
Plancherel measure 421 422 427 435—436
Plancherel, M. 422 427 435 436
Poincare algebra 43—46
Poincare algebra, contraction of 45—46
Poincare group 515—516
Poincare group two-dimensional 476 548
Poincare group, classification of orbits 516—517
Poincare group, elementary particle 525
Poincare group, extended, representations of 525—527
Poincare group, extended, representations of, parity operator 527
Poincare group, extended, representations of, time reflection 525—526
Poincare group, extensions of 620—622
Poincare group, generalized 431
Poincare group, imaginary mass representations 632
Poincare group, invariant operators of 439—440
Poincare group, irreducible unitary representations 517—531 543
Poincare group, irreducible unitary representations, classification of 517—521
Poincare group, irreducible unitary representations, explicit realization of 522—524
Poincare group, irreducible unitary representations, indecomposable 527—531
Poincare group, irreducible unitary representations, indecomposable, applications of 531—536
Poincare group, irreducible unitary representations, SLS induced 529—531
Poincare — Birkhoff — Witt basis 250
Poincare, H. 43 45 97 250 513 514 525 527 620
Polyvector 292
Polyvector representations of GL(n) 295—296
Polyvector representations of SO(n,C) 299
Pontriagin duality theorem 163
Pontriagin, L.S. 70 163 574
Popov, V.S. 260 273
Poulsen, N.S. 501
Pozzi, G.A. 145
Principle of uniform boundness 637
Probability conserving transformation in H space 398
Proca, A. 602 603 608 635
Projection operators, generalized 459
Projective representations 400 401
Projective representations, central extension 401—402
Projective transformations 401
Pukanszky, L. 552
Pure state 395
Racah, G. 253 255 263 274
Raczka, R. 313 314 371 375 437 445 453 455 470 471 472 536 577 579
Rademacher, H. 187
Radical of a Lie group 225
Radon — Nikodym derivative 130 475
Radon — Nikodym theorem 639
Radon, I. 130 562 563 639
Raikov, D.A. 154
Rarita, W. 604 605 635
Rashid, M. 470 579
Ray representations of a group G 400
Reciprocity theorem 549
Reciprocity theorem of Mackey 550
Reciprocity theorem, generalized 552
Regge, T. 609
Relativistic position operator 581 584—588 591—593
Relativity principle 394
Rellich — Hilbert — Schmidt theorem 655
Rellich, F. 655
Representations of group(s) equivalent in H space 138
Representations of group(s) equivalent in H space, unitarily 140—141
Representations of group(s) multiplier 484—485
Representations of group(s) of a compact group 167
Representations of group(s) of a compact group, Peter — Weyl theorem 172
Representations of group(s) of a compact group, regular decomposition of 175
Representations of group(s) of a compact group, unitary 167 169 170
Representations of group(s) of a compact group, unitary, irreducible 168 170 171
Representations of group(s) of a compact group, Weyl approximation theorem 176
Representations of group(s) of a compact group, Weyl operator 167
Representations of group(s) of finite group 191—193
Representations of group(s) of finite group 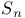 193—199 193—199
Representations of group(s) of semisimple Lie groups 200—205
Representations of group(s) of solvable groups 200—202
Representations of group(s) SLS 528—529
Representations of group(s), bounded 135
Representations of group(s), carrier space of 135
Representations of group(s), character of 192 236
Representations of group(s), character of, compound 192
Representations of group(s), character of, primitive 192
Representations of group(s), conjugate 139
Representations of group(s), conjugate, contragradient 139
Representations of group(s), continuous, strongly 134
Representations of group(s), continuous, unitary 157
Representations of group(s), continuous, weakly 137
Representations of group(s), contragradient 139
Representations of group(s), cyclic 145
Representations of group(s), decomposition of the tensor product of 235
Representations of group(s), dense set of analytic vectors for 364
Representations of group(s), direct integral of 151 152
Representations of group(s), direct sum of 142 150
Representations of group(s), discrete irreducible 577
Representations of group(s), discretely decomposable 143—144
Representations of group(s), factor 145
Representations of group(s), factor, decomposition of 181—182
Representations of group(s), factor, type I, II, III 158
Representations of group(s), faithful 138 203
Representations of group(s), finite-dimensional 202
Representations of group(s), full reducibility of 204 227
Representations of group(s), fundamental 223 295
Representations of group(s), Gel'fand — Raikov theorem 153
Representations of group(s), general properties of the Lie group representations 199—205
Representations of group(s), indecomposable 143—144 168
Representations of group(s), induced 205—213 473—476
Representations of group(s), invariant subspace of 141
Representations of group(s), irreducible 141 146 200 225—227
Representations of group(s), irreducible, algebraically 141
Representations of group(s), irreducible, projection operators 177—180
Representations of group(s), irreducible, topologically 141
Representations of group(s), Mautner theorem 153
Representations of group(s), measurable 156
Representations of group(s), multiplicity free 145 155
Representations of group(s), operator irreducibility of 144
Representations of group(s), primary 144
Representations of group(s), quasiregular 137
Representations of group(s), reducible 141 145
Representations of group(s), reducible, completely 143
Representations of group(s), reducible, decomposition of 151
Representations of group(s), reduction to a subgroup 228
Representations of group(s), regular 137 205
Representations of group(s), regular vector of, in H space 322
Representations of group(s), regular, left (right) 136
Representations of group(s), Schur's lemma 143—144 253
Representations of group(s), tensor product of 232—238
Representations of group(s), trivial 136
Representations of group(s), unitary 135—136 143 146 156—157 167 169 170—171
Rerich, K.V. 370
Richard, J.L. 636
Rideau, G. 437
Riemann, B. 646
Riesz, M. 67 640
Robinson, G.B. 192
Romm, B.D. 436
Ross, D.W. 67 71 156 163 438
Rotational invariance and superselection rules 405
Rotelli, P. 196
Ruehl, W. 437
S-theorem 253 263
Sachs, R.G. 525
Salam, A. 635
Savelev, M.V. 436 577
Schmidt, E. 169 430 655
Schouten, J.A. 94
Schrader, R. 636
Schroedinger, E. 417 418 581 588 591
Schur's lemma 143—144 253
Schur, I. 143 144
| Schwartz space of 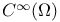 functions 319 346 functions 319 346
Schwartz, J.T. 34 36 47 48 638 644
Schwartz, L. 364
Schwinger, J. 314 604 605 635
Scull, S.C. 579
Segal, I.E. 157 275 314 372 435 436
Semidirect product of groups 96 503 622
Semidirect product of groups of type I 536
Semigroup 637
Sesquilinear form 394 395 396
Sesquilinear system (SLS) 528
Set, closed 55
Set, closure of 56
Set, complement of 55
Set, dense, in X 56
Set, open 52 54
Sharp, D. 274
Shelepin, L.A. 236
Simms, D. 402 553 625
Simon, J. 317 352 357 358 372 373
SL(2,C) group 514—515 564 567
SL(n,C) group 559—560
Smirnov, V.I. 645
SNAG theorem on abelian group representations 160 163 589
Snellman, H. 317 352 358 373
Space, globally symmetric Riemannian 126 177
Space, Hausdorff 55—58
Space, symmetric homogeneous 124 126
Space, tangent 77 78
Space, topological 52
Space, topological, homogeneous 63 123
Space, topological, separable 56
Space-time and internal symmetries 626—630
Spectral analysis 438
Spectral synthesis formula 420 422 426 430—432 438 659
Spectral theorem of von Neumann 658
Spectral theorems 649—661
Spectrum of generator of a noncompact group 577
Speiser, A. 236
Spinor of the first kind 223
Spinor of the second kind 223
Spinorial wave function 598
Stability (stationary, isotropy, little) group 473
Stein, E.M. 577
Steinberg, R. 235
Sternheimer, D. 317 352 358 373 629
Stinespring, W.F. 322 324 372 434
Stone's theorem on representations of the additive vector group 162
Stone, M.H. 71 160 162 163 274 591 643
Straumann, N. 236
Subalgebra 1
Subgroups of a topological group 61
Subgroups of a topological group, central 65
Subgroups of a topological group, invariant 62
Superposition principle 394
Superposition principle in quantum theory 395
Superselection rules 396 404—405 415
Suttorp, L.G. 196
Symmetric group SN 188
Symmetric space 124 126—127
Symmetric space and representation theory 302
Symmetric space associated with groups 447
Symmetries of physical systems 406—412
Symmetries of physical systems, dynamical 412—417
Symmetry algebra 393 409—410
Symmetry groups 409
Symmetry transformations 399 403 410
Talman, J. 313 437
Thirring, W. 197
Thrall, R.M. 192
Titchmarsh, E.C. 457
Todorov, I.T. 371
Topological manifold 75
Topological mapping 53
Topological space 52
Topological space, compact 58
Topological space, compact, locally 58 59
Topological space, connected 59
Topological space, connected, n 61
Topological space, connected, simply 60
Topological space, hausdorff 55 58 75
Topological space, separable 56
Topological space, vector 53
topology 52
Topology in metric space 54
Topology, coarsest 53
Topology, discrete 52
Topology, induced 56
Topology, natural 53
Topology, product 56
Topology, quotient 57
Topology, strong 54
Topology, uniform 72
Topology, weak 55
Tranposition 189
Uhlhorn, U. 418
Umezawa, H. 635
Unit ray 395
Universal enveloping algebra 249 250 251 258 324
Universal enveloping algebra center of 250 258
Universal enveloping algebra, element symmetric in 324
Universal enveloping algebra, elliptic element of 324
van Dam, H. 274
van Dantzig, M.D. 71
Van der Waerden, B.L. 46 197
Vector field, analytic 79
Vector field, measurable 655—656
Vector field, product of two vector fields 79—80
Vector, analytic, for a Lie group representation 322
Vector, analytic, for an operator in H space 331
Vector, cyclic 145
Vector, regular, for a Lie group representation 322
Vector, tangent 77 86
Vilenkin, N.Ya. 313 437 470 577 619
von Neumann algebra 658
von Neumann, J. 71 323 395 417 591 649 658
Wallach, N. 557 577
Warner, G. 577
Wave equation(s) 597—598
Wave equation(s) for massive particle of spin two 603—604
Wave equation(s) for massive particle with arbitrary spin 604—605
Wave equation(s) for massless particles 605
Wave equation(s), Bargmann — Wignei 605
Wave equation(s), Bhabba 631—632
Wave equation(s), Dirac 601—602
Wave equation(s), dynamical group interpretation 616
Wave equation(s), Fierz — Pauli 630
Wave equation(s), infinite component 609—616
Wave equation(s), infinite component, Gel'fand — Yaglom 609—611
Wave equation(s), infinite component, Majorana 611—613
Wave equation(s), Kemmer — Duffin — Petiau 631
Wave equation(s), Proca 602—603
Wave equation(s), Rarita — Schwinger 604
Wave equation(s), Weyl (for neutrino) 608
Weierstrass, C. 57 58
Weight algebra 49
Weight vectors 229 253
Weight, component of 229
Weight, diagrams 229—231
Weight, fundamental 224 295
Weight, highest 224 228 230 253 278
Weil, A. 71 160
Weinberg, S. 635
Weisskopf, Y. 534
Weyl form of the Heisenberg commutation relations 588—589
Weyl group 105 111 231 253
Weyl unitary trick 204
Weyl, H. 3 23 46 51 71 167 172 175 204 231 236 237 238 292 313 426 581 588 634
Whippman, M.L. 229
Wick, G.C. 418
|
|
 |
| Реклама |
 |
|
|
 |
 |
|
 |
|
О проекте
|
|
О проекте



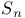
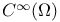 functions
functions