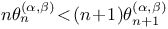P. Leopardi and the author recently investigated, among other things, the validity of the inequality between the largest zero 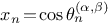and 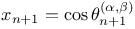of the Jacobi polynomial resp. , α > − 1, β > − 1. The domain in the parameter space (α, β) in which the inequality holds for all n ≥ 1, conjectured by us, is shown here to require a small adjustment—the deletion of a very narrow lens-shaped region in the square { − 1 < α < − 1/2,  − 1/2 < β < 0}.