Авторизация
Поиск по указателям
Milnor J., Husemoller D. — Symmetric Bilinear Forms
Обсудите книгу на научном форуме Нашли опечатку?
Название: Symmetric Bilinear FormsАвторы: Milnor J., Husemoller D. Аннотация: The theory of quadratic forms and the intimately related theory of symmetric bilinear forms have a long and rich history, highlighted by the work of Legendre, Gauss, Minkowski, and Hasse. (Compare [Dickson] and [Bourbaki, 24, p. 185].) Our exposition will concentrate on the relatively recent developments which begin with and are inspired by Witt's 1937 paper "Theorie der quadratischen Formen in beliebigen Korpern." We will be particularly interested in the work of A. Pfister and M. Knebusch. However, some older material will be described, particularly in ChapterII. The presentation is based on lectures by Milnor at the Institute for Advanced Study, and at Haverford College under the Phillips Lecture Program, during the Fall of 1970, as well as lectures at Princeton University in 1966
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1973Количество страниц: 146Добавлена в каталог: 18.11.2009Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
3 28 31 139 46 49 5 4 27—29 31 35 44 47 103 139 3 4 20 15 42 43 63 64 16 31 Anisotropic space 56 112 Arason, J.K. 76 Arf, Cahit 112 Artin — Schreier theorem 60 Asymptotic estimates 17 31 35 50 Bachet de Meziriac 40 Bilinear form 1 Bilinear form module or space 2 Blichfeldt, H.F. 29 35 Braun, Hel 127 130 Characteristic two 56 82 100 112 Convex set 16 Convolution 55 Conway, J.H. 28 46 Cyclic Witt groups 23 66 69 87 99 Dedekind domains 7 91 93 Density 42 Density of packing 34 Determinant 4 12 16 115 119 Dirichlet, P.G.L. 98 129 Discrete valuation 84 Discriminant d of a Witt class 77 Discriminant of a field extension 107 Dual basis 4 Dual lattice 25 26 48 91 127 Dyadic prime 94 Dynkin diagram 139 Eichler, Martin 27 Eisenstein, Gotthold 18 45 Euler, Leonhard 39 Exterior power 12 107 Extreme lattice or matrix 29 38 Face centered cubic lattice 30 31 35 Fermat, Pierre de 39 Finite field 21 81 87 117 Fourier series 129 Fundamental domain 15 Fundamental ideal I 66 Gauss sums 51 127 Gaussian integers 39 95 Genus of symmetric bilinear form 43 Global field 120 Hasse invariant H 79 Hasse norm theorem 121 Hasse — Minkowski theorem 20 89 120 Hasse — Witt invariant h 80 Hermite, Charles 18 Hermitian form 114 Hilbert symbol 78 134 Hlawka, E. 32 46 Hyperbolic plane 9 12—13 101 I (fundamental ideal) 66 Ideal class group 93 95 Idele group 124 Indecomposable lattice 27 Indefinite bilinear form 21 Index of isotropy 57 Inertia theorem 23 61 Inner product 1 Inner product module or space 2 Integers 15 90 Integers of number field 94 107 Intersection number 100 Involution 114 Jacob son, Nathan 115 118 Jacobi, C.G.J. 23 45 61 Klein bottle 101 Knebusch, Manfred 12 14 85 95 127 131 Kneser, Martin 28 Korkine, Alexander ( = Korkin, Aleksandr) 28 29 38146 Lagrange, Joseph Louis 40 Lattice 15 91 Leech, John 28 35 135 Legendre symbol 43 51 132 Leicht, J. 65 Level s of a field 75 Local ring 6—8 14 68 86 Lorenz, Falko 65 Lusztig, g. 106 Mass (of genus) 49 Mathieu group 137 Meyer, Arnold 20 Milgram, James 25 127 Minimal vector 27 Minkowski, Hermann 16 20 31 41 Multiplicative inner product space 72 Niemeier, H.-V. 138 Nilpotent elements, nilradical 68 69 76 Norm (in various senses) 23 72 115 121 Number field 61 64 81 94 107 118 Ordering of field 59 67 Orientation 94 101 103 Orthogonal 2 Orthogonal sum, complement, basis 4—6 p-adic integers 21 25 42 p-adic numbers 20 81 89 Packing of euclidean space 34 Pfaffian 7 Pfister, A. 65 72 76 Positive definite 16 26 61 Projective module 2 Projective plane 101 103 Pythagorean field 71 Quadratic form 22 110 Quadratic inner product space 111 Quadratic reciprocity 132—134 Quaternion algebra 118 Radical 68 69 95 Radon — Nikodym theorem 42 Rank 3 11 Rational numbers 20 87 Real fields, real closure 60 71 Reflection 7 138 Regular hexagonal lattice 30 35 Represent 120 124 Residue class field 84 Residue class form 85 Rogers, C.A. 31 35 Root systems 31 138 Scharlau, Winfried 71 133 Self—dual lattice 46 91 Shoe box principle 21 Siegel, Carl Ludwig 44—49 Signature 23—25 62—64 69 127 Similarity transformation 29 76 Simple finite groups 28 137 Skew bilinear form 2 Smith, H.J.S. 28 41 Split inner product space 12 Springer, T.A. 85 Square theorem 124 Steinberg, Robert 47 78 81 Steinitz, Ernst 7 107 Stirling's formula 31 Sums of squares 39 41 45 74 Sylvester, J.J. 23 61 Symbol 78 Symmetric bilinear form 2 Symplectic basis 7 106 Symplectic bilinear form 2 7 105 Tensor product 10 47 73 111 Thompson, John 46 Thue, A. 35 Torsion in Witt ring 68 69 72 Torus 16 101 Totally positive 61 95 Totally real, imaginary 95 Type I, type II 22—25 Unimodular lattice 16 Valuation, valuation ring 84 van der Blij, F. 24 25 Vector bundles 105—107 Volume 15 34 42 Voronoi polyhedron 30 Weak approximation theorem 123 Weil, Andre 131—134 Witt ring of 23 90 Witt ring, Witt class 14 112 114 Witt rings of special fields 81 87 88 Witt, Ernst 8 28 84 137 Zolotareff, G. (= Zolotarev, E.I.) 28 38
Реклама
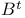(transpose of matrix) 
(root systems) 
(genus of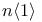 
) 
(orthogonal complement)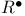 
(units of ring) 
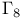(lattice) 
, 
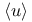(inner product space specified by matrix)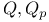 
, 
, 
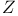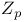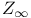
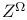
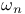(volume) 
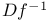of solutions 
|
|
О проекте