Авторизация
Поиск по указателям
Protter P.E. — Stochastic Integration and Differential Equations
Обсудите книгу на научном форуме Нашли опечатку?
Название: Stochastic Integration and Differential EquationsАвтор: Protter P.E. Аннотация: It has been 15 years since the first edition of Stochastic Integration and Differential Equations, A New Approach appeared, and in those years many other texts on the same subject have been published, often with connections to applications, especially mathematical finance. Yet in spite of the apparent simplicity of approach, none of these books has used the functional analytic method of presenting semimartingales and stochastic integration. Thus a 2nd edition seems worthwhile and timely, though it is no longer appropriate to call it "a new approach". The new edition has several significant changes, most prominently the addition of exercises for solution. These are intended to supplement the text, but lemmas needed in a proof are never relegated to the exercises. Many of the exercises have been tested by graduate students at Purdue and Cornell Universities. Chapter 3 has been completely redone, with a new, more intuitive and simultaneously elementary proof of the fundamental Doob-Meyer decomposition theorem, the more general version of the Girsanov theorem due to Lenglart, the Kazamaki-Novikov criteria for exponential local martingales to be martingales, and a modern treatment of compensators. Chapter 4 treats sigma martingales (important in finance theory) and gives a more comprehensive treatment of martingale representation, including both the Jacod-Yor theory and Emery’s examples of martingales that actually have martingale representation (thus going beyond the standard cases of Brownian motion and the compensated Poisson process). New topics added include an introduction to the theory of the expansion of filtrations, a treatment of the Fefferman martingale inequality, and that the dual space of the martingale space H^1 can be identified with BMO martingales. Solutions to selected exercises are available at the web site of the author, with current URL http://www.orie.cornell.edu/~protter/books.html.
Язык: Рубрика: Математика /Вероятность /Стохастические процессы /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Издание: второеГод издания: 2004Количество страниц: 430Добавлена в каталог: 07.06.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
248 369 369 154 193 247 260 245 247 260 244 Absolutely continuous 131 Absolutely Continuous Compensators Theorem 192 Absolutely continuous space 191 Accessible stopping time 103 Adapted process with bounded caglad paths 56 Adapted process with cadlag paths 56 Adapted process with caglad paths 56 Adapted process, decomposable 55 Adapted process, definition of 3 Adapted process, space 155 Angle bracket 123 Announcing sequence for stopping time 103 Approximation of the compensator by Laplacians 150 Arcsine law 228 Associated jump process 27 Asymptotic martingale (AMART) 218 Autonomous 250 293 Azema's martingale 204 Azema's martingale and last exit from zero 228 Azema's martingale, definition of 228 Azema's martingale, local time of 230 Backwards Convergence Theorem 9 Banach — Steinhaus theorem 43 Barlow's Theorem 240 Bichteler — Dellacherie Theorem 144 BMO see bounded mean oscillation Bouleau — Yor formula 226 227 Bounded functional Lipschitz 257 Bounded jumps 25 Bounded mean oscillation, BMO norm 195 Bounded mean oscillation, BMO space of martingales 195 Bounded mean oscillation, Duality Theorem 199 Bracket process 66 Brownian bridge 97 299 384 Brownian motion, absolute value of 217 Brownian motion, arcsine law for last exit from zero 228 Brownian motion, Azema's martingale 228 Brownian motion, Brownian bridge see Brownian bridge Brownian motion, completed natural filtration right continuous 19 Brownian motion, conditional quadratic variation of 123 Brownian motion, continuous modification 17 Brownian motion, continuous paths 221 Brownian motion, definition of 16 Brownian motion, Fisk — Stratonovich exponential of 280 Brownian motion, Fisk — Stratonovich integral for 288 Brownian motion, geometric 86 Brownian motion, Girsanov — Meyer Theorem and 141 Brownian motion, Ito integral for 78 Brownian motion, last exit from zero 228 Brownian motion, last exit time 46 Brownian motion, Levy's characterization of 88 Brownian motion, Levy's Theorem (martingale characterization) 86 87 Brownian motion, local time of 217 225 Brownian motion, martingale characterization (Levy's Theorem) 86 87 Brownian motion, martingale representation for 187 Brownian motion, martingales as time change of 88 Brownian motion, maximum process 23 Brownian motion, on the sphere 281 Brownian motion, Ornstein — Uhlenbeck 298 Brownian motion, quadratic variation of 17 67 123 Brownian motion, reflection principle 23 228 Brownian motion, reversibility for integrals 380 Brownian motion, semimartingale 55 Brownian motion, standard 17 Brownian motion, starting at x 17 Brownian motion, stochastic area formula 89 Brownian motion, stochastic exponential for see geometric Brownian motion Brownian motion, stochastic integral exists 173 Brownian motion, strong Markov property for 23 Brownian motion, Tanaka's formula 217 Brownian motion, tied down or pinned see Brownian bridge Brownian motion, time change of 88 Brownian motion, unbounded variation 19 Brownian motion, white noise 141 243 Burkholder — Davis — Gundy inequalities 193 Burkholder's inequality 222 Cadlag 4 Caglad 4 Canonical decomposition 129 154 Censored data 121 Centering function 32 Change of time 88 190 Change of time, exercises in Chap. II 98 Change of time, Lebesgue's formula 190 Change of variables see Ito's formula see Change of Variables Theorem for continuous FV processes 41 Change of Variables Theorem for right continuous FV processes 78 Class D 106 Classical semimartingale 102 127 144 Closed martingale 8 Comparison theorem 324 Compensated Poisson process 31 42 Compensator 118 Compensator of 371 Compensator, Absolutely Continuous Compensators Theorem 192 Compensator, Knight's compensator calculation method 151 Compound Poisson process 33 Conditional quadratic variation 70 Conditional quadratic variation, Brownian motion 123 Conditional quadratic variation, polarization identity 123 Continuous local martingale part of semimartingale 70 221 Continuous martingale part 191 Converge (pre) locally in 260 Convex functions and Meyer-Ito formula 214 Convex functions of semimartingale 210 Countably-valued random variables corollary 365 Counting process 12 Counting process, explosion time 13 Counting process, without explosions 13 Crude hazard rate 122 Decomposable adapted process 55 101 Diffeomorphism of Rn 319 Diffusion 243 291 297 Diffusion coefficient 298 Diffusion examples 298 Diffusion, rotation invariant 282 Discrete Laplacian approximations 150 Doleans — Dade exponential see stochastic exponential Dominated Convergence Theorem for stochastic integrals in 267 Dominated Convergence Theorem for stochastic integrals in ucp 174 Doob class see Class D Doob decomposition 105 Doob — Meyer Decomposition Theorem, case without Class D 115 Doob — Meyer Decomposition Theorem, general case 111 Doob — Meyer Decomposition Theorem, totally inaccessible jumps 106 Doob's maximal quadratic inequality 11 Doob's Optional Sampling Theorem 9 Drift coefficient 143 298 Drift coefficient, removal of 137 Duality theorem 199 Dynkin's expectation formula 350 Dynkin's formula 56 350 Einstein convention 305 Emery — Perkins Theorem 240 Emery's example of stochastic integral behaving badly 176 Emery's inequality 246 Emery's structure equation see structure equation Enveloping sequence for stopping time 103 Equivalent probability measures 131 Euler method of approximation 353 Evanescent 158 Example of local martingale that is not martingale 37 74 Example of process that is not semimartingale 217 Example of stochastic integral that is not local martingale 176 Example, Emery's example of stochastic integral behaving badly 176 Example, expansion via end of SDE 367 Example, Gaussian expansions 366 Example, hazard rates and censored data 121 Example, Ito's example 366 Example, reversibility for Brownian integrals 380 Example, reversibility for Levy process integrals 380 Examples of diffusions 298 Existence of Solutions of Structure Equation Theorem 201 Expanded filtration 370 Expansion by a natural exponential r.v. 386 Expansion via end of SDE 367 Explosion time 13 254 303 Extended Gronwall's inequality 352 Extremal point 183 F-S see Fisk-Stratonovich Fefferman's inequality 195 Fefferman's inequality, strengthened 195 Feller process 35 Filtration shrinkage 367 Filtration Shrinkage Theorem 369 Filtration, countably-valued random variables corollary 365 Filtration, definition of 3 Filtration, expansion by a natural exponential r.v. 386 Filtration, filtration shrinkage 367 Filtration, Filtration Shrinkage Theorem 369 Filtration, Gaussian expansions 366 Filtration, independence corollary 365 Filtration, Ito's example 366 Filtration, natural 16 Filtration, progressive expansion 355 369 Filtration, quasi left continuous 148 189 Filtration, right continuous 3 Finite quadratic variation 271 Finite variation process, definition of 39 101 Finite variation process, integrable variation 111 Fisk — Stratonovich, acceptable 278 Fisk — Stratonovich, approximation as limit of sums 284 Fisk — Stratonovich, integral 82 271 Fisk — Stratonovich, integral for Brownian motion 288 Fisk — Stratonovich, Integration by Parts Theorem 278 Fisk — Stratonovich, Ito's circle 82 Fisk — Stratonovich, Ito's formula 277 Fisk — Stratonovich, stochastic exponential 280 Flow of SDE 301 Flow of solution of SDE 382 Flow, strongly injective 311 Flow, weakly injective 311 Fubini's Theorem for stochastic integrals 207 208 Function space 301 Functional Lipschitz 250 Fundamental L martingale 372 Fundamental sequence 37 Fundamental Theorem of Local Martingales 125 FV see finite variation process Gamma process 33 Gaussian expansions 366 Generalized Ito's formula 271 Generator of stable subspace 179 Geometric Brownian motion 86 Girsanov — Meyer Theorem 132 Girsanov — Meyer Theorem, predictable version 133 Graph of stopping time 104 Gronwall's inequality 342 352 Gronwall's inequality, extended 352 Hadamard's Theorem 330 Hahn — Banach theorem 199 Hazard rates 121 Hitting time 4 Holder continuous 99 Honest random variable 373 Hunt process 36 Hypothesis A 221 Increasing process 39 Independence corollary 365 Independent increments of Levy process 20 Independent increments of Poisson process 13 Index of stable law 34 Indistinguishable 3 Infinitesimal generator 349 Injective flow see strongly injective flow Integrable variation process 111 Integration by Parts Theorem for Fisk-Stratonovich integrals 278 Integration by Parts Theorem for semimartingales 68 83 Intrinsic Levy process 20 Ito integral see stochastic integral Ito — Meyer formula see Meyer — Ito formula Ito's circle 82 Ito's example 366 Ito's formula for an n-tuple of semimartingales 81 Ito's formula for complex semimartingales 83 84 Ito's formula for continuous semimartingales 81 Ito's formula for Fisk — Stratonovich integrals 277 Ito's formula for semimartingales 78 Ito's formula, generalized 271 Ito's Theorem for Levy processes 356 Jacod — Yor Theorem on martingale representation 199 Jacod's Countable Expansion 366 Jacod's Countable Expansion Theorem 53 356 Jacod's criterion 363 Jensen's inequality 11 Jeulin's Lemma 360 Kazamaki's criterion 139 Knight's compensator calculation method 151 Kolmogorov's continuity criterion 220 Kolmogorov's Lemma 218 Kronecker's delta 305 Kunita — Watanabe inequality 69 Last exit from zero 228 Le Jan's Theorem 124 Lebesgue's change of time formula 190 Lenglart — Girsanov Theorem 135 Lenglart's Inclusion Theorem 177 Levy Decomposition Theorem 31 Levy measure 26 Levy process is a semimartingale 55 Levy process, associated jump process 27 Levy process, bounded jumps 25 Levy process, cadlag version 25 Levy process, centering function 32 Levy process, definition of 20 Levy process, has finite moments of all orders 25 Levy process, intrinsic 20 Levy process, Ito's Theorem for 356 Levy process, Levy measure 26 Levy process, reflection principle 49 Levy process, reversibility for integrals 380 Levy process, strong Markov property for 23 Levy process, symmetric 49 Levy — Khintchine formula 31 Levy's arcsine law 228 Levy's stochastic area formula 89 Levy's stochastic area process 89 Levy's Theorem characterizing Brownian motion 86 87 Linkage operators 329 Lipschitz process 250 311 Lipschitz, bounded functional 257 Lipschitz, definition of 250 293 Lipschitz, functional 250 Lipschitz, locally 251 254 303 Lipschitz, random 250 Local behavior of stochastic integral 62 165 170 Local behavior of the stochastic integral at random times 375 Local convergence in 260 Local martingale, BMO 195
Реклама
 |
|
О проекте
|
|
О проекте



 -sliceable
-sliceable 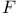 optional projection
optional projection  semimartingale
semimartingale  norm
norm 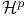 norm
norm 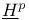 , (pre)locally in
, (pre)locally in 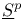 , (pre) locally in
, (pre) locally in 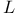 of with caglad paths
of with caglad paths 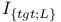 for F
for F