Let
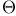
be a smooth compact oriented manifold without boundary, imbedded in a Euclidean space
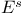
, and let

be a smooth map of

into a Riemannian manifold Λ. An unknown state

is observed via X =

, where

> 0 is a small parameter and
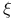
is a white Gaussian noise. For a given smooth prior

on

and smooth estimators

of the map

we derive a secondorder asymptotic expansion for the related Bayesian risk. The calculation involves the geometry of
the underlying spaces

and

, in particular, the integration-by-parts formula. Using this result, a second-order minimax estimator of

is found based on the modern theory of harmonic maps and hypo-elliptic differential operators.
 |
|
О проекте
|
|
О проекте


 be a smooth compact oriented manifold without boundary, imbedded in a Euclidean space
be a smooth compact oriented manifold without boundary, imbedded in a Euclidean space 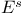 , and let
, and let  be a smooth map of
be a smooth map of  into a Riemannian manifold Λ. An unknown state
into a Riemannian manifold Λ. An unknown state  is observed via X =
is observed via X =  , where
, where  > 0 is a small parameter and
> 0 is a small parameter and 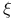 is a white Gaussian noise. For a given smooth prior
is a white Gaussian noise. For a given smooth prior  on
on  of the map
of the map  , in particular, the integration-by-parts formula. Using this result, a second-order minimax estimator of
, in particular, the integration-by-parts formula. Using this result, a second-order minimax estimator of 