Borwein P. — Computational Excursions in Analysis and Number Theory :: Электронная библиотека попечительского совета мехмата МГУ

Главная Ex Libris Книги Журналы Статьи Серии Каталог Wanted Загрузка ХудЛит Справка Поиск по индексам Поиск Форум

Авторизация

Поиск по указателям

Borwein P. — Computational Excursions in Analysis and Number Theory

Обсудите книгу на научном форуме

Нашли опечатку?
Выделите ее мышкой и нажмите Ctrl+Enter

Название: Computational Excursions in Analysis and Number Theory

Автор: Borwein P.

Аннотация:

This book is designed for a computationally intensive graduate course based around a collection of classical unsolved extremal problems for polynomials. These problems, all of which lend themselves to extensive computational exploration, live at the interface of analysis, combinatorics and number theory so the techniques involved are diverse.A main computational tool used is the LLL algorithm for finding small vectors in a lattice.
Many exercises and open research problems are included. Indeed one aim of the book is to tempt the able reader into the rich possibilities for research in this area.

Язык:

Рубрика: Математика/Теория чисел/Вычислительная теория чисел/

Статус предметного указателя: Готов указатель с номерами страниц

ed2k: ed2k stats

Год издания: 2002

Количество страниц: 232

Добавлена в каталог: 01.06.2005

Операции: Положить на полку | Скопировать ссылку для форума | Скопировать ID

Предметный указатель

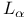$L_{\alpha}$       3
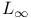$L_{\infty}$       3
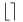$\lfloor\rceil$       156
$\mathbb{Z}[z]$       2
$\mathbb{Z}_p[z]$       2
$\mathcal{A}$       2
$\mathcal{A}_n$       2
$\mathcal{L}$       2
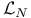$\mathcal{L}_N$       2
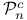$\mathcal{P}^c_n$       2
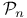$\mathcal{P}_n$       2
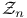$\mathcal{Z}$ 2 $\mathcal{Z}_n$       1
Acyclic autocorrelation      6 109
Algebraic integer      15 80
Approximating zeros      67
Atkinson, F.      104
Autocorrelation coefficient      109 115
Average norms of polynomials      32
Barker polynomial      6 34 109 128 196
Basic PSLQ algorithm      173
Beck, J.      127
Bernasconi Model      122
Bernstein-type inequality for rational functions      146
Bernstein-type inequality in 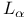$L_{\alpha}$       142
Bernstein’s inequality for trigonometric polynomials      142
Bernstein’s inequality on the disk      142
Bombieri’s norm      151
Boyd, D.      17 20 60 64
Boyd’s algorithm      25
Boyd’s conjecture      20
Byrnes, J.      66
Cauchy’s integral formula      4
CHARACTER      182
Character polynomial      182
Character, primitive character      182
Chebyshev polynomials      56 75
Chebyshev polynomials of the second kind      56
Chebyshev’s inequality      143
Chernick, J.      88
Choi, S.      viii
Chudnovsky, G.      79 83 200
Classes of polynomials      1
Closure of measures problem      6
Cohen, H.      154
Conjecture of Boyd      20
Conjecture of Erdos      127
Conjecture of Erdos and Szekeres      103
Conjecture of Konyagin      128
Conjecture of Littlewood      124
Conjecture of Montgomery      79
Conjecture of Schinzel and Zassenhaus      6 20 196
Conjecture of Wright      87
Conjecture on Barker polynomials      110
Conjecture on bounded merit factors      122
Conrey, B.      40 182
Cyclotomic polynomial      15 43 124
de Bruijn, N.G.      49 143
Denseness of zeros      72
Diophantine approximation      67
Dirichlet box argument      23
Distribution of zeros      54
Dobrowolski, E.      16
Easier Waring problem      95 97 201
Elliptic curve      90
Enestrom — Kakeya theorem      148
Equal sums of like powers      85
Equioscillation property      56
Erdos — Szekeres problem      5 103 195
Erdos, another problem      64
Erdos, P.      133
Erdos, problem for real trigonometric polynomials      127
Erdos, problem in 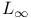$L_{\infty}$       5
Even ideal symmetric solution      86
Everest, G.      143
Expected norm      125
Explicit merit factor formulae      181
Fejer’s theorem      149
Fekete polynomial      37 123 181
Fekete polynomial, shifted      123
Fekete, M.      76
Ferguson, H.      153 157
Ferguson, R.      viii
Flat polynomials      127
Forcade, R.      153 157
Fractal set      73 199
Gauss sum      182
Gauss, K.F.      38
Ghate, E.      17
Golay and Harris heuristic      130
Golay complementary pair      31 109—111
Golay, M.      6 124 182
Golden mean      23
Golomb ruler problem      129
Gongalves’s inequality      148
Gorshkov — Wirsing polynomials      78 79
Gorshkov — Wirsing polynomials on [0, 1]      81
Graeffe’s root powering method      44
Gray codes      9
Guy, R.      64
H(p)      4
Habsieger, L.      77 79
Hadamard’s inequality      105
Hare, K.      viii
Hastad, J.      169
Height      2 3 142
Hilbert, D.      1 76
Hironaka, E.      17
HJLS      153 169 170
Holder’s Inequality      4
Ideal solution      86 97 103
Inequality for factors      150
Inequality for length and height      148
Inequality for measure      148
Inequality for reciprocal polynomials      145
Inequality for zeros      148
Inequality of Bernstein      142
Inequality of Chebyshev      143
Inequality of Holder      4
Inequality of Markov      143
Inequality, involving coefficients      147
Integer Chebyshev constant      75 76
Integer Chebyshev problem      vii 5 12 195
Integer relation      12 106
Integer relation, algorithms      153
Integer transfinite diameter      75 84
Jacobi symbol      182
Jedwab, J.      viii 119
Jensen’s theorem      3—5
Joo, I.      133
Just, B.      169
Kahane, J.-P.      127
Kapoor, V.      viii
Kashin, B.      76
Kneser’s inequality      150
Knuth, D.      9
Komornik, K.      133
Konyagin, S.      125 128
Konyagin’s conjecture      128

Kronecker’s theorem      15 43 51
L(p)      4
Lagarias, J.      169
Lattice      vii 11 77 153
Lattice, basis reduction algorithm      153
Lau, K.-S.      136
Lax-type inequality      146
Lax’s inequality      144
Legendre symbol      37 122 181
Lehmer’s conjecture      62
Lehmer’s problem      vii 6 17 196
Length      3 142
Lenstra, Lenstra, and Lovasz      11 154
Limit points of Salem numbers      22
Littlewood polynomials      2 43 121
Littlewood polynomials, random      125
Littlewood’s conjecture      124
Littlewood’s problem      5 121 126 195
Littlewood’s problem in 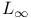$L_{\infty}$       126
LLL algorithm      12 153 156
Location of zeros      53
Lorentz, G.G.      143
Loreti, P.      135
Lucas’s theorem      150
m(P)      3
Mahler measure      3 15
Mahler’s problem      6 20 196
Maier, H.      52
Malik, M.      144
Maltby, R.      104 106
Markov’s inequality      77 143
Maximum multiplicity of the vanishing at 1      60 61
McCollum, S.      viii
Meichsner, A.      viii 153
Mercer, I.      viii
Merit factor      115 122 123 182
Merit factor problem      6 110 196
Mignotte, M.      148
Monk integer Chebyshev polynomials      82
Montgomery question      7 79 197
Montgomery, H.      38 78
Mossinghoff, M.J.      17 25
Multiplicative      3 46 51 189
Multivariate Mahler measure      17
Nazarov, M.      126
Negative reciprocal polynomial      16
NP-hard      11
Odd ideal symmetric solution      86
Odlyzko question      65
Odlyzko, A.      63 126
Open problems      195
Parseval’s formula      17
Pedicini, M.      135
Pellet’s theorem      149
Pentagonal number theorem      106
Perron number      24
Pinner, C.      25 67
Pisot number      15 133
Polynomial, average norm      32
Polynomial, Barker polynomial      6 34 109
Polynomial, Chebyshev polynomials      56 75
Polynomial, classes of polynomials      1
Polynomial, cyclotomic polynomial      15 43
Polynomial, Fekete polynomial      37 123
Polynomial, Littlewood polynomials      2 43 121
Polynomial, negative reciprocal polynomial      16
Polynomial, reciprocal polynomial      6 16
Polynomial, Rudin — Shapiro polynomials      27
Polynomial, self-inversive polynomial      16
Polynomial, shifted Fekete polynomials      40
Polynomial, skewsymmetric polynomial      32
Polynomial, symmetric polynomial      8
Polynomial, Turyn-type polynomials      123
Poonen, B.      63
Pott, A.      119
Primitive character      182
Pritsker, I.      79
Products of cyclotomic polynomials      43
Prouhet — Tarry — Escott problem      5 12 85 97 103 195
Pseudocode for HJLS      170 172
Pseudocode for LLL      156
Pseudocode for PSLQ      160
PSLQ algorithm      11 12 153 157 160
Quadratic character      37
Quadratic reciprocity theorem      41
Random Littlewood polynomial      125
Reciprocal polynomial      6 16
Reduced basis      11
Research problems      195
Rhin, G.      25 197
Riemann hypothesis is false      9
Riesz’s identity      143
Robinson, L.      126
Rouche’s Theorem      4
Rudin — Shapiro polynomials      27 37 112 122 126
Saffari, B.      116 126
Salem number      15
Salvy, B.      77 79
Schinzel, A.      16
Schinzel’s theorem      19
Schmidt, B.      116
Schnorr, C.P.      169
Schur — Siegel — Smyth trace problem      7 79 197
Schur, I.      79
Schur’s Theorem      53
Self-inversive polynomial      16
Self-inversive polynomial, Barker polynomials      116
Shapiro, H.      27 144
Shifted Fekete polynomials      40 42 123
Siegel zero      40
Skewsymmetric polynomial      32 123
Smallest Pisot number      16
Smallest Salem number      16
Smyth, C.      viii 16 79 90
Smyth’s theorem      17
Smyth’s theorem, weak form      17
Solomyak, B.      135
Spectra      133
Supremum norm      3 141
Symmetric polynomial      8
Szasz’s inequality      147
Szego’s theorem      149
Turyn, R.      116 117 122
Turyn-type polynomials      123 182 192
Vaaler, J.      25
Vandermonde determinant      105
Vaughan, R.      100
Visser’s inequality      147
von Golitschek, M.      143
Voutier, P.      16
Walsh’s two-circle theorem      150
Ward, T.      143
Waring problem      97
Waring problem, easier Waring problem      97 201
Weak form of Smyth’s theorem      17
Wooley, T.      100
Wright, E.M.      85 87 97
Zassenhaus, H.      20

О проекте