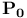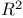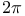 -periodic integrable function 158 -periodic integrable function 158
 -bounded 238 -bounded 238
 -martingale convergence theorem 238 -martingale convergence theorem 238
 -measurable 32 -measurable 32
 -simple function 30 -simple function 30
 -negative set 62 -negative set 62
 -positive set 62 -positive set 62
 -additive 9 -additive 9
 -additive probability on a Boolean algebra 17 -additive probability on a Boolean algebra 17
 -algebra 9 -algebra 9
 -algebra generated by -algebra generated by 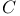 14 14
 -algebra of -algebra of 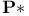 -measurable sets 24 -measurable sets 24
 -algebra of Borel subsets of -algebra of Borel subsets of  14 14
 -algebra of Borel subsets of -algebra of Borel subsets of 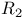 87 87
 -algebra of Borel subsets of -algebra of Borel subsets of 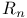 87 87
 -algebra of Borel subsets of E 131 -algebra of Borel subsets of E 131
 -algebra of Lebesgue measurable subsets of -algebra of Lebesgue measurable subsets of 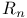 104 104
 -field 10 -field 10
 -finite measure 44 -finite measure 44
 -finite measure space 45 -finite measure space 45
 -finite signed measure 61 -finite signed measure 61
Absolutely continuous function on [a,b] 76
Absolutely continuous probability 57
Absolutely continuous with respect to  66 66
Absolutely convergent 54
Absolutely summable 146
Adapted 229
Almost isomorphic measure spaces 206
Analyst’s distribution function 200
Approximate identity 191
Approximation to the identity 191
Archimedean property 2
Arithmetic mean 142
At most countable 15
atoms 224 230
Axiom of the least upper bound 2
Backward martingale 248
Banach algebra 134
Banach space 146
Bessel’s inequality 155
Betting system 232
Bolzano — Weierstrass property 28
Boolean algebra 6
Boolean ring 45
Borel function 36
Borel — Cantelli lemma 148
Borel’s strong law of large numbers 205
Bounded interval 4
Bounded variation 71
Cantor discontinuum 8
Cantor set 8
Cantor — Lebesgue function 84
Cantor’s diagonal argument 15
Cartesian product 15
Cauchy sequence 146
Cauchy — Schwarz inequality 59 216
Central limit theorem: i.i.d. case 275
Central limit theorem: Lindebergh condition 280
Central limit theorem: triangular arrays 281
Cesaro mean 162
Characteristic function 79 263
Characteristic function of a set 30
Chebychev’s Inequality 169
Closed 14
Closed interval 4
Closed monotone class 134
Closure 15
Compact 20
Compact support 150
Compact support in an open set 150
Complete measure space 105
Complete metric space 146
Complete orthonormal system see Orthonormal system
Completing the measure see Completion
Completion 47 105
Completion of  81 81
Complex conjugate of a complex number 289
Conditional distribution function of X given Y=y 221
Conditional expectation of X 212
Conditional probability of E given A 47
Conditionally convergent 54
Conditionally independent 226
Conjugate indices 141
Continuity theorem, characteristic function 269
Continuous at 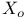 36 36
Continuous at 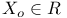 36 36
Continuous measure 83
Continuous on  36 36
Continuous on E 36
Converge in distribution 255
Converge in law 255
Converge weakly 251 255
Convergence 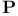 -a.e. 167 -a.e. 167
Convergence  -a.e 167 -a.e 167
Convergence in measure 168
Convergence in probability 168
Converges to 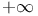 2 2
Converges to 0 in  145 145
Converges to 0 in  -norm 145 -norm 145
Converges to B 2
Converges to x 146
Converges uniformly 134
Converges weakly 251
Convex 144
Convex set 214
Convolution kernels 120
Convolution of integrable functions 157
Convolution of two probabilities 108
Countable 15
Countable additivity 9
Countably additive 9
Countably generated 248
Countably subadditive 21
Dedekind cut 2
Dense 16
Dini derivatives 194
Dirac measure at a 26
Dirac measure at the origin 26
Dirichlet’s kernel 162
Discrete distribution function 57
Discrete measure 83
Distribution function 11
Distribution of a random vector X 88
Distribution of X 37
Doob decomposition 243
Doob’s  -inequality: countable case 239 -inequality: countable case 239
Doob’s maximal inequality: finite case 237
Egororov’s theorem 168 202
Empirical distribution function 250
Equicontinuous 283
Ergodic probability space 94
Essential supremum 144
Essentially bounded 144
Excessive function 231
Expectation 30
Expectation of X 38
Exponentially distributed (with parameter  ) 56 ) 56
Fatou’s Lemma 39
Fejer kernel 160
Fejer’s theorem 163
Filtration 225 229
Finite expectation 42
Finite measure 44
Finite measure space 45
Finitely additive probability space 6
Fourier inversion theorem 271
Fourier series 154
Fourier transform 79
Fubini’s theorem for  -functions 106 -functions 106
Fubini’s theorem for integrable random variables 100
| Fubini’s theorem for Lebesgue integrable functions on  131 131
Fubini’s theorem for positive
Fubini’s theorem for positive functions 105
g.l.b. see Greatest lower bound
Gamma distribution 56
Geometric mean 142
Glivenko — Cantelli theorem 261
Greatest lower bound 2
Hahn decomposition 64
Half-open interval 4
Hardy — Littlewood maximal function 187
Harmonic function 231
Heaviside function 26
Heine — Borel theorem 19
Helly’s first selection principle 269
Hermite polynomial 80
Hilbert space 154 216
Holder’s Inequality 142
Homogeneous Markov chain 119
I.i.d. sequence 170
Image measure 138
Image of P 37
Improper Riemann integral of f over 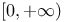 54 54
Increasing process 243
Independent collections of events 89
Independent events 89
Independent family of classes of events 132
Independent family of events or sets 111
Independent family of random variables 111
Independent, finite collection of random variables 94
Indicator function of a set 30
Inequality of Cauchy — Schwarz 139
inf see Infimum
Infimum 20
Infinite product of a sequence of probability spaces 118
Infinite product set 113
Infinite series 3
Infinitely differentiable function 79 see
Initial distribution 122
Inner product 59
Integers 1
Integrable random variable 41 see
integral 30
Integral of X 38
Integration by parts 135
Integration by parts: functions 281
Integration by parts: series 241
interval 4
Inverse of a distribution function 259
Inversion theorem, characteristic function 272
Irrational 1
Isomorphic measure spaces 206
Jensen’s Inequality 144
Jensen’s inequality, conditional expectation 219
Joint distribution, finite-dimensional 110
Jordan decomposition 65
Khintchine’s weak law of large numbers 172
Kolmogorov’s 0-1 law 111
Kolmogorov’s criterion 179
Kolmogorov’s inequality 178 237
Kolmogorov’s strong law of large numbers 177 240
Kolmogorov’s strong law: backward martingale 249
Kronecker’s lemma 241
l.u.b. see Least upper bound
Laplace transform 79
Law of a random vector X see Distribution of a random vector X
Law of X see Distribution of X
Least squares 218
Least upper bound 1
Lebesgue decomposition theorem for measures 68
Lebesgue integral 48
Lebesgue measurable sets 51
Lebesgue measure 46
Lebesgue measure on  104 104
Lebesgue measure on  104 104
Lebesgue measure on E 48
Lebesgue point 188
Lebesgue set 188
Lebesgue’s differentiation theorem 188
Left continuous 11
Left limit 11
Liminf see Limit infimum
Limit infimum 33
Limit supremum 33
Limsup see limit supremum
Lindebergh condition 280
Lipschitz truncation of a random variable at height c>0 181
Locally integrable function 189
Lower bound 1
Lower semi-continuous 191
Lower semi-continuous at 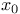 191 191
Lower sum 49
Lower variation 65
Lusin’s theorem 202
Marginal distributions 89
Markov chain 119
Markov chain with transition probability  , initial distribution , initial distribution 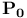 127 127
Markov process 225
Markov process with transition kernel N 225
Markov property 127 226
Markovian kernel see Transition kernel
Martingale 229
Martingale convergence theorem: countable case 246
Martingale transform 232
Maximal function see Hardy — Littlewood maximal function
Maximal function: martingale 239
Maximal function: submartingale 237
Measurable 86
Measurable function 32
Measurable space 32
Measure space 44
Measure zero 105
Metric 146
Metric space 146
Metric space E 48
Minkowski’s inequality 142
Modulus of a complex number 289
Monotone class 25
Monotone class of functions 134
Monotone class theorem for functions 133
Monotone class theorem for sets 25
Monotone class theorem, Dynkin’s version 92
Monotone rearrangement 260
Multivariate normal distribution 107
Mutually singular 65
N-dimensional random vector 88
Natural numbers 1
Nearest neighbours 120
Neighbourhood of 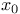 36 36
Non-increasing rearrangement 260
Non-Lebesgue measurable set 49
Non-negative integrable random variable 38
Non-negative measure 44
Norm 58
Normal number 171
Normally summable 146
Normed vector space 146
Null function 41
Null set 104 see
Null variable 41
Occurrence of a set prior to a stopping time 234
open 14
Open ball in a metric space about a of radius  146 146
Open interval 4
Open relative to E see Open subset of E
Open set in a metric space 146
Open subset of 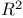 87 87
Open subset of  87 87
Open subset of E 131
Optional stopping theorem: finite case 235
|
 |
|
О проекте
|
|
О проекте



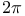 -periodic integrable function
-periodic integrable function  -bounded
-bounded  -measurable
-measurable  -negative set
-negative set  -additive
-additive 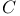
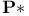 -measurable sets
-measurable sets 


 -a.e.
-a.e. 

 )
)  -functions
-functions 
 , initial distribution
, initial distribution