|
Электронная библиотека Попечительского совета механико-математического факультета Московского государственного университета |
| Главная Ex Libris Книги Журналы Статьи Серии Каталог Wanted Загрузка ХудЛит Справка Поиск по индексам Поиск |
|
© Электронная библиотека попечительского совета мехмата МГУ, 2004-2025 |
|
О проекте |
|
О проекте
|



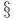 3.2
3.2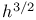 )
) 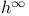 )
) 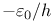 ))
))  )
)  norm
norm  dinger operator
dinger operator