|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Greene R.E., Wu H. — Function Theory on Manifolds Which Possess a Pole |
|
|
 |
| Предметный указатель |
 estimates of estimates of  145—146 145—146
Ahlfors' generalized Schwarz lemma 81 86 112 131
Bergman kernel 142 175 178
Bergman metric 3 55 133
Bergman metric in terms of special basis 153 155
Bergman metric of CH manifolds 141ff
Bounded exhaustion functions 99
Bounded exhaustion functions, existence of subharmonic 101
Canonical bundle 158
CH manifolds (Cartan — Hadamard manifolds) 1 5 20 57 111
CH manifolds (Cartan — Hadamard manifolds), Bergman metric of 141ff
CH manifolds (Cartan — Hadamard manifolds), conditions for biholomorphism to 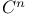 180ff 180ff
Chern — Kobayashi volume generalization of Schwarz lemma 131
Complete hyperbolic manifolds 3 111 112 113
Complex Laplacian 12
Complex manifold (notations) 8—9
Complex normal coordinates 11 155 176
Complex structure of a 2-dimensional Riemannian manifold 89 120
Complex structure of a 2-dimensional Riemannian manifold, Milnor's theorem on 89 120
Complex structure of a 2-dimensional Riemannian manifold, Yang's theorem on 120
Complex structure tensor 10
Connection, Hermitian 11
Connection, Levi-Civita 11
Convex function 7 14
Convex function, strictly convex function 14
Curvature 5
Curvature of a Hermitian metric 85—86
Curvature of a pseudo-Hermitian metric 85
Curvature, holomorphic sectional 5 112 113 128
Curvature, Ricci 5 129
Divergence form (of a second order elliptic operator) 78
Domination by a weal model 43
Eberlein — O'Neill boundary 176
Elat metric 123
Exhaustion function 14
Exhaustion function, bounded 99
Exhaustion function, bounded subharmonic 101
Exponential mapping 1
Gauss' lemma 22
Geodesic polar coordinates 23 31
Green's identity 44
Harmonic functions 2—3 15
Harmonic functions,  norm of 47 norm of 47
Harmonic functions, existence of bounded 116
Harmonic functions, mean value theorem for 48
Harnack inequality, Moser's 79
Hermitian metric 9 82 136
Hermitian metric on unit ball 136
hessian 7
Hessian comparison theorem 19—20 99
Hodge theory 7 17
Holomorphic convexity 200
Holomorphic functions 15 195
Holomorphic functions of minimal degree 195
Holomorphic functions, infinity of  norm of 47 norm of 47
Holomorphic n-forms 141
Holomorphic n-forms, of slow growth in  sense 182 184 188 sense 182 184 188
Holomorphic n-forms, of slow growth in 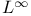 sense 184 sense 184
Holomorphic n-forms, of slow growth, nonvanishing of 188
Holomorphic sectional curvature 82 83 84 112
Holomorphic tangent space 9
Hyperbolic manifold 82 84
Hyperbolic manifold, measure hyperbolic 134
Hyperbolic metric, infinitesmal 112
Hyperbolicity 81
Imbedding by harmonic functions 15
Imbedding by holomorphic functions 114
Imbedding of convex surfaces 69
| Involutive isometry 116
Jacobi equation 26 29 30 33 55
Jacobi field 22 23 26
Kaehler form 9
Kaehler manifold 11 15 99
Kaehler manifold, holomorphic functions on, constancy implied by boundednes 57
Kaehler metric 11
Kernel form 142
Laplacian 1 7
Laplacian, comparison theorem 26—27
Laplacian, complex 12
Levi form 12 100
Levi form of functions of distance 32—33 35
Levi form, computation of 13—14
Levi problem, Grauert's solution of 14
Lu Qi-keng conjecture 178
Manifold with a pole 5
Measure hyperbolic 134
Models 2 24 69
Models, 2-dimensional 69
Moser's Harnack inequality 79
Normal coordinate system 8
Normal coordinate system, complex 11 155
Normal geodesic 6
Plurisubharmonic function 13 126
Poincare — Lelong equation 192
Pole 2 5
Pseudo-distance, Kobayashi 81 82
Pseudo-Hermitian metric 85 124 129
Pseudo-Hermitian metric, singular set of 85 129
Quasi-isometry 2 56
Quasi-isometry theorem for manifold with pole 56
Radial curvature 5 29—30 99 111 113 148
Radial curvature on a Kaehler manifold 32 35
Radial curvature, function of a model 30
Radial plane 5
Radial vector field 5
Rauch comparison theorem 20 52 75
Real form 9
Ricci curvature 5 129
Ricci form 158
Ricci tensor 11 12 129
Rotationally symmetric Hermitian metric 85
Schwarz lemma, generalized 86
Schwarz lemma, generalized, volume (Chern — Kobayashi) 131
Schwarz lemma, generalized, Yau's 176
Second variation formula 6
Singular set of a pseudo-Hermitian metric 85 129
Siu — Yau theorem on manifolds biholomorphic to 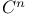 180ff 180ff
Special basis (of  holomorphic (n,0) forms) 147—148 holomorphic (n,0) forms) 147—148
Special basis (of  holomorphic (n,0) forms), Bergman metric in terms of 153 155 holomorphic (n,0) forms), Bergman metric in terms of 153 155
Spherically symmetric metric 24
Stein manifold 14 15
Stokes' theorem 44
Strictly convex function 7
Strictly plurisubharmonic function 14
Strong pseudo-convexity 176
Strongly pseudoconvex domain 177
Sturm comparison theorem 40 41
Subharmonic function 8
Subharmonic function, sub-mean value theorem for 43
Symmetric Riemannian manifold (with respect to hypersurface) 116
Totally geodesic submanifold 25 116
Transversal vector field 6
Uniform ellipticity 79
Volume function 51—52
Volume function, estimates of 52—55
Weak model 24
Yau's generalized Schwarz lemma 176
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 estimates of
estimates of 
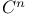
 norm of
norm of 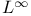 sense
sense