|
|
 |
| Àâòîðèçàöèÿ |
|
|
 |
| Ïîèñê ïî óêàçàòåëÿì |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Fiedler B. — Global Bifurcation of Periodic Solutions with Symmetry |
|
|
 |
| Ïðåäìåòíûé óêàçàòåëü |
1986 19 96
ALCON 109
Analytic semigroup 9 12 25f 31 84 91 97f 116
Answers 3 11 96—101 106f 115
Approximation 38 84
Approximation, generic 3 10 13 23 33f
Approximation, genericand equivariant 3 13 23 85—91 108—111
Arms, number of 99f 105
Arms, reduction 99
Arnold — Bogdanov — Takens singularity see “B-point”
Arrhenius kinetics 103
Artin conjecture 19 97
Assumptions, analyticity 22 34 46 87f
Assumptions, boundedness 22 25 85—90
Assumptions, equivariance 2 25
Assumptions, genericity 20 49 59 68 84 121f 129f
Assumptions, nondegeneracy 9 22 46 85—90 98 112—115
Assumptions, regularity 16 130f
auto 109
Axisymmetry 98f
B-point 109 113f
Baire space 13 26
Belousov — Zhabotinskii reaction 3—6 92 99 104f
Benard convection 104
BIFPACK 109
Bifurcation see also “B-point” “Flip” “Flip-flop” “Flop” “Freezing” “Hopf E.” “Jug-handle” “Ljapunov “Period” “Periodic” “Saddle-node” “Torus” “Stationary” “Symmetry “Type”
Bifurcation, diagram 3f 13 31f 68 86 89 109—114
Bifurcation, multiparameter 3 9 109 110 114f
Bifurcation, with two parameters 3 103 110 112—114
Binary orbit 11f 18—24 67 79 85f 94 98 101 108
Biological clock 102
Brouwer degree 4 23 27—29 110
Brusselator 92—101
Cartan decomposition 99
Catalysis 103
Catastrophe, blue sky 113 115
Catastrophe, theory 9 109
Center 16 93 95
Center and symmetry 48f
Center and virtual periods 42f 46f 85
Center index 17
Center index and orbit index 33 77—83 91 104 E.
Center manifold 9
Center, continuum of 34 46f 87
Center, generic 16
Center, generic, genericity of 48f 121f
Center, H-center 16
Change of stability 1f 9 12 17 “Hopf E.
Chaos 103 114
Characteristic equation 93f
China 17
Compactness assumption for continua 84f
Compactness assumption for groups 2 39 45 74
Compactness assumption for semiflows 25f 91 122 127f
Complexification 17 23 42 119f
Concentric wave see “Wave”
Continuum of periodic solutions see also “Center”
Continuum of periodic solutions, excluding period, global 12f 21 24 34 85f 95f 101 110f 113f
Continuum of periodic solutions, including period, global 9 31 96 100 110f
Continuum of periodic solutions, limiting 33 84f
Continuum of periodic solutions, local 107f
Continuum of periodic solutions, unbounded 21 24 27f 33f 88f 99f
Coupled oscillators 2f 8 10 92—97 102f 105
Coupled oscillators with electric coupling 103
Crossing number 1 17 78 111 “Hopf E. “Transverse”)
Crossing number, net 23 94f
Crossing number, odd 9 27 96 100
Curvature condition 16 28 30 53 57
Curvature condition, genericity of 103 130f 134
Cusp 109
Cyclic group 6
Degenerate stationary solutions 98 114f
DERPER 109
Dihedral group 3 92—97 104 112
Discrete wave see “Wave”
Equivariant see “Assumptions” “Hopf E.” “Hopf H. “Orbit “Snake” “Stationary”
Fermat's last theorem 19
Field — Koros — Noyes model 92
Fixed point subspace 5 10 12 15 45 131
Fixed point subspace and orbit index 81
Fixed point subspace, dimension of 101 102 104 108
Flip 30 57
Flip, doubling 30 55 59—61
Flip, pitchfork 59—61
Flip-flop 57
Flip-flop, doubling 59—61 65f
Flip-flop, pitchfork 59—61
FLOP 54
Flop, doubling 54 59—61 65f
Floquet multipliers 29 91 109 129 131
Floquet multipliers and Floquet exponents 69
Floquet multipliers and orbit index 70—82
Floquet multipliers and virtual periods 43
Floquet multipliers of rotating waves 134
Fluid dynamics 3 104
Fredholm map 35f 69 91 117f
Fredholm map, index 117f 123 130
Freezing 7 50f 67 81—83 114 132f
Frequency doubling 102
Frozen wave see “Wave”
Fruit of the Loom 130 133
Fuller index 9 29 110 112
GENERIC 13 (see also “Approximation” “Localization” “Perturbations”)
global see “Continuum” “Hopf E.” “Stationary”
Graph of oscillators 102f
Haar measure 39
Hamiltonian systems 9 31 102
Heteroclinic orbit 103f
Heterogeneous oscillations 3 96—105
Hint 65
Homoclinic orbit 106 113—115
homogeneous solution 3 93—98
Homogeneous solution, nonhomogeneous solution 3 96—105
Homotopy invariance of Brouwer degree 23 29 110
Homotopy invariance of the Fuller index 110
Homotopy invariance of the Hopf index 23 106 112—114
Homotopy invariance of the orbit index 13 32f 70—77 81f 91
Hopf, E. bifurcation see also “Center”
Hopf, E. bifurcation, global 1f 7 9f 110f
Hopf, E. bifurcation, global, equivariant 2f 10f 15—26 29—34 79f 82f 92—104 110 113f
Hopf, E. bifurcation, history 9f
Hopf, E. bifurcation, local 1f 9f 79
Hopf, E. bifurcation, local, equivarint 3 10 45f 48f 100 106f
Hopf, E. bifurcation, local, infinite-dimensional 9
Hopf, E. bifurcation, local, planar 9
Hopf, E. index, global equivariant 2 (see also “Center”)
Hopf, H. theorem 110
Horseshoe 114
Hyperbolicity of periodic solutions and type 0, 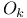 solutions 31 127 129 solutions 31 127 129
Hyperbolicity of periodic solutions and virtual period/symmetry 11 96 100
Hyperbolicity of periodic solutions at generic centers 16 123f
Hyperbolicity of periodic solutions, rotating waves 51 133
Hypercycle 102
INDEX see “Homotopy invariance” “Fredholm” “Fuller” “Hopf E.” “Orbit”
Induction over period/symmetry 116 124 127—134
Infinitesimal rotation 7 25 49 81 88
Inhomogeneous see “Homogeneous”
Instability see “Stability” “Unstable”
Integral equations 12 38 103
Irreducible see “Representation”
Isotropy group 4
Isotropy group, maximal 12 95 100f 104 106f
Isotropy group, submaximal 108 (see also “Virtual”)
Isotypic, action 119f
Isotypic, decomposition 22 74f 95 99
Iterated, maps 9 29 54 56f 62
Iterated, multiplication by two 11 18f
J-homomorphism 110
j-jug-handle 111f
Jug-handle 31f 110f
| Kupka — Smale theorem 13 31 48
Langmuir — Hinshelwood kinetics 103
Laplace operator 97f
Laplace operator, discretized 92f 98
Laser equations 104
Lattices, and virtual symmetry 45
Lattices, crystal 103
Lattices, hexagonal 104
Linked periodic solutions 32
Ljapunov center theorem 102
Ljapunov — Schmidf reduction and virtual symmetry 37 53
Ljapunov — Schmidf reduction at rotating waves 50 132
Ljapunov — Schmidf reduction at secondary bifurcations 66 130f
local see “Global”
Localization of genericity 116 122 127f
Loop in parameter space 112f
Loop of periodic solutions 31f 111f
Lorenz equation 114
Manifolds of solutions 114f
Maximal see “Isotropy” “Torus”
Minimal see “Period”
Mode interaction 104
Models 92 102f
Multiparameter see “Bifurcation”
Net crossing see “Crossing number”
Network see “Graph”
Neural nets 103
Non-free group action 5 110
Normal form 62 102 104 113f
Normal form, Arnold normal form of matrices 119—121
Numerical, algorithms 109
Numerical, analysis 4 102 109
Numerical, simulation 98 100 103
Obstruction 112—114
Odd see “Crossing number”
Operator setting for periodic solutions 7 10 31 48 66 68
Orbit index, equivariant of discrete waves 70
Orbit index, equivariant of rotating waves 81
Orbit index, nonequivariant 32 (see also “Floquet” “Homotopy” “Stability”)
Orthogonal groups, 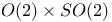 104 104
Orthogonal groups, O(2) 14 104 108f 112 114
Orthogonal groups, O(3) 92 97—101 107
Orthogonal groups, O(N) 2
Orthogonal groups, SO(2) 6 14 34 103 110 115
Parameters see “Bifurcation”
Pattern 3f 6 97 99 104
Period see also “Virtual”
Period, blowup 21 24 50 68 110—114
Period, doubling 13 18 30f 47 51—62 72—76 81 110f 127 130
Period, doubling, cascade 114
Period, jump 13 29 59f 73 86 111f 127
Period, lower bound on 90 127 129
Period, minimal 5 12 31f 49f 73 110f
Period, minimal and transversality 31 116 124f
Period, upper bound on 132 134
Periodic solutions see “Bifurcation” “Hopf E.” “Hyperbolicity” “Linked” “Operator “Poincare” “Symmetry”
Perturbations see also “Generic” “Singular” “Transversality”
Perturbations, breaking equivariance 13f 104 109
Perturbations, of centers 49 86 122f
Perturbations, of matrices 118f
Perturbations, of Poincare maps 62 66 124f
Perturbations, of rotating waves 7 132f
Perturbations, of stationary solutions 28—31
Petri dish 6
Pitchfork 30 53f 57 59f 71f 109 112 127
PITCON 109
PL methods 109
Poincare map 29f 50 55—68 118 124f 131 134
Poincare section 29 50 55 115 124 130
Poincare time 55 62f 125
Poincare — Andronov — Hopf bifurcation see “Hopf E.
Proper 117 123 130
Quaternions 114f
Questions see also “Answers”
Questions, open 68 112 115
Questions, principal 2
Reaction diffusion systems 3 10 97—101 109
Regularity see “Assumptions”
Relative, equilibrium 115
Relative, periodic solution 115
Representation, cyclic 17 22 118f
Representation, irreducible 17 22 74f 109 110 118f
Representation, subspace 2 12 22f 75 81 95 99 120
Rescaling 7 35 70—77 80
Resolvent 25f 69 91
Resonance 102 108 114
Roots of unity 30f 43 119 127 129 134
Rotating wave see “Wave”
Rotation number see “Torus”
Saddle-node bifurcation 31
Sard theorem 13 28 31 118
Sard theorem, Smale — Sard theorem 118
Scylla & Charybdis 109
Silnikov homoclinic theorem 114
Singular perturbations 96 99 101
Singularity 4 13f 48 104 109 112f
Singularity, Arnold — Bogdanov — Takens see “B-point”
Singularity, theory 9 104 106 109
Sink 80 83
Smale see “Horseshoe” “Sard
Snake 21
Snake, equivariant 79
Snake, global 33 79 82
Solution see “Manifold” “Periodic” “Stationary”
Source 80 83
Speed see “Rotating wave”
Spherical harmonics 98f 107
Spherical pendulum 102
Spiral cells 104
spirals 3f 99f 104f
Stability 104 108f
Stability and orbit index 104
Stability, exchange of 33 73 76 77—79 104
Stationary solutions, bifurcation of see also “Bifurcation” “Degenerate” “Flop” “Saddle-node” “Turn”
Stationary solutions, bifurcation of global 9 27f 34
Stationary solutions, bifurcation of global equivariant 10 110
Stationary solutions, bifurcation of local 9
Stationary solutions, bifurcation of local equivariant 10
Stratification 116
Stroboscope 66
Strongly monotone systems 109
Swallow-tail 109
Symmetry see also “Virtual”
Symmetry, breaking 10 31 52 57 59f 66 102 112 127 131 134
Symmetry, of periodic solutions 6
Symmetry, of periodic solutions, control over 9—13 21 24 96f 110f
Syntheses 34 45 112
Target pattern see “Pattern”
Taylor — Couette flow 104
Temptation 64
Topological, approach 9f 13 29 68 103 106 109—114
Topological, restrictions 4
Topological, results 3 9f 109f
Topological, “charge” 99f
Topology, of sets 84
Topology, uniform operator 25 73 77
Topology, weak 13 26 130
Torus, group 45 104
Torus, invariant 103f 114f
Torus, invariant, periodically foliated 59 104 115
Torus, invariant, primary bifurcation of 106
Torus, invariant, rotation number on 115
Torus, invariant, secondary bifurcation of 104f 114f
Torus, lattice 45
Torus, maximal 99f
Transversality 116—134
Transversality, assumption 117 124 130f
Transversality, theorem 117 122 127 130—134
Transverse crossing 16 28 30 53 57 71f 123 130f
Turing ring 2 7 92—97 102—105
|
|
 |
| Ðåêëàìà |
 |
|
|
 |
|
Î ïðîåêòå
|
|
Î ïðîåêòå



 solutions
solutions