|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Bhatia N.P., Szego G.P. — Dynamical Systems: Stability Theory and Applications |
|
|
 |
| Предметный указатель |
Solution Funnel, cross section of 3.2.10 3.2.14 3.2.15 3.2.20 3.3.13
Solution Funnel, segment of 3.2.10
Solutions of ODE, continuity of 3.1.26
Solutions of ODE, convergence of 3.2.2
Solutions of ODE, differentiability of 3.2.24 3.2.29
Solutions of ODE, differentiability of, approximate 291 3.4.4
Solutions of ODE, existence and uniqueness of 3.1.46 3.1.47
Solutions of ODE, existence of (Caratheodory) 3.1.11
Solutions of ODE, existence of (Peano) 3.1.8
Solutions of ODE, existence of, on maximal intervals 3.1.13
Solutions of ODE, extendability of 274 3.1.52 3.1.59
Solutions of ODE, maximal 3.1.31
Solutions of ODE, maximal, existence of 3.1.40 3.1.42 3.1.44
Solutions of ODE, minimal 3.1.31
Solutions of ODE, minimal, existence of 3.1.40 3.1.42
Sphere, closed, 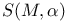 0.1.6 0.1.6
Sphere, open, 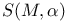 0.1.5 0.1.5
Spherical hypersurface, 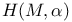 0.1.7 0.1.7
Stability additive pair 2.15.8
Stability additive pair, conditions for 2.15.9 2.15.10
Stability,  , for compact sets 2.13.20 , for compact sets 2.13.20
Stability, absolute, for compact sets 2.13.18
Stability, absolute, for compact sets, conditions for 2.13.24
Stability, asymptotic, for compact sets 1.5.16 1.5.26—1.5.28 2.6.1 2.6.12 2.6.13 2.11.37 2.11.38
Stability, asymptotic, for compact sets, componentwise 2.6.19
Stability, asymptotic, for compact sets, conditions for 2.7.1 2.7.18—2.7.20
Stability, asymptotic, for compact sets, global 1.5.16 2.6.1
Stability, asymptotic, for compact sets, global, conditions for 3.6.26 3.6.30 3.8.6 3.8.13
Stability, asymptotic, for motions 1.11.12
Stability, asymptotic, for sets 1.6.26 2.12.12 2.12.19 2.12.20
Stability, asymptotic, for sets, conditions for 1.7.8 2.12.18 2.12.24
Stability, asymptotic, for sets, semi 2.12.12
Stability, asymptotic, for sets, semi, conditions for 2.12.17
Stability, asymptotic, for sets, strong 3.3.17
Stability, asymptotic, for sets, uniform 1.6.26 2.12.12 2.12.22
Stability, asymptotic, for sets, uniform, conditions for 2.12.23 2.12.25
Stability, asymptotic, for sets, weak 3.3.17
Stability, equi, for sets 2.12.1
Stability, equi, for sets, conditions for 2.12.1
Stability, Lagrange (L-stability) 1.5.1 1.5.2 2.5.1
Stability, Lagrange (L-stability) and recurrence 2.9.12
Stability, Lagrange (L-stability), negative (L-stability) 1.5.1 1.5.2 2.5.1 2.10.12
Stability, Lagrange (L-stability), positive (L-stability) 1.5.1 1.5.2 2.5.1 2.10.14 2.10.15
Stability, Liapunov, for compact sets 1.5.6. 2.6.1
Stability, Liapunov, for compact sets, componentwise 2.6.8 2.15.5
Stability, Liapunov, for compact sets, conditions for 1.7.1 2.15.6 3.6.6 3.7.3
Stability, Liapunov, for motions 1.11.1—1.11.8 2.10.1 2.10.7
Stability, Liapunov, for motions, with respect to a set 1.11.9 2.10.12 2.10.15
| Stability, Liapunov, for sets 1.6.1—1.6.10 1.6.24 2.12.1
Stability, Liapunov, for sets, conditions for 1.7.3 2.12.6
Stability, Poisson 1.10.4 1.10.7 2.5.4
Stability, Poisson, negative 1.10.4 1.10.7 2.5.4
Stability, Poisson, positive 1.10.4 1.10.7 2.5.4 2.5.11
Stability, relative, for compact sets 2.1 5.2
Stability, relative, for compact sets, conditions for 2.15.3
Stability, uniform, for motions 2.10.9 2.10.12
Stability, uniform, for sets 1.6.1—1.6.10 2.12.1 2.12.2
Stability, uniform, for sets, conditions for, conditions for 2.12.8 2.13.30
Stakhi, A.M. 301
Stepanov, V.V. 1.1.9 1.2.48 2.5.15 2.9.14 2.11.40
Sternberg, S. 3.1.47 3.1.70
Strauss, A. 3.7.16
Strong stability properties 1.9.1
Subtangential vectors 3.4.10
Szegoe, G.P. 1.5.43 1.9.11 2.8.14 3.6.33 3.8.33
Tietze extension theorem 3.4.9
Topological transformation group 1.1.8
Trajectory 1.2.1 1.2.6 2.2.1
Trajectory segment 1.2.1
Trajectory uniformly approximating its limit set 2.10.13
Trajectory, periodic 2.4.2
Trajectory, positive (negative) semi 1.2.1 1.2.6 2.2.2 2.2.3
Trajectory, positively asymptotic 1.3.29 1.10.11
Trajectory, recurrent 2.9.4—2.9.12
Trajectory, self-intersecting 2.4.4
Trajectory, stable see “Stability”
Transition 1.1.1 1.1.3—1.1.5 115
Tube 2.11.23—2.11.25 2.11.28
Tube, compactly based 2.11.29 2.11.34 2.11.35
Uniqueness conditions for solutions of ODE 3.1.18 3.1.27 3.1.46 3.1.47 3.1.51
Ura, T. 1.3.35 1.4.14 1.5.43 2.2.17 2.3.18 2.13.31 2.15.11 298
van Kampen, E.R. 3.1.51
Vinograd, R.E. 3.1.70
Vrkoc, J. 2.7.25 3.6.33
Walter, W. 3.1.70
Wazewski, T. 1.8.6
Whitney, H. 1.1.9 2.11.40
Whyburn, G.T. 1.1.9
Wintner extendability conditions 3.1.61
Wintner, A. 277
Yorke, J. 3.1.70 3.2.19 366
Yoshizawa, T. 2.7.25 316 2.6.33
Young, G. 2.2.17
Zippin, L. 1.1.9
Zorn Lemma 1.2.48
Zubov, V.I. 1.5.43 1.6.52 1.7.16 2.7.25 2.12.28 3.6.33
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



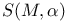
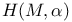
 , for compact sets
, for compact sets