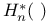Авторизация
Поиск по указателям
Abhyankar S.S., Sathaye A.M. — Geometric Theory of Algebraic Space Curves
Обсудите книгу на научном форуме Нашли опечатку?
Название: Geometric Theory of Algebraic Space CurvesАвторы: Abhyankar S.S., Sathaye A.M. Аннотация: The original main part of this book was just a sequel to the Montreal Notes [3 ]. The main part was the proof to the Theorem (36.9), namely that "All irreducible nonsingular space curves of degree at most five and genus at most one over an algebraically closed ground field are complete intersections." As such, the Theorem was completely proved in 1971. Two versions of the proof have been written and circulated, but none published. We intended to give a completely self-contained treatment of the Theorem, and in the process, the size of the proof, or rather, the preparatory material, enlarged; while the proof continued to become clearer and somewhat sharper. The present version was finally started in June 1973, and we finally decided that it had to be a book.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1974Количество страниц: 302Добавлена в каталог: 06.07.2008Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
2.1 2.2 2.5 2.10 2.64 4.3 4.13 4.4 4.6 4.2 4.3 4.5 2.1 2.7 2.9 1.1 1.4 3.21 2.25 2.72 2.75 2.45 2.54 2.4 1.4 1.30 1.6 1.20 1.28 4.3 1.15 1.16 1.20 3.2 4.19 1.3 5.13 5.18 2.2 2.4 4.12 2.8 2.7 2.10 2.11 2.12 1.2 2.13 2.16 1.4 2.2 2.4 2.15 2.17 2.14 2.17 2.54 2.73 2.74 2.75 2.89 2.83 2.31 2.86 3.2 adj( ), tradj( ) 1.15 1.16 1.21 adjoint, true adjoint 1.16 1.17 1.21 1.28 1.30 adjoint, true adjoint, over-, an undefined descriptive 1.17 2.78 adjoint, true adjoint, under-, an undefined descriptive 2.79 Bezout's theorem for hypersurfaces 5.12 Bezout's theorem for plane curves 2.89 Bezout's theorem, "little" 2.65 Complementary module 3.1 Complete intersection 4.15 Conductor 1.3 Conversion formula for different 3.12 3.18 Cusp types 1.37 d-chord 2.90 d-secant 2.90 Dedekind's formula for conductor and different 3.1 3.11 3.19 5.28 Dedekind's formula for conductor and differential 3.24 Degree (filtered case) of elements 4.3 Degree (filtered case) of filtered domains 4.13 Degree (filtered case) of principal ideals 4.3 Degree (homogeneous case) of a homogeneous domain with dimension one 2.75 Degree (homogeneous case) of a homogeneous domain with dimension zero 2.65 Degree (homogeneous case) of elements 2.1 Degree (homogeneous case) of principal ideals 2.5 Dehomogenization 4.5 Dehomogenization, natural 4.6 Different 3.1 5.26 5.28 Differential 3.22 5.24 Differential, order of 3.24 Dim( ) 2.2 Double points (geometric words for) 1.37 Elementary step 4.18 Elementary transformation 4.18 Emdim( ) 2.2 2.4 Essentially hyperplanar, planar 4.15 Euclidean, chain of euclidean curves 5.19 Euclidean, chain of euclidean domains 5.19 F*( ) 1.1 Filterd, domain 4.1 Filterd, homomorphism 4.2 Filterd, subdomain 4.2 Filtration 4.1 Filtration, natural 4.2 Flat 2.7 Function field 2.2 Genus 3.22 3.41 3.52 4.13 genus( ) 3.23 3.42 3.53 4.14 Genus, formulas 3.25 3.42 3.52 3.53 Ground field of a filtered domain 2.1 Ground field of a homogeneous domain 4.1 H*( ), 2.5 2.9 Homogeneous, coordinate system 2.20 Homogeneous, dimension 2.2 Homogeneous, domain 2.1 Homogeneous, embedding dimension 2.2 2.4 Homogeneous, homomorphism 2.3 Homogeneous, ideal 2.3 Homogeneous, localization 2.13 2.16 Homogeneous, subdomain 2.3 Homogenization 4.3 Homogenization, natural 4.4 Length as affine intersection multiplicity 1.6 1.20 4.2 Length as projective intersection multicity 2.53 2.73 2.89 5.12 Length of a module 1.1 Model of a homogeneous domain 2.2 Model, normal 1.3 node types 1.37 ord( ) 2.23 2.28 Osculating flat 2.46 2.55 Projecting center, good, better, best 2.90 Projection (filtered case) 4.11 Projection (filtered case), birational 4.11 Projection (filtered case), integral 4.11 Projection (homogeneous case) 2.7 2.9 Projection (homogeneous case), 2.28 2.29 Projection (homogeneous case), birational 2.9 Projection (homogeneous case), formulas 2.68 2.69 5.17 Projection (homogeneous case), Lemmas 2.25 2.27 Projective center of a valuation 2.14 2.17 Separably generated 3.9 Separating transcendental 3.9 T( ) 2.84 Tangent cone to a hypersurface (algebraic definition) 2.84 Tangent flat 2.73 2.76 Uniformizing, coordinate 3.32 Uniformizing, parameter 3.32 Valued vector space 2.30 [( ):( )] 1.1
Реклама
 |
|
О проекте
|
|
О проекте



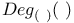
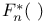
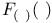
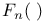
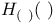
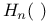 , H( )
, H( ) 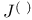 (projection symbol)
(projection symbol) 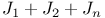 ,
, 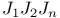 ,
, 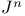 ,
, 
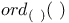
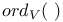
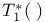
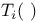 ,
, 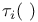
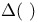
 (conventions of)
(conventions of) 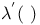
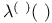
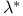
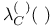
 ,
, 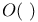
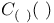
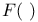 ,
, 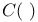
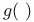
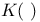 ,
, 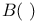
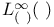

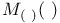
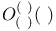
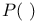
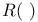
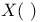 ,
, 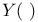
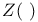
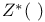
 ,
, 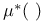 ,
, 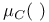 ,
, 
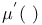
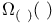 , Z( ), Z'( ), Y( ), Y'( ), T*( ),
, Z( ), Z'( ), Y( ), Y'( ), T*( ), 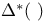 , P( )
, P( )  -quasihyperplane,
-quasihyperplane, 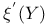 ,
,