Авторизация
Поиск по указателям
Van Neerven J. — The Adjoint Of A Semigroup Of Linear Operators
Обсудите книгу на научном форуме Нашли опечатку?
Название: The Adjoint Of A Semigroup Of Linear OperatorsАвтор: Van Neerven J. Аннотация: This monograph provides a systematic treatment of the abstract theory of adjoint semigroups. After presenting the basic elementary results, the following topics are treated in detail: The sigma (X, X )-topology, -reflexivity, the Favard class, Hille-Yosida operators, interpolation and extrapolation, weak -continuous semigroups, the codimension of X in X , adjoint semigroups and the Radon-Nikodym property, tensor products of semigroups and duality, positive semigroups and multiplication semigroups. The major part of the material is reasonably self-contained and is accessible to anyone with basic knowledge of semi- group theory and Banach space theory. Most of the results are proved in detail. The book is addressed primarily to researchers working in semigroup theory, but in view of the "Banach space theory" flavour of many of the results, it will also be of interest to Banach space geometers and operator theorists.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2008Количество страниц: 208Добавлена в каталог: 05.07.2008Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
1.3 1.5 2.5 3.2 3.4 5.1 6.1 6.2 8.1 8.4 Adjoint semigroup 1.2 AL-space 8.3 AM-space 8.3 Approximation property 7.3 atom 8.4 Axiom K 5.5 Baire-1 functional 5.2 5.3 Banach lattice 8.1 Band 8.1 Band projection 8.1 Basis 1.5 2.1 2.3 3.2 6.2 Basis constant 1.5 2.3 Basis, boundedly complete 1.5 3.2 Basis, Schauder see "Basis" Basis, shrinking 1.5 3.2 Basis, summing 2.3 6.2 Basis, unconditional 1.6 8.4 Bilinear form 7.2 Bosom 1.3 Cauchy problem 4.3 Characteristic 2.6 Circled 2.3 Co-dimension 1.5 5.2 6.2 Complexification 8.4 8.6 Coordinate functional 1.5 Dedekind complete 8.1 Dedekind complete, 8.1 Disjointness 8.2 Domain 1.1 Dual, semigroup- 1.3 Dunford — Pettis property 1.5 2.5 5.2 6.2 8.4 Embedding A1 Embedding, 3.2 Embedding, natural, j 1.3 2.3 Embedding, natural, k 5.1 Embedding, semi- 3.2 Equicontinuous 2.2 Equicontinuous, weakly 2.2 Essentially separably valued A2 Exponential formula A3 Factorisation scheme 6.3 7.4 Favard class 3.2 3.4 4.2 4.4 6.2 Frechet space 1.6 Gagliardo completion 3.5 Generator A3 Generator of intertwined semigroup 3.1 4.4 4.5 Generator of regular semigroup 4.4 Generator, weak*- 1.2 4.4 Graph 1.1 Grothendieck property 1.5 1.6 5.2 6.2 Group, A3 5.3 6.2 Growth bound 4.4 8.4 Hille — Yosida operator 3.1 Hille — Yosida operator, generalized 3.1 Hille — Yosida operator, holomorphic 3.1 3.5 Hoelder space 3.4 Ideal 8.1 Ideal, M- 2.6 Ideal, operator- 2.2 7.3 Injection A1 Integral, Bochner A2 Integral, Pettis 5.2 A2 Integral, Riemann weak*- 1.6 A2 Integral, weak*- 1.2 5.2 A2 Integrated semigroup 2.5 4.4 Integrated semigroup, exponentially bounded 4.4 Integrated semigroup, locally Lipschitz 4.4 Integrated semigroup, non-degenerate 4.4 Interpolation space 3.3 James function space JF 8.3 James space J 1.5 6.2 K-functional 3.3 Laplace transform A3 Lattice isomorphism 8.1 Lebesgue number 2.2 Limes superior estimate 8.2 Mackey topology 2.6 Martin's Axiom 8.3 Measurable, Riemann 8.3 Measurable, strongly A2 A3 Measurable, weakly A2 5.2 8.3 Measurable, weakly Borel A2 5.2 8.3 Modulus 8.1 Modulus of an operator 7.4 8.4 Norm, 1.3 2.3 3.2 Norm, cross 7.2 Norm, injective tensor 7.2 Norm, projective tensor 7.2 Norming A2 Null set, E- 8.4 Operator, adjoint 1.1 Operator, band preserving 8.4 Operator, closed 1.1 Operator, cone absolutely summing 7.4 Operator, densely defined 1.1 Operator, integral 7.3 Operator, l-nuclear 7.4 Operator, nuclear 7.3 Operator, positive 8.1 Operator, r-compact 7.4 Operator, representable 6.2 7.3 Operator, weakly compact A1 2.5 6.3 Order bounded set 8.1 Order continuous norm 1.6 8.1 8.2 8.3 8.4 Order interval 8.1 Order unit 8.3 Order unit, weak 8.4 Part of an operator 1.3 Perturbation 4.1 4.2 Pettis Integral Property (PIP) 5.5 polar 2.3 Projection band 8.1 Quasi-complete 2.6 Quasi-interior point 8.2 8.6 Quasi-reflexive 2.5 6.2 6.3 Radon — Nikodym Property (RNP) 1.6 3.1 6.2 7.4 Reflexive A1 1.3 1.5 3.1 3.2 8.4 Representation space 8.4 Resolvent 1.4 Resolvent identity 1.4 Resolvent set 1.4 Riesz space 8.1 Riesz space homomorphism 8.1 Riesz space isomorphism 8.1 Riesz subspace 8.1 Schauder basis see "Basis" Schauder decomposition 1.6 Semigroup, A3 Semigroup, A3 3.1 5.3 6.2 Semigroup, adjoint 1.2 Semigroup, compact 5.2 Semigroup, contraction A3 Semigroup, convolution 6.2 Semigroup, holomorphic 5.2 Semigroup, intertwined 3.1 4.1 4.4 Semigroup, lattice 8.1 Semigroup, locally bounded A3 Semigroup, multiplication 8.4 8.5 Semigroup, one-parameter A3 Semigroup, Pettis integrable 5.2 Semigroup, positive 8.1 Semigroup, quotient 2.5 5.2 Semigroup, regular 4.4 Semigroup, rotation 1.3 Semigroup, strongly continuous A3 Semigroup, strongly measurable A3 5.3 6.2 Semigroup, summing 2.3 6.2 Semigroup, translation 1.3 2.2 5.2 6.1 7.1 7.3 7.4 8.2 8.3 Semigroup, uniformly continuous A3 1.3 3.2 6.2 Semigroup, weak*-continuous A3 1.6 4.4 6.2 Semigroup, weakly Borel measurable 5.2 8.3 Semigroup, weakly compact 5.2 6.2 8.4 Semigroup, weakly continuous A3 Solid hull 8.1 Solution, classical 4.3 Solution, mild 4.3 Spectrum 1.4 Stone — Cech compactification 8.4 Stonean 8.4 Subcouple 3.3 Subcouple, exact 3.3 Sublattice 8.1 Sun 1.3 Tensor product, algebraic 7.2 Tensor product, injective 7.2 Tensor product, l- 7.4 Tensor product, projective 7.2 Theorem, Banach — Alaoglu A1 Theorem, bipolar 6.1 Theorem, Bochner A2 Theorem, closed graph A1 Theorem, dichotomy 5.3 Theorem, Eberlein — Shmulyan A1 2.2 Theorem, Gantmacher A1 Theorem, Goldstine A1 Theorem, Grothendieck 7.3 Theorem, Hahn — Banach A1 6.1 Theorem, Hille — Yosida A3 Theorem, Kakutani 7.3 8.3 Theorem, Kakutani — Krein 5.2 8.3 Theorem, Lotz 1.5 1.6 6.2 8.6 Theorem, measurable semigroup A3 5.3 6.2 Theorem, Odell — Rosenthal 5.2 8.3 Theorem, open mapping A1 Theorem, Pettis measurability A2 5.3 7.1 Theorem, Riddle — Saab — Uhl 5.2 5.4 8.3 Theorem, Sobczyk 6.2 6.3 Theorem, uniform boundedness A1 Theorem, weak semigroup A3 1.5 5.2 Theorem, Wiener — Young 8.2 Theorem, Zippin 1.5 3.2 Three space problem 5.5 6.1 Topology, 1.6 Topology, 2.1 Topology, 2.6 Topology, weak A1 Topology, weak*- A1 Universally measurable 5.2 Variation 7.1 Vector lattice 8.1 Weakly Compactly Generated (WCG) 1.6 2.5 6.3 ZFC 5.5
Реклама
 |
|
О проекте
|
|
О проекте



 -reflexive
-reflexive  -
-  -
- 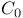 -
- 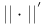 -
- 

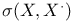 -
-