|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Soardi P.M — Potential Theory On Infinite Networks |
|
|
 |
| Предметный указатель |
0-chain 9
0-chain, finite 9
1-chain 8
1-chain of finite energy 32
1-chain, finite 8
1-transitive 5 99
Associated Markov chain 14
Automorphism group 5 88 169
Automorphism group, compact 152 153
Automorphism group, topology 151
Automorphism of a graph 5
Automorphism of a network 7
Automorphism, bounded 73—76
Boundary see "Royden’s boundary"
Boundary of 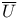 155 155
Boundary of a tile 126
Boundary of a tree 121—125
Boundary, combinatorial 82 155 170
Boundary, harmonic 134
Boundary, operator 9 115
Boundary, point (combinatorial) 20
Branch see "Edge"
Branching continuous fraction 57
Canonical decomposition 54
Capacity 122
Cartesian product of graphs 77
Cascade, grounded 114
Cascade, ungrounded 109
Cascade, uniform 110 115 116
Cayley graph 16 120
Coboundary 10
Cochain 10
Conductance of an edge 7
Conductance of finite energy 33 39
Conductance, effective between a point and  46 46
Conductance, effective between two points 25
Conductance, limit 38 39 73
Conductance, minimal 39 73
Conductance, sources (external) 12
Conductance, total 7 8 114 115
Conductance, uniqueness 18 35 72
Cutting edges 7
CYCLE 10
Cycle, finite 10 12 13
Cycle, spaces 38 115
Degree of a homogeneoue tree 6
Degree of a vertex 4
Degree, bounded 82 161
Dipole 9
Dirichlet, algebra 132 154
Dirichlet, finite function 34
Dirichlet, principle 29 51
Dirichlet, space 35
Dirichlet, sum 34
Distance (geodesic) in a graph 5 161
EDGE 3
Edge graph 126
Endpoints 3
ends 79 87 96
Ends of a tree 121
Energy of a 1-chain 28 32
Energy, sources 12
Exhaustion of a graph 4
Exhaustion of a network 8
Extension (of a 1-chain) 31
Extremal length 63
Extremities see "Endpoints"
Flow 12
Flow of value m 100
Foster’s averaging formula 25 96
Fourier transform (on the torus) 105
Graph 3
Graph, connected 5
Graph, countable 5
Graph, locally finite 4
Graph, recurrent 17
Graph, transient 17
Graph, uniformly embedded 89
Green, function 17
Green, operator 17
Growth, exponential 82 125 127
Growth, linear 87
Growth, moderate 87 88 96 98 172
Growth, polynomial 75—77
Growth, subexponential 87 98
Harmonic function 19
Harmonic function, bounded and Dirichlet finite 62 131
Harmonic function, Dirichlet finite 59
Harmonic function, HD-minimal 139
Harmonic function, nonnegative 62
Harmonic, boundary see "Boundary"
Harmonic, kernel 149
| Harmonic, measure 146
Isomorphism of graphs 4
Isomorphism of networks 7
k-fuzz of a graph 162
Kirchhoff’s equations 12 13 33 34 115
Kirchhoff’s equations, homogeneous 14 72
Kirchhoff’s laws see "Kirchhoff’s equations"
Laplacian (discrete) 16
Law of conservation of the energy 28 38 42
Length of a path 5
Limit set 93
Loop law 12
Lyons, better test of transience 51
Lyons, product of 1-chains 100
Lyons, simple criterion of transience 45
Minimum principle 20 135 136
Morphism of a graph 4
Morphism of a network 7
Nash — Williams theorem 55
Neighbour 4
Network, finite 22
Network, generalized 115
Network, recurrent 17
Network, transient 17
Node see "Vertex"
Node law 12
Open-circuit resistance parameters 112
Orbit 87 169
Orientation of a graph 4
Path 5
Path, finite 5
Path, one-ended (one-sided) 5
Path, two-ended (two-sided) 5
Path, unbranched 119
Point see "Boundary point" "Vertex"
Point,  139 139
Point, interior 20
Point, isolated in  139 151 139 151
Poisson’s equation 16 34
Potential 13 24 43 49 108
Potential kernel 105 106
Random walk 17
Random walk, operator 16
Rayleigh’s principle 29 52
Resistance of an edge 7
Resistance, degree at a vertex 97
Resistance, effective between a node and  45 51 54 45 51 54
Resistance, effective between two nodes 25 54
Resistance, limit 38 96
Resistance, minimal 42 96
Resistance, operator 12
Restriction of a 1-chain 39
Rough isometry 160
Roughly isometric 161
Royden’s boundary 134
Royden’s compactification 134
Royden’s decomposition theorem 60 132
Self-loop 4
Shorting vertices 8
Sobolev — Dirichlet inequality 86
Stage of a cascade 109 114
Strong isoperimetric inequality 82
Strong isoperimetric inequality and rough isometries 171
Strong isoperimetric inequality for hyperbolic graphs 95
Strong isoperimetric inequality for trees 119
Subgraph 4
Subgraph, induced 4
Subharmonic function 19
Subharmonic function, Dirichlet finite 46
Subnetwork 7
Subnetwork, compactification 154
Superharmonic function 19
Superharmonic function, Dirichlet finite 46
Superharmonic function, Evans 137
Superharmonic function, integral representation 146
Superharmonic function, nonnegative 20 61
Terminal of a cascade 110 114
Terminal of a tree 119
Thomson’s principle 28 41
Tile 125
Tiling 89 126
Transition probabilities 14 17
TREE 6
Tree, binary 153
Tree, homogeneous 6 19 57 119 145
Union of graphs 4
Uniqueness 32 72
Vertex 3
Vertex transitive 5
Virtanen’s theorem 62
Voltage generators (internal) 12
Walk 65
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте