|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Fienberg S.E. Ч Analysis of Cross-Classified Categorical Data |
|
|
 |
| ѕредметный указатель |
 see УLikelihood-ratio statistic see УLikelihood-ratio statistic
Additive models 5
Aitkin, M.A. 80 177
Altham, P.M.E. 32 177
Analysis of covariance 3 see
Andersen, A.H. 167 177
Anderson, J.A. 137 177
Anderson, J.B. 17 177
ANOVA, analogies with 3Ч4
Antecedent variables 123 see
Arc sine transformation 23
Armitage, P. 23 177
Ashford, J.R. 66 67 177
Association, measures of see УCross-product ratioФ
Asymptotic standard errors 83Ч84 88 104 170
Banwart, A. 121 188
Bartlett, M.S. 1 5 36 177
Baseball wins and losses 152Ч154
Beaton, A.E. 45 177
Benedetti, J.K. 80 177
Berkson, J. 6 22 177
Bhapkar, V.P. 6 31 88 177
Bickel, P.J. 53 177
Binary response 6 see univariateФ
Binomial distribution, comparing two proportions 8Ч10 13Ч15
Binomial distribution, for paired comparisons 150Ч152
Birch, M.W. 6 31 33 177
Bishop, Y.M.M. xi 2 5 6 19 23 33 38 39 49 56 60 71 73 74 83 84 96 97 98 99 117 142 143 144 147 150 153 166 175 177 178
Blalock, H.M., Jr. 1 121 178
Bliss, C.I. 1 95 178
Bloomfield, P. 43 178
Blyth, C.R. 50 178
Bock, R.D. 2 6 62 88 178
Bradley Terry model 150Ч154
Brier, S.S. 32 134 178
Brown, D.T. 38 81 178
Brown, M.B. 56 80 177 178
Brunswick, A.F. 148 178
Bunker, J.P. 5 6 179
Campbell, B.J. 90 184
Causal analysis 120Ч138
Chen, T.T. 113 142 179
Chi-square distribution, percentage points of 171
Clayton, D.G. 62 179
Closed-form estimates see УDirect estimatesФ
cluster sampling 32
Cochran, W.G. 1 48 136 179 188
Cohen, J.E. 32 179
Coleman, J.S. 125 126 179
collapsing tables 48Ч51 153Ч156
Complex-sample survey data 31Ч32
conditional tests 57Ч58 81
Conover, W.J. 22 179
Consistent estimator 106 166
Continuation ratios 110Ч111 114Ч116
Continuous explanatory variables see УLogit model continuous
Contrasts see УLinear contrastФ
Cornfield, J. 6 17 105 179 189
Correction for continuity 21Ч22
Cox, D.R. xi 2 3 6 22 23 71 85 98 101 102 165 179
Cross-product ratio as linear contrast 16 19
Cross-product ratio, association measures based on 18
Cross-product ratio, asymptotic variance of 18
Cross-product ratio, definition of 17
Cross-product ratio, equality of 36Ч37
Cross-product ratio, historical development 4Ч5
Cross-product ratio, interpretation of 17
Cross-product ratio, invariance 17 135
Cross-product ratio, properties of 17 135
Cross-product ratio, related to u-terms 16 19
Cross-product ratio, use in substantive fields 17
Daniel, C. 85 179
Darroch, J.N. 5 6 38 49 51 60 63 180
Davidovits, P. 17 177
Dawber, T.R. 6 180
Degrees of freedom, adjusting for empty cells 142
Degrees of freedom, for complete tables 74Ч75
Degrees of freedom, for quasi-independence 145
Degrees of freedom, for three-dimensional models 41
Degrees of freedom, general formula 40 142
Delta method 83
Deming, W.E. 38 180
Deming-Stephan procedure see УFitting iterative
Dempster, A.P. 2 3 180
Descriptive level of significance 165Ч166
Design effect 32
Deviance see УLikelihood-ratio statistic
Dillon, W.R. 106 182
Direct estimates, asymptotic variances for 170
Direct estimates, asymptotic variances for complete tables 32Ч34 72Ч73
Direct estimates, differences between models 60Ч61
Direct estimates, equivalence to iteration 39Ч40
Direct estimates, partitioning  for models with 60Ч61 for models with 60Ч61
Discriminant analysis 105Ч109
Doering, C.R. 84 186
Draper, N.R. 80 180
DuMouchel, W. 99 180
Duncan, B. 54 180
Duncan, D.B. 3 104 111 189
Duncan, O.D. 54 120 134 153 155 156 180
Dyke Ч Patterson data 84Ч88 98Ч99
Dyke, G.V. 84 85 86 180
Dykes, M.H.M. 9 180
Efron, B. 104 118 180
Empty cells see УФZero entries
Epidemiological studies 6Ч7 31 135Ч137
Estimated expected values see УMaximum-likelihood estimatesФ
Estimation, methods of 5Ч6
Exact tests see УHypergeometric distributionФ
Explanatory variables 2 15
Farewell, V.T. 137 180
Fay, R.E., III 32 181
Fellegi, I. 32 181
Feller, W. 15 181
Fetal monitoring data 104Ч105 117
Fienberg, S.E. xi 2 5 6 19 23 25 33 38 39 49 52 56 60 71 73 83 104 105 106 111 113 117 142 143 144 147 150 151 152 153 166 172 175 178 179 181 187
Fisher, F.M. 134 181
Fisher, R.A. 15 181
Fitting, iterative proportional, comparison with Newton Ч Raphson, algorithm 63 64 103Ч104
Fitting, iterative proportional, complete tables 37Ч40 74
Fitting, iterative proportional, convergence of 38
Fitting, iterative proportional, incomplete tables 144Ч145 147Ч148
Fitting, iterative proportional, ordered categories 63Ч66
Fleiss, J.L. 1 181
Foreman, HД 121 188
Forrest, W.H., Jr. 5 6 179
Fourfold table (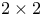 table), descriptive parameters of 8Ч15 16Ч19 table), descriptive parameters of 8Ч15 16Ч19
Framingham study 6Ч7
Frankel, M.R. 32 184
Freedman, D. 53 182
Freeman, D.H., Jr. 32 88 169 170 184 185
Freeman, J.L. 32 184
Friedman, E.A. 104 105 106 187
Gait, J.J. 18 182
Generalized iterative scaling 63Ч66
Gokhale, D.V. 2 38 104 182
Goldberger, A.S. 134 182
Goldstein, M. 106 182
Good, I.J. 6 72 182
Goodman, L.A. 2 5 6 18 56 57 60 61 63 71 73 74 77 79 80 83 84 97 120 123 125 128 138 143 157 158 159 182 183
Goodness-of-fit, asymptotic distribution of test statistics for 40 see
Gottfredson, M.R. 93 189
Greenland, S. 104 105 106 187
Grizzle, J.E. 2 6 22 37 62 136 148 149 183
Guttman scaling 155Ч159
Haberman, S.J. xi 2 3 22 23 31 38 43 62 64 66 88 101 102 103 104 143 144 147 167 168 175 183 184
Hagen, E.P. 44 189
Half-normal plot 86
Hamdan, M.A. 4 185
Hammel, E.A. 53 177
| Hansen, W.L. 112 184
Hartley, H.O. 171 187
Heckman, J.J. 134 184
Heron, D. 5 187
Hewlett, P.S. 23 184
Hierarchical models 43 72Ч73
Hierarchy of models 57Ч58 65 68Ч69
Hierarchy principle 43
Hinkley, D.V. 165 179
Hoaglin, D. 171 184
Hocking, R.R. 113 184
Holland, P.W. xi 2 5 6 19 23 33 38 39 49 60 71 73 83 117 142 143 144 147 153 166 175 178
Homogeneity of proportions 13
Hoyt, C.J. 91 184
Hypergeometric distribution 22 31
Imrey, P.B. 160 184
Incomplete arrays 142Ч150
Independence, conditional 28
Independence, for 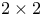 tables 10Ч13 tables 10Ч13
Independence, in rectangular arrays 13Ч15
Independence, in three-dimensional arrays 27Ч28
Independence, possible models of 72
Independence, quasi-independence 142Ч146
Indirect estimates see УFitting iterative
Intervening variable 123
Ireland, C.T. 38 184
Iterative proportional fitting see УFitting iterative
Iterative scaling see УFitting iterative
Jennrich, R.I. 101 184
Johnson, W.D. 160 184
Jureen, L. 124 189
Kannel, W.B. 6 105 180 189
Kastenbaum, M. 5 6 184 188
Katz, B.M. 69 184
Kempthorne, O. 22 184
Kihlberg, J.K. 90 184
Killion, R.A. 6 184
Kimura, M.L. 17 184
Kish, L. 32 184
Koch, G.G. 2 6 31 32 37 62 88 160 169 170 177 183 184 185
Koehler, K.J. 175 185
Krishnaiah, P.R. 91 184
Kruskal, W.H. 5 18 56 183 185
Ku, H.H. 2 56 60 88 185
Kullback, S. 2 38 56 60 88 136 182 184 185
Kupperman, M. 60 185
Lancaster, H.O. 2 4 60 185
Larntz, K. 41 144 151 162 172 173 174 181 185
Lauh, E. 71 85 179
Least squares, weighted 99 101 104
Lee, S.K. 88 170 174 185
Lerman, S.R. 136 186
Light, K.J. 172 186
Likelihood-ratio statistic,  see also УPartitioning chi-square likelihood see also УPartitioning chi-square likelihood
Likelihood-ratio statistic, asymptotic distribution of 40 166 174Ч176
Likelihood-ratio statistic, compared with Pearson's chi-square statistic 40Ч41 172Ч173
Likelihood-ratio statistic, definition of 40
Likelihood-ratio statistic, differences of, for nested models 56Ч57 175Ч176
Likelihood-ratio statistic, small-sample behavior of 172Ч174
Lindley, D.V. 51 83 185 186
Lindsey, J.K. xi 2 186
Linear contrast see also УLoglinear modelФ; Уu-termsФ
Linear contrast, asymptotic variance of 82Ч84
Linear contrast, definition of 82
Lizard data 11Ч13 27Ч29 35Ч40 42Ч43
Logistic regression see УLogistic response functionФ
Logistic response function see УLogit modelФ
Logistic response function, comparison with discriminant, function 105Ч109
Logistic response function, multivariate 110Ч116
Logistic response function, univariate 22 102Ч109
Logit model, assessment of predictive ability 99 104
Logit model, categorical predictors 95Ч99
Logit model, continuous predictors 102Ч109
Logit model, definition of 96
Logit model, nonrecursive systems of 133Ч134
Logit model, ordered categorical predictors 99Ч102
Logit model, recursive systems of 123Ч133
Logit model, simultaneous equations model involving 133Ч134
Loglinear contrast see УLinear contrastФ
Loglinear model see also УLinear contrastФ; УCross-product ratioФ; УDirect estimatesФ
Loglinear model, for 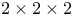 table 27Ч29 table 27Ч29
Loglinear model, for 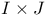 table 13Ч15 table 13Ч15
Loglinear model, general 29 71Ч77
Lombard, H.L. 84 186
Lyell, L.P. 6 180
Mallar, C.D. 134 186
Manski, C.F. 136 186
Marginal totals, analyses on 20Ч21
Marginal totals, fixed, by conditioning 97Ч99
Marginal totals, fixed, by design 95Ч96
Marginality constraints see УHierarchical modelsФ
Margolin, B.H. 172 186
Mason, W.M. 111 112 181 189
Maximum-likelihood estimates for higher-dimensional tables 71Ч77
Maximum-likelihood estimates for incomplete tables 142Ч150
Maximum-likelihood estimates for independence 13
Maximum-likelihood estimates for loglinear models with ordered categories 61Ч68
Maximum-likelihood estimates for three-dimensional loglinear models 32Ч40
Maximum-likelihood estimation, definition of 165Ч166
Maximum-likelihood estimation, equivalence of 15Ч16 31
Maximum-likelihood estimation, existence of 168
Maximum-likelihood estimation, for multinomial 169
Maximum-likelihood estimation, for Poisson 167
Maximum-likelihood estimation, for product-multinomial 169
Maxwell, A.E. 1 186
McFadden, D. 136 186
McHugh, R.B. 161 186
Meehl, P.E. 51 186
Meier, P. 9 180
Minimal sufficient statistics see УSufficient statistics minimalФ
Minimum modified chi-square statistic 37
Missing observations 113 160
Mitra, S.K. 5 188
MLE see УMaximum-likelihood estimatesФ
Model see УLoglinear modelФ
Model, selection of 56Ч61 77Ч80
Model, simplicity in building a 56
Moore, R.H. 101 184
Morgan, B.J.T. 160 186
Morris, C. 175 186
Mosteller, F. 5 6 17 99 152 178 179 186
Multinomial distribution, asymptotic Multinomial distribution, variance of loglinear contrasts for 82Ч84
Multinomial distribution, estimation for 30Ч31 168Ч169
Multinomial distribution, product-multinomial 15 30Ч31 168Ч169
Multinomial distribution, sampling distribution 15 30 31
Multivariate analysis, forms of 2Ч4
Muthen, B. 134 186
Narragon, E.A. 90 184
National Halothane Study 6 99
NBER TH data 20Ч21 44Ч48 61 149Ч151
Nelder, J.A. 43 99 101 104 186 187
Nerlove, M. 3 62 104 134 187
Nested models, differences in  56Ч57 56Ч57
Neutra, R.R. 104 105 106 187
Newton Raphson algorithm 63Ч64 66 104
Newton's method, unidimensional 63
Novick, M.R. 51 186
O'Connell, J.W. 53 177
Odds ratio 105 see
Odoroff, C.L. 172 187
Ordered categories 61Ч68 99Ч102
Owens, M.W.B. 32 187
Oxspring, H.R 113 184
Paired comparisons 150Ч154
Parameters see Уu-termsФ
Parsimony 56
Partitioning chi-square, Lancaster's method 4 5 60
Partitioning chi-square, likelihood ratio 57Ч61 74Ч77
Path diagrams 120Ч123 128
Patterson, H.D. 84 85 86 180
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте




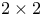 table), descriptive parameters of
table), descriptive parameters of 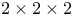 table
table 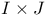 table
table