|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Bridges Th.J., Furter J.E. — Singularity Theory and Equivariant Symplectic Maps |
|
|
 |
| Предметный указатель |
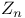 (cyclic group) 1 14 63 85 (cyclic group) 1 14 63 85
 (dihedral group) 18 69 89 133 (dihedral group) 18 69 89 133
action 11.
Action, action functional 10
Action, Lagrangian action 10.
Action-angle variables 8
Admissable variations 11
Algebraic transversality 53
Area-preserving map 85 101 146 174
Arnold, V. 9 21 47 151
Aubry, S. 2.
Averaging over the group 49 53 59 211.
Bartsch, T. 5 127—129
Bernstein, D. 2
Bhowal, A. 164.
Bifurcation, degenerate 77 101.
Bifurcation, diagrams 74 77 98—99 105—106 108 113 115 159 163—165 183
Bifurcation, multiparameter 9 85
Bifurcation, reduced bifurcation equation 19 20 137—138 155
Block diagonalization 16 121 125.
Bridges, T. 6 7 144—145 149 151 164 173—174 193
Bruce, J. 48.
Casimir function 146 147 173
Catastrophe map 45
Category, Lusternik — Schnirelmann 5 129.
Category, WII-category 5 129
Circulant matrix 14
Clapp, M. 5 128.
Classification theorems for paths 47.
Classification theorems, 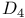 problems 90. problems 90.
Classification theorems, 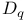 , problems 89. , problems 89.
Classification theorems, 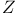 q problems 70 73 74 q problems 70 73 74
Classification theorems, 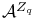 for potentials 65. for potentials 65.
Codimension, 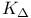 47—48. 47—48.
Codimension, gradient 35 38 39 50
Codimension, infinite 34.
Codimension, topological 70 73 74 89
Collision of multipliers at third root of unity 117.
Collision of multipliers at ±i 160.
Collision of multipliers, collision singularity 23 189
Collision of multipliers, irrational 149 171.
Collision of multipliers, rational 149
Collision of multipliers, rational, nonlinear 150.
Collision of multipliers, secondary 164 170 171.
Configuration space 11.
Configuration space, linear stability in 190—192.
Configuration space, signature in 185—190
Contact group 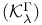 36 36
Critical dimension 127
Curl operator 49
Curve selection lemma 58
Cushman, R. 7 142 144—145 149 173—174 193.
Cyclic group see 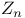
Damon, J. 45 46 52 53 58 212
Darboux theorem 122 202
Davis, P. 14 15 88
Dellnitz, M. 175 202
Dewar, R. 199 200.
Discriminant variety 45
Distinguished parameter 3 89.
du-Plessis, A. 48.
Elliptic fixed point 9
Equivalence, 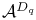 89 91. 89 91.
Equivalence, 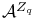 63—65. 63—65.
Equivalence, 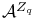 (for gradients) 68—69 (for gradients) 68—69
Equivalence,  89. 89.
Equivalence, dynamical 199
Equivalence, equivariant contact 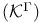 33 35 36 33 35 36
Equivalence, equivariant right 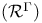 3 33 3 33
Equivalence, left-right 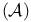 34 34
Equivalence, path 2 34 40—41
Equivalence, topological 70
Equivariant, preparation theorem 55 56 62
Equivariant, singularity theory 33
Equivariant, splitting lemma 3 20 124—125 185
Equivariant, symplectic map 119.
Field, M. 58.
Fixed-point subspace 55 126 214.
Floquet theory (discrete) 24
Floquet, multipliers, exponents 24 31 167.
Flowcharts, 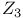 -invariant potentials 68. -invariant potentials 68.
Flowcharts, 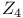 -invariant potentials 78. -invariant potentials 78.
Flowcharts, 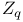 q-invariant potentials 80 q-invariant potentials 80
Fourier matrix 18 125.
Fundamental lemma 46 61
Fundamental Lemma, proof 56
Fundamental theorem 47.
Fundamental Theorem, proof 58
Furter, J. 69 89—90 104 108 118 152.
G-orbit 60.
Gel'fand, I. 136
Generating function 11
Gibson, C 209.
Golubitsky, M. 3 20 34 63 69 89—91 93 214.
Gradient, bifurcation problem 33.
Gradient, higher-order terms 40
Group action 19.
Group orbit 135
Group, compact Lie 38 121
Group, Lie 60
Group, spatial 18.
GSS-II 35—37 40 48 51 56—57 61—62 75 121 126 132—133 213—214
Hamiltonian systems 3 120 130
Hessian matrix 15 21 23 154 166 212
Higher-order terms 39 48
Hilbert basis 20 137 144.
Howard, J. 28 186
Hummel, A. 3 34.
Ideal, instrinsic 48
Invariant circle 146.
Invariant function 137
Invariant function, ![$\mathbb{D}_{[p,q]}$](/math_tex/66e6dec63b460d206dbd907bcd45df0682.gif) 206 206
Invariant function, 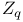 63 67 63 67
Involution 18.
Involution, anti-symplectic 101 197 209
Isotypic decomposition 121.
Katok, A. 2
Kielhoefer, H. 128
Kook, H. 2 24 26
Kozlov, V. 21.
Krawcewicz, W. 129.
Krein locus 171.
Lagrangian (continuous time) 141 142
Lagrangian generating function 1—2
Lahiri, A. 7 164
Lidskii, V. 136
Liftable vectorfields 53.
Looijenga, E. 45 58.
MacKay, R. 2 7 10 12 24 28 144 149 173—174 186 190
Magnus, R. 185
Manifold 59 60.
Manifold, analytic 44.
Manifold, solid torus 136.
| Manifold, submanifold 60
Marsden, J. 175
Marzantowicz, W. 129
Mather's Lemma 60—61
Mather, J. 3 60
Mawhin, J. 210 212
Meiss, J. 2 10 12 24 26 190 199 200
Melbourne, I. 175 202
Meyer, K. 1 2 6 10 20—21 87—88 103
Modal parameters 34
Mode interactions 10 205
Momentum map 194
Mond, D. 3 35 53 58
Montaldi, J. 3 5 35 40 120 128 143 202.
Morse index 19 127.
Moser, J. 10 120 130
Nakayama's lemma 73
Neishtadt, A. 21
Noether's theorem 5 194.
Noether's theorem, conserved quantities 5 136 141 194—196.
Non-degeneracy conditions 67—68 70 74 79 82
Normal form for bifurcation function 20 23 36 74 155 181.
Normal form, unipotent normal form 173.
Normal form, Williamson normal form 192
Normal space, extended 37.
O(2) 5.
O(2), equivariant syniplectic maps 132 143
O(2), invariant generating function 132
Orbit space 144.
Palais, R. 126
Path formulation 3 34 40—41
Percival, I. 2
Period-q point 13
Pfenniger, D. 7
Planar pendulum 142 143
Poisson bracket 145
Potential function 3 33—34
Potential function in  47. 47.
Pull-back mapping 49.
q-resonant subspace 119 123
Quaternionic algebra 123.
Rannou map 85.
Rannou, F. 85
Rational Collision Theorem 155 161
Recognition problem 40
Representation, absolutely irreducible 130
Residue 25 166—167
Resonance 10
Reversibility 196.
Reversibility, CS-reversibility 18 101 196 199.
Reversibility, k-reversibility 101.
Reversibility, R-reversibility 101 197 199
Reversible maps 18 22 196 209.
Reversible period-q points 22
Rimmer, R. 1 22.
Roberts, R. 5 40 42 63 69 89—91 93 120 128 143 202
Rotation number 6 9.
Roy, T. 164.
Scalar product, 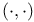 on on  11 11
Scalar product, 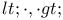 on on  38 204 38 204
Scalar product, 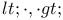 on on 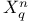 14 14
Scalar product, ![$[ \cdot, \cdot]$](/math_tex/d7bcfe0511636c9c7dd688f0fc0160dc82.gif) on on 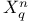 11 11
Schaeffer, D. 3 20 34
Schmidt, D. 6
Schoenberg, I. 88
Schwartz's Theorem 20
Sevryuk, M. 7
Signature 127 193.
Signature for equivariant maps 127 188.
Signature on configuration space 185—190
Signature, Krein's definition 186
Slodowy, P. 44—45.
SO(2)-symmetric rational collision 181
Sokol'skij, A. 6.
Spectrum, second variation of action 15 154
Spectrum, symplectic matrix 28.
Spherical pendulum 140—143
Stability for equivariant maps 146.
Stability, Instability Lemma 24 163 212.
Stability, linear, on configuration space 190—192
Stability, reduced 23.
Stability, Stability Lemma I 23 26.
Stability, Stability Lemma II 171
Standard map, generalized 85 101
Stark, J. 2 10 12.
Starzhinskii, V. 186.
Stewart, I 5 20 40 120 128 143 202
Stratification, Whitney 58
Subgroup, isotropy 134 207 213
Subgroup, isotropy, lattice 207—209.
Subgroup, normalizer 126.
Subgroup, r-spatial 126.
Subgroup, r-spatio-temporal 126.
Subgroup, twisted 126 213—214.
Subgroup, Weyl 126 181
Subharmonic bifurcation 121 130
Symmetry group (discrete) 18
Symmetry, of solutions 129 131.
Symmetry, spatial or temporal 18 126 128.
Symmetry, symmetric criticality 126.
Symplectic form 7 202.
Symplectic group 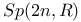 13 151 192—193 13 151 192—193
Symplectic Lie algebra 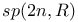 13 151 13 151
Symplectic operator 7 196 202
Symplectomorphism 3
Symplectomorphism, q-regular 123.
Tangent map 24 168
Tangent space 36 64.
Tangent, extended tangent space 50 65 73
Tangent, extended tangent space, gradient 38.
Tangent, restricted tangent space 37 50
Tensor product 14
Terao, H. 53.
Torus 143
Twist 199
Twist mapping 199.
Unfolding 37 39 44 52
Unfolding, universal 37 40 48 65.
Unfolding, universal, (gradient)  35 39 35 39
Unfolding, versal 37 47 150.
Universality Theorem 39
Universality Theorem, proof 51.
van der Meer, J. 6 175 182
Vanderbauwhede, A. 45.
Variational Principle 11.
Wall, C 42 48
Weinstein — Moser Theory 10 120 130.
Weinstein, A. 10 120 130
Willem, M. 210 212
Williamson, J. 193.
Yakubovich, V. 186
Yau, S. 42.
Zuppa, C. 34.
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 (cyclic group)
(cyclic group)  (dihedral group)
(dihedral group) 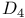 problems
problems 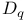 , problems
, problems 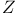 q problems
q problems 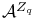 for potentials
for potentials 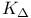
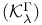
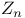
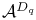

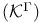
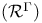
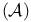
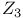 -invariant potentials
-invariant potentials 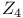 -invariant potentials
-invariant potentials 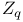 q-invariant potentials
q-invariant potentials ![$\mathbb{D}_{[p,q]}$](/math_tex/66e6dec63b460d206dbd907bcd45df0682.gif)

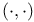 on
on 
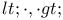 on
on 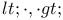 on
on 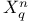
![$[ \cdot, \cdot]$](/math_tex/d7bcfe0511636c9c7dd688f0fc0160dc82.gif) on
on