|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Valls C., Barreira L. — Stability of Nonautonomous Differential Equations |
|
|
 |
| Предметный указатель |
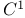 stable manifold 75 100 stable manifold 75 100
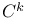 stable manifold 102 stable manifold 102
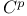 stable manifold 116 stable manifold 116
 57 57
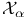 57 57
Admissibility 29
angle 24 252
Backward, filtration 245
Backward, Lyapunov exponent 245
Backward, regular point 245
Basis, dual 221 236 251 256
Basis, normal 236 256
Basis, normal, for a Lyapunov exponent 256
Center manifold 171 175
Center manifold, equivariance 197 214
Center manifold, existence 173
Center manifold, reversibility 197 202
Change of coordinates, Lyapunov 246
Characterization of regularity 235 256
Characterization, stable space 110
Coefficient, Perron 237 254
Coefficient, Perron, lower bound 259
Coefficient, Perron, upper bound 259
Coefficient, regularity 221 251 254
Coefficient, regularity, lower bound 259
Coefficient, regularity, upper bound 259
Cone family 86
Cone family, invariant 86
Conjugacy for flows 145
Conjugacy for maps 146
Conjugacy, Holder regularity 145 155
Dual basis 221 236 251 256
Dual normal basis 236 256
Equation, equivariant 213
Equation, Lyapunov regular 222
Equation, negative Lyapunov exponents 241
Equation, reversible 197 199
Equivariant, center manifold 197 214
Equivariant, equation 213
Equivariant, nonautonomous equation 213
Evolution operator 19
Existence of center manifolds 173
Existence of exponential dichotomies 24 222
Existence of Lipschitz stable manifolds 56
Existence of Lipschitz unstable manifolds 71
Existence of smooth stable manifolds 120
Existence of topological conjugacies 149
Exponential dichotomy 19 24 219
Exponential dichotomy for maps 147
Exponential dichotomy, basic properties 19
Exponential dichotomy, existence 24
Exponential dichotomy, nonuniform 19 222
Exponential dichotomy, robustness 27
Exponential dichotomy, uniform 19 20
Exponential trichotomy 172
Exponential trichotomy, nonuniform 172
Faa di Bruno formula 12 119 125 126
Filtration, backward 245
Filtration, forward 244
Flow, measure-preserving 244
Forward filtration 244
Forward Lyapunov exponent 244
Forward regular point 245
Grobman — Hartman theorem 145
Grobman — Hartman theorem, nonautonomous 145
Holder, conjugacy 145 155
Holder, derivatives 129
Hyperbolic solution 59
Hyperbolic trajectory 59 77 121
Inner product, Lyapunov 246
Invariant cone family 86
Linear extension of a vector field 107
Lipschitz, manifold 57
Lipschitz, stable manifold 55 58 60 242
Lipschitz, unstable manifold 71 73
Local stable manifold 58 60 77 78
Local unstable manifold 73
Lower bound Perron coefficient 259
Lower bound regularity coefficient 226
Lyapunov, change of coordinates 246
Lyapunov, exponent 25 219—221
Lyapunov, exponent, backward 245
| Lyapunov, exponent, forward 244
Lyapunov, exponent, negative 241
Lyapunov, exponent, normal basis 236
Lyapunov, inner product 246
Lyapunov, norm 83 156 246
Lyapunov, regular equation 222 251 265
Lyapunov, regular point 246
Lyapunov, regularity 219 249
Lyapunov, regularity in a Hilbert space 249
Manifold, center 171 175
Manifold, Lipschitz 57
Measure-preserving flow 244
Negative Lyapunov exponent 241
Nonautonomous equation, equivariance 213
Nonautonomous equation, reversibility 197
Nonautonomous equation, stability 265
Nonautonomous Grobman — Hartman theorem 145
Nonuniform exponential dichotomy 19
Nonuniform exponential dichotomy for maps 147 155
Nonuniform exponential dichotomy in J 20
Nonuniform exponential dichotomy, existence 222
Nonuniform exponential dichotomy, strong in J 20
Nonuniform exponential trichotomy 172
Nonuniformly hyperbolic solution 59
Nonuniformly hyperbolic trajectory 59 77 121
Nonuniformly partially hyperbolic solution 175
Norm, Lyapunov 83 156 246
Normal basis 236 256
Normal basis for a Lyapunov exponent 236 256
Perron coefficient 237 254
Reduction to a triangular matrix 232
Reduction upper triangular 252
Regular 246
Regular equation 222 251 265
Regular point 246
Regular point, backward 245
Regular point, forward 245
Regularity 219 249
Regularity, characterization 235 256
Regularity, coefficient 221 226 251 254
Regularity, coefficient, lower bound 226
Regularity, coefficient, upper bound 227
Regularity, Lyapunov 219 249
Reversibility 197
Reversibility for nonautonomous equation 197
Reversibility in center manifold 197 202
Reversible equation 197 199
Robustness 27
Smooth stable manifold 75 119 243
Smooth stable manifold in  75 75
Smooth stable manifold in a Banach space 119
Smooth stable manifold, existence 120
Solution, exponentially unstable 266
Space, stable 22 41 148
Space, unstable 22 41 148
Stability 267
Stability of a nonautonomous equation 265
Stable manifold 77 78
Stable manifold, 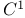 75 100 75 100
Stable manifold, Ck 102 116
Stable manifold, Lipschitz 55 56 58 60 242
Stable manifold, local 58 60 77 78
Stable manifold, smooth 75 119 243
Stable space 22 41 92 110 148
Stable space, characterization 110
Stable space, construction 92
Stable space, continuity 92
Strong exponential dichotomy for maps 155
Strong exponential dichotomy in J 20
Tangent set 95 96
Topological conjugacy 149
Triangular matrix 232
Triangular operator 252 265
Triangular reduction 232
Uniform exponential dichotomy 19 20
Unstable manifold, Lipschitz 71 73
Unstable manifold, local 73
Unstable solution 266
Unstable space 22 41 148
Upper triangular 252 265
Upper triangular, reduction 252
Vector field, linear extension 107
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



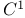 stable manifold
stable manifold 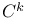 stable manifold
stable manifold 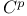 stable manifold
stable manifold