Авторизация
Поиск по указателям
F.Giannessi, F.Giannessi — Constrained Optimization and Image Space Analysis
Обсудите книгу на научном форуме Нашли опечатку?
Название: Constrained Optimization and Image Space AnalysisАвторы: F.Giannessi, F.Giannessi Аннотация: Over the last twenty years, Professor Franco Giannessi, a highly respected researcher, has been working on an approach to optimization theory based on image space analysis. His theory has been elaborated by many other researchers in a wealth of papers. Constrained Optimization and Image Space Analysis unites his results and presents optimization theory and variational inequalities in their light.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2005Количество страниц: 395Добавлена в каталог: 10.05.2008Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Admissible cone 67 Affine(ly), function 95 Affine(ly), hull 52 Affine(ly), independent 52 275 328 329 Affine(ly), multifunction 286 Affine(ly), set 52 Alexandroff A.D. 226 Alternative, strong 308 Alternative, weak 308 Altitude of a simplex 10 37 Analytical complexity 129 Antitone functions 100 Archimedes 125 Astrodynamics 23 27 Bad convex functions 131—132 Base of a simplex 37 Bellman R. 237 Bellman Recurrence Equation 237 Bernoulli Jakob 36 Bernoulli Johann 36 Bilateral constraint(s) 1 3 4 Bouligand’s contingent 126 Brachistochrone problem 36 Caratheodory theorem 50 328 Cauchy A.L. 226 228 Cavalieri B. F. 37 Centre of a set 127 128 Clarke derivative 153 156 157 Coerciveness 19 136 167 246 Combinatorial optimization 4 39 294 Comer point 57 Complementarity condition 316—317 322 330 Complementarity problem(s) 15 18 22 42 Complementarity systems 16 25 41 353 Complementarity vector system 17 Complex H-function 124 Complex minimum 8 Complex minimum point 8 Complex polar 77 Concave, set 47 Concave, strictly 48 Concavelike function 124 238 262 264 Cone(s) 58 Cone(s), acute 58 Cone(s), admissible 67 235 Cone(s), apex of 59 Cone(s), closed 4 Cone(s), convex 4 Cone(s), derived 235 Cone(s), differential 235 Cone(s), generated 61 Cone(s), hypertangent 68 Cone(s), interior 68 Cone(s), normal 68 114 Cone(s), pointed 4 58 86 Cone(s), polyhedral 61 Cone(s), properly pointed 58 59 90 126 Cone(s), reachable 67 235 Cone(s), right circular 6 Cone(s), solid 58 86 Cone(s), spanned 61 Cone(s), tangent 63 126 235 Conic extension 169 231 245 290 Conjugate function 116 117 Constrained problem(s) 2 4 Constraint(s), bilateral 1 3 4 Constraint(s), equilibrium 18 Constraint(s), unilateral 1 3 4 Contingent 126 Continuous Optimization 40 345 Convex, bad functions 131—132 Convex, body 47 Convex, closure 55 Convex, combination 48 Convex, cone 58 Convex, function 95 Convex, geodesic hull 293 Convex, hull 48 293 Convex, multifunction 286 Convex, proper combination 48 Convex, set 47 Convex, strictly (set) 47 Courant R. 308 Cutting halfspace 369 Cycloid 36 deFermat P. 10 37 Derivative(s), Clarke 153 Derivative(s), Dini directional 153 Derivative(s), directional 143 330 Derivative(s), Frechet 191 Derivative(s), G- 143 Derivative(s), G-semi 150 225 Derivative(s), Gateaux 191 Derivative(s), partial G- 143 Descent method 36 Dido’s problem 10 35 Differentiable functions 110 Differentiable functions, 144 152 Differentiable functions, 144 Differentiable functions, semi- 150 158 Differential, G- 148 Differential, G-sub 147 Differential, G-super 148 Differential, sub- 110 Dimension of a face 73 Dini, directional derivatives 150 Dini, Theorem 321 Discrete optimization 4 294 301 345 Duality 308 Equilibrium, constraints 18 Equilibrium, flows 28 29 Erdos P. 10 37 Erdos problem 10 37 Euler L. 10 36 127 307 363 Euler, equation 307 332 Excess function 98 Extreme point 48 Face of a set 73 127 Face of a set, dimension of 73 Face of a set, exposed 73 Face of a set, improper 73 Face of a set, minimal 73 Face of a set, proper 73 Fan 287 Farkas J. 78 251 270 284 294 302 Fasbender E. 37 Feasible region 1 2 Feasible solution 2 Fenchel inequality 116 Fermat — Torricelli problem 10 37 Fixed-charge 40 Flight mechanics 23 26 Function(s), 144 Function(s), (non)decreasing 100 Function(s), (non)increasing 100 Function(s), affine 95 Function(s), antitone 100 Function(s), bad convex 131—132 Function(s), C-differentiable 144 152 Function(s), coercive 19 136 Function(s), complex H- 124 Function(s), concave 95 Function(s), concavelike 124 238 262 Function(s), conjugate 116 117 Function(s), convex 95 Function(s), convexlike 124 Function(s), differentiable 110 Function(s), directionally derivable 143 Function(s), excess 98 Function(s), G-derivable 143 Function(s), G-differentiable 143 Function(s), G-semidifferentiable 150 Function(s), gauge 115—116 Function(s), geodesic convex 124 Function(s), Green 25 Function(s), H- 124 Function(s), H-concavelike 124 262 Function(s), H-convex 124 136 Function(s), H-convexlike 124 136 Function(s), Hamiltonian 366 Function(s), indicator 114 115 117 Function(s), isotone 100 Function(s), Lagrangian 192—194 198 312 313 316 322 331 366 Function(s), likelihood 31 Function(s), max- 7 116 Function(s), Minkowski 115—116 Function(s), monotone 100 Function(s), multi- 15 180 354 Function(s), norm 114 Function(s), objective 2 Function(s), optimal value 167 Function(s), parabolic exponential 258 313 Function(s), Peano 122 136 Function(s), perturbation 167 Function(s), positively homogeneous 107 108 116 143 225 Function(s), pseudoantitone 122 Function(s), pseudoconcave 120 Function(s), pseudoconvex 120 135 Function(s), pseudoisotone 122 Function(s), quasiconcave 117—118 Function(s), quasiconvex 117—118 133 Function(s), selection 181 279 282 295 Function(s), semidifferentiable 150 158 224 Function(s), separation 252 254 258 259 290 Function(s), stricly quasiconcave 117—118 Function(s), strictly antitone 100 Function(s), strictly concave 95 Function(s), strictly convex 95 Function(s), strictly isotone 100 Function(s), strictly pseudoconcave 120 Function(s), strictly pseudoconvex 120 Function(s), strictly quasiconvex 117—118 Function(s), strong separation 255 289 Function(s), strongly convex 114 Function(s), subadditive 107 Function(s), subdifferentiable 110 Function(s), sublinear 107 143 Function(s), sum-decomposable 293 Function(s), superlinear 107 Function(s), support 76 116 117 Function(s), weak separation 252 254 258 282 290 299 310 312 Galilei G. 36 Gauge function 115—116 Generalized selective functions 279 282 295 Generalized systems 15 18 Generalized systems, parametric 18 Geodesic, convex functions 124 Geodesic, convex hull 293 Geodesic, set 38 Geodesic-type problem 3 4 Global minimum point 1 Global vector minimum point 5 Gozzi L. 39 Gradient, G-sub 148 Gradient, G-super 148 Gradient, semi- 224 Gradient, sub- 110 Graves L.M. 15 H-convex function 124 H-function 124 Hahn — Banach theorem 70—71 251 Hamiltonian function 366 Hartman — Stampacchia Theorem 19 Helly theorem 51 278 Hestenes M.R. 235 Hilder condition 347 Holomorphic field 135 Homogenization Lemma 172 195 197 235 328 Homogenization of the image 169 239 241 245 321—322 327—328 Hypertangent cone 68 Image 165 180 285 Image, conic extension of 169 Image, homogenization of 169 Image, perturbed problem 165 Image, selected 187 285 364 Image, space 165 180 Incidence-matrix 29 Indicator function 114 115 Inequality, Fenchel 116 Inequality, isoperimetrical 48 335 337 339 366 Inequality, Jensen 98 Inequality, Jung 53 Inequality, Minty Vector Variational 16 Inequality, Stampacchia Vector Variational 16 Inequality, Variational 15 16 42 242 353 372 Inequality, Vector Variational 16 Interior, cone 68 Interior, quasi relative 61 Isodiametrical problems 38 Isoperimetric(al) inequality 48 335 337 339 366 Isoperimetric(al) problems 10 36 38 334 Isoperimetric(al)-type problems 2 4 Isoproblems 38 Isotone, function 100 Isotone, gradient 114 Jensen inequality 98 John F. 321 Jordan — Brower Theorem 294 Jung Inequality 53 Lagrange G.L. 10 36 307 308 316 320 321—322 329 363 Lagrange G.L., auxiliary function 366 Lagrange G.L., Method of Multipliers 307 308 320 321—322 329 Lagrange G.L., necessary conditions 308 320 331—332 Lagrange G.L., principle 363 Lavel set 252 Lemma, Farkas 251 270 284 294 Lemma, Homogenization 172 195 197 235 328 Lemma, Linearization 172 Lemma, Minty 16 Lemma, Neyman — Pearson 32 Likelihood function 31 Linearization Lemma 172 Ljusternik Theorem 321 Local minimum point 1 Lower, semistationary point 1 Maier G. 19 22 Malfatti G. 38 39 Malfatti problem 38—39 Map(ping)s see “Functions” Matrix, incidence 29 Max-function 7 116 Maximum principle 364 Maximum Principle, Bellman 237 Maximum Principle, Dubovitskii — Milyutin 130 240 Maxmin problems 7 Mayer’s problem 14 Mean arithmetic 336 Mean geometric 336 Mean harmonic 336 Mengoli P. 226 Mersenne M. 10 Method(s), decomposition 244 Method(s), descent 36 Miele A. 26 28 Minimax problems 7 13 38 Minimum point 1 Minimum point, complex 8 Minimum point, global 1 Minimum point, global vector 5 Minimum point, isolated 1
Реклама
 |
|
О проекте
|
|
О проекте




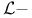
 -differentiable
-differentiable