|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Dehn E. — Algebraic Equations |
|
|
 |
| Предметный указатель |
Highest function 41
Highest term 41
Holder, theorem of 113
Identical element 127
Identical permutation, or identity 17 24 26 119 144
Identity (group) 29 32 37 115 120 121 141 143 144
Imprimitive domain 136 162 163
Imprimitive equation 162
Imprimitive group 105 106 162
Imprimitive number 134
Imprimitive system 105 106
Independent permutations 38 57
Independent transpositions 38
Index of group 31 33
Integral function 1 2 10 132 142
Integral function, continuity of 9
Integral functions, division of 3
Integral functions, quotient of 4
Interpolation 2
Interpolation formula of Lagrange 2 122
Intransitive group 101 102 157
Intransitive subgroup 105
Intransitive system 102 106 157
Intransitive system, degree of 102
Invariant domain 136
Invariant equation 136
Invariant subgroup 36
Inverse of element 127
Inverse of permutation 24 25 34
Inverse, transform of 34
Involution on permutation 21
Irrationality, natural 169 170
Irreducible but solvable equation 190 191 192 193
Irreducible equation 131 134 135 136 158 171 172 182 190 191 192 193
Irreducible factor 6 156 157
Irreducible function 6 8 130 131 134 143
Isomorphic groups 99 100 127
Isomorphic groups, n to 1 100
Isomorphic groups, simply 99 146
Jerrard 196
Jordan — Holder, theorem of 113
kronecker 169
Lagrange 1 11 31 67 81 155 156 195
Lagrange — Galois, theorem of 151 156
Lagrange, generalized theorem of 151
Lagrange, interpolation formula of 2 122
Lagrange’s plan 74 75 81 139 140 149 152 154 155
Lagrange’s solvent (resolvent) 78 80
Lagrange’s Theorem 67 73 139 142 151 156
Law of combination 19 126
Law, associative 23 126
Law, commutative 20
Linear group 121
Maximum normal subgroup 111 112
Metacyclic equation 115 190 195
Metacyclic group 115 121 123 124 125 126 190 198
Metacyclic group in 5 letters 125
Multiple (double) root 10 51 131 141 171 193
Multiplication of groups 107
Multiplication of permutations 19
Multiplication table of elements 127
n to 1 isomorphic groups 100
Natural irrationality 169 170
Non-circular permutation 21 56
Non-circular permutation, order of 22
Non-primitive group 105
Normal domain 136 144 165
Normal domain, substitution group of 188 146
Normal domain, substitution of 137 138
Normal equation 136 144 158 169 171 173 182
Normal equation of prime degree 173
Normal permutation 115 116
Normal subgroup 86 37 53 58 61 64 76 78 80 100 105 110 111 116 121 123 126
Normal subgroup, maximum 111 112
Normal subgroups of alternating group 61
Normal subgroups of symmetric group 61
normalizer 119
Notation, circular 18
Number belonging to exponent 130 180
Number in algebraic domain 130 133 135
Number of Fermat 190
Number one 17 29 129
Number, imprimitive 134
Number, primitive 134 135
Number, rational 129
Number. 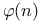 177 182 177 182
Numbers, conjugate 183 134
Odd permutation 56
Odd prime 180
Operations, algebraic 1 80 152 170 183
Operations, rational 1 4 5 129 183
Order of circular permutation 22
Order of cycle 21
Order of group 29 31 32 33 107 108 127
Order of non-circular permutation 22
Order of permutation 21 22 23 32
Order of transitive group 102
Order, absolute 117
Order, off actor-group 110
Order, prime 23 32 119
Order, relative 116
Ordinary resolvent 151 167
Partial resolvent 168
Partition of group 30
Period of cycle 21
Period of permutation 21
Permutable 21
Permutation 17 38
Permutation, absolute order of 117
Permutation, circular 18 21 56 58 101 123
Permutation, cycle of 18 38 56
Permutation, degree of 18 23 56
Permutation, even 56 57 126
Permutation, generating 38
Permutation, identical 17 24 26 119 144
Permutation, inverse of 24 25 34
Permutation, involution on 21
Permutation, non-circular 21 56
Permutation, normal 115 116
Permutation, odd 56
Permutation, order of 21 22 23 32
Permutation, period of 21
Permutation, power of 21 34
Permutation, regular 19
Permutation, relative order of 116
Permutation, transform of 33 34 35
Permutationgroup 27 29 127
Permutationof prime degree and order 23
Permutationof prime order 23 119
Permutations between conjugate functions 64
Permutations, class of 118 119
Permutations, commutative 20 21 23 24 37 115 119
Permutations, conjugate 33
Permutations, division of 25
Permutations, group of 27 29 127
Permutations, independent 38 57
Permutations, multiplication of 19
Permutations, product of 19 34
Permutations, similar 19 24 35 36
Plan of Galois 140 149 152 155 166 168
Plan of Lagrange 74 75 81 139 140 149 152 154 155
Polygon, regular 187 189
Polynomial 2
Power of permutation 21 34
Power, transform of 34
Primary Galoisian function 143 414 146 149
Prime degree and order of group 32 123
Prime degree and order of permutation 23
Prime degree of equation 173 190 191 192 193 195
Prime degree of group 120 121 123 124 125
| Prime number, odd 180
Prime number, primitive root of 180 182 185
Prime order of group 32
Prime order of permutation 23 119
Prime root of unity 12 76 80 152 170 175 176 182 183 187
Primitive domain 136 162
Primitive function 7
Primitive group 105 162
Primitive number 134 135
Primitive root of prime number 180 182 185
Primitive root of unity 12 76 80 152 170 175 176
Product of elements 127
Product of functions 7 10
Product of groups 107
Product of groups, direct 108 118
Product of permutations 19 34
Product of permutations, transform of 34
Property (1) of Galoisian group 146
Property (2) of Galoisian group 148
Quadratic equation 51 82
Quadratic group 61
Quadratic, discriminant of 51
Quadratic, solution of 83
Quintic equation 195 200
Quintic equation, Bring — Jerrard normal form of 195 200
Quintic equation, discriminant of 199
Quintic equation, resolvent sextic of 195 199 200
Quintic equation, solvable 200
Quotient of groups 109 110
Quotient of integral functions 4
Quotient, abstract 109
Rational equation 131 148
Rational function 1 2 67 129 131 132 142 143 146 147 148 149 150 151 154 155 156 164
Rational in domain 129
Rational numbers, domain of 129 182 195
Rational operation 1 4 5 129 183
Rational root 193
Rational value 146 147 148
Rationally known 130
Reducible equation 157
Reducible function 6 130
Reduction of group 164 170
Regular group 103 158 182
Regular permutation 19
Regular polygon 187 189
Relative order 116
Remainder 4
Resolvent (equation) 66 150 151 154 156 167 168 183
Resolvent for group 67
Resolvent of composition 168 169 173
Resolvent of Galois 148 144 145 149 151 166 167 169
Resolvent of Lagrange 78
Resolvent, binomial 76 78
Resolvent, general 193
Resolvent, group of 67 167
Resolvent, ordinary 151 167
Resolvent, partial 168
Resolvent, sextic 195 199 200
Resolvent, total 168
Resultant 46 47 50
Root of congruence 178
Root of discriminant 52 55 56
Root of prime number 180 182 185
Root of unity 12 76 80 152 170 175 176 182 183 187
Root, algebraic 184
Root, common 47
Root, double (multiple) 10 51 131 141 171 193
Root, extraction of 1
Root, rational 193
Roots of cyclic equation 174
Roots of cyclotomic equation 189
Roots, cycle of 173 174
Rule of transforms 35
Self-conjugate subgroup 36
Set, transitive 102
Similar permutations 19 24 35 36
Simple group 37 61
Simply isomorphic groups 99 146
Simply transitive group 104
Soluble group 115 116 118 120 124 169 173
Solution of biquadratic 75 97
Solution of cubic 11 13 89
Solution of equation 1 (see also “Solvable equation”)
Solution, algebraic 1
Solutionof quadratic 83
Solutionof quintic 200
Solvable equation 80 152 170 183 191 192 193 195 200
Solvable quintic 200
Solvent of Lagrange 78 80
Special equation 139 149 152 155 156
Special equation, group of 155
Subdomain 131
Subgroup 29 61 62 110
Subgroup, central 115 116
Subgroup, greatest common 53 64 110
Subgroup, intransitive 105
Subgroup, invariant 36
Subgroup, maximum normal 111 112
Subgroup, normal 86 37 53 58 61 64 76 78 80 100 105 110 111 116 121 123 126
Subgroup, self-conjugate 36
Subgroups of alternating group 62
Subgroups of normal domain 137 138
Subgroups of quotient-group 111
Subgroups of symmetric group 61
Subgroups, conjugate 33 34 99 103 150 155
Subgroups, group of normal domain 138 146
Substitution 17 187
Sum of conjugate values 73\
Sum, symmetric 40 41
Symmetric function 15 40 43 154
Symmetric function of conjugate values 72 73
Symmetric function, elementary 41 43
Symmetric functions, fundamental theorem of 43
Symmetric group 29 38 61 120 154 155 156
Symmetric group in 2 letters 39
Symmetric group in 3 letters 39
Symmetric group in 4 letters 39
Symmetric group, composition-series of 61 120
Symmetric group, normal subgroups of 61
Symmetric group, subgroups of 61
Symmetric sum 40 41
System, imprimitive 105 106
System, intransitive 102 106 157
System, transitive 102
Term, highest 41
Theorem of algebra, fundamental 10 184
Theorem of Cauchy 119
Theorem of Cayley 127
Theorem of Eisenstein 8
Theorem of Fermat 179
Theorem of Galois 190
Theorem of Jordan — Holder 113
Theorem of Lagrange 67 73 139 142 151 156
Theorem of Lagrange generalized 151
Theorem of Lagrange — Galois 151 156
Theorem of symmetric functions, fundamental 43
Theory of groups 27 126 127
Total degree 45
Total resolvent 168
Transform of group 33 99
Transform of inverse 34
Transform of permutation 33 34 35
Transform of power 34
Transform of product 34
Transformation, Tschirnhaus 196
Transforms, rule of 35
Transitive composition-series 121
Transitive group 101 102 103 104 105 120 121 124 156 158 173 190
Transitive group, composition-series of 121
Transitive group, doubly 104
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте