Hobson E.W. — The Theory of Functions of a Real Variable and the Theory of Fourier's Series. Vol. II :: Электронная библиотека попечительского совета мехмата МГУ

Главная Ex Libris Книги Журналы Статьи Серии Каталог Wanted Загрузка ХудЛит Справка Поиск по индексам Поиск Форум

Авторизация

Поиск по указателям

Hobson E.W. — The Theory of Functions of a Real Variable and the Theory of Fourier's Series. Vol. II

Обсудите книгу на научном форуме

Нашли опечатку?
Выделите ее мышкой и нажмите Ctrl+Enter

Название: The Theory of Functions of a Real Variable and the Theory of Fourier's Series. Vol. II

Автор: Hobson E.W.

Язык:

Рубрика: Математика/

Статус предметного указателя: Готов указатель с номерами страниц

ed2k: ed2k stats

Издание: Second Edition

Год издания: 1926

Количество страниц: 780

Добавлена в каталог: 17.04.2008

Операции: Положить на полку | Скопировать ссылку для форума | Скопировать ID

Предметный указатель

Abel      6 11 14 22 34 57 117 175 178
Abel’s lemma      34
Abel’s theorem      175
Abel’s theorem for power-series      175
Abel’s Theorem, converse of      182
Absolute convergence of double series and sequences      52
Absolute convergence of trigonometrical series      548
Absolutely convergent series of numbers      36
Alexandroff      384 676
Anderson      70 76 85
Appell      180
Application of second mean value theorem to Fourier’s series      509
Applications of singular integrals      459
Approach, uniform      108
Approximate representation of functions by trigonometrical series      636
Approximately continuous functions, standard sets of      257
Arithmetic means related to Fourier’s series      557 561 563 587
Arzel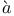 $\grave{a}$       107 118 132 168 293 306 314 659 698
B $\hat{o}$ cher      119 494 498 642
Bachmann      7
Baire      137 148 150 264 276
Baire’s classification of functions      276
Baker      498
Banach      755
Bary      678
Bauer      384
Bendixson      306
Bernoulli, D.      477 478 479
Bernstein      551
Bertrand      22 25 29
Besikovitch      697
bessel      482 554
Birkhoff      116
Bolzano      5 402
Bonnet      22 29 510
Borel      69 234 265 643
Brod $\acute{e}$ n      401 539
Bromwich      8 55 69 70 85 181 227 330 351 387 404 471
Brouwer      154
Burkhardt      6 476 478
Cajori      58
Cantor      G. 120 399 658 660 674
Cantor’s method of condensation of singularities      399
Carath $\acute{e}$ odory      150 265 489
Carleman      590 600
Catalan      34
Cauchy      6 16 22 24 25 57 64 200 210 289 481
Cauchy-product of series of numbers      56 75
Cell $\acute{e}$ rier      402
Cerni      698
Ces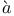 $\grave{a}$ ro      41 66 182
Ces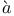 $\grave{a}$ ro sum of series of numbers      41
Ces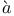 $\grave{a}$ ro’s summation of series      27 47 85
Cesaro summation of divergence      715
Chapman      69 70 73 75 81 85 567
Classes of monotone sequences      157
Complete sequence of normal orthogonal functions      753
Completely integrable sequences      289
Condensation, Cauchy’s test      17
Condensation, Cauchy’s test, of singularities      389
Condition of convergence of series of numbers      1 5
Continuous functions, standard sets of      238
Continuous oscillation of sequences      163
Convergence at a point of trigonometrical series      672
Convergence by segments      132
Convergence of a sequence in a measurable domain      144
Convergence of divergence      705
Convergence of Fourier’s series      491 521
Convergence of monotone sequences      274
Convergence of numerical series in general      34
Convergence of series of normal orthogonal functions      755 762
Convergence of singular integrals      446
Convergence, of sequences on the average      239
Convergence, of series with positive terms      9
Convergence-factors for Fourier’s series      623
Convergence-function      135
Criteria of convergence      15
Cross-neighbourhood      445
Dantscher      214
de la Vall $\acute{e}$ e Poussin’s test for convergence of Fourier’s series      531
Dedekind      34
Dell’ Agnola      137 265
Den joy      257
Diagonal sum of double series and sequences      54
Differentiable everywhere-oscillating functions      412
Differentiation and integration of power-series      196
Differentiation of Fourier’s series      639
Differentiation of series      332 335
Dini      14 15 24 26 105 125 337 389 399 402 412 422 510 526 528 659
Dircksen      482
Dirichlet      117 482 493 507
Dirichlet’s conditions      507
Dirichlet’s investigation of Fourier’s series      502
Distribution of points of non-uniform convergence      135
Divergence, of series with positive terms      9
Double Fourier’s series, divergence      698
Double limits      46
Double series and sequences      45
du Bois-Reymond      3 11 15 111 210 224 344 386 402 412 422 497 539 647 659
Duhamel      29
D’Alembert      476 477
Egoroff      145
Egoroff’s theorem      144
Equi-continuous functions      167
Ermakoff      33
Essentially uniform convergence      145
Euler      477 478 479
Extension of functions      154
Extensions of Abel’s theorem for power-series      178
Extensions of Parseval’s theorem      578 591 599
F. Riesz’ classification of summable functions      249
Faber      402 411
Failure of convergence of series of normal orthogonal functions      755
Failure of convergence of singular integrals      456
Falanga      402 407
Fatou      539 548 552 576 631 632 634 635 643 644 697
Fej $\acute{e}$ r      225 498 540 541 559
Fischer      240 577
Ford      67
Formal multiplication of trigonometrical series      585
FOURIER      480 481 494
Fourier transforms      742
Fourier’s repeated integral      725
Fourier’s series, Formal expression of      482
Fourier’s single integral      721
Fr $\acute{e}$ chet      146
Frobenius      178
Fubini      335
Functions involving a parameter      141
Functions of bounded variation      702
Functions represented by sequences of continuous functions      185 270 273
Functions, defined by sequences or series      61
Gauss      30
Geiringer      698 704 717
General convergence theorem      422
General convergence theorem for non-summable functions      435
General definition of Fourier’s series      487
General property of sequences      7
Generalization of Riesz — Fischer theorem      599
Generalized integrals      363 371
Generalized second derivatives      664
Genocehi      644
Gibbs      498
Gibbs’ phenomenon      498
Gibson      478
Gronwall      498 541
Gross      717
H $\ddot{o}$ lder      66 539 631 659 667
Haar      540 757 772
hadamard      12 15

Hahn      67 109 112 125 139 150 152 160 167 282 422 570
Hake      384
Hamack      353 360 639 659
Hancock      214
hankel      389 412
Hardy      43 54 55 56 66 68 69 81 117 130 181 185 227 326 353 360 387 404 533 534 539 552 563 567 570 583 588 598 600 624 635 644 678 697 698 712 722 734 735
Hausdorff      150 152 280 600 676
Heine      656 657
hilbert      56
History of theory of trigonometrical series      480 656
History of theory of uniform convergence      130
Hobson      4 107 125 132 133 137 233 293 314 342 422 460 523 535 545 573 724 763 767 771 772
Holder’s summation of series      66 85
Homogeneous oscillation of sequences      169
Hurwitz      491 576 586
Infinite measure of non-uniform convergence      137
Infinite products, convergence of      58
Ingham      185
Integrable sequences      289
Integrable sequences of continuous functions      312
Integrable sequences of functions that are integrable (R)      312
Integrals containing parameters, limits of      322
Integrals of products, convergence of      464
Integrals, differentiation of      353
Integration of divergence      712
Integration of Fourier’s series      551
Integration of series      289 303
Integration, generalized      363 371
Inversion of order of repeated integrals over infinite domains      344
Ja $\check{s}$ ek      402
Jackson, Dunham      234 498
JORDAN      52 341 353 510 522
K $\ddot{o}$ pcke      412
K $\ddot{u}$ stermann      698 704 711 717
Kaczmarz      768
Kempisty      257
Kneser      494
Knopp      67 70 73 75 87 89 403 407
Kogbetliantz      653
Kohn      33
Kolmogoroff      539 614 626
Krause      698
kronecker      539 658
Kummer      28
Lagrange      200 478
Landau      6 84 179 185 386 460
Laskey      181
Lebesgue      230 234 264 265 270 277 278 282 289 422 438 454 459 469 487 514 528 538 540 561 563 576 586 663 667 675
Lebesgue’s constants      541
Lerch      230 643
Liapounoff      576
Limiting values of Fourier’s coefficients      514
Limits of coefficients of trigonometrical series      659
Liouville      771
Lipschitz      520 528
Littlewood      23 69 181 184 185 539 552 563 570 588 598 600 635 644 678 697
London      46
Looman      384 757
Love      498
Lusin      284 539 549 550 697
M. Riesz’ extension of Parseval’s theorem      610
Maxima and minima of functions of two variables      213
Maxima and minima, of functions of one variable      151
Maximal and minimal functions      102
Measure of non-uniform convergence      133
Menchoff      678 763 767 768
Mercer      87 772
Mertens      57
Method of monotone sequences      374
Michelson      498
Mittag-Leffler      230
Moigno      289
Mono-tonoid functions      703
Monotone sequences associated with a given sequence      159
Monotone sequences, of functions      148
Monotonoid functions      703
Moore, C. N.      70 227 387 698 717
Moore, E. H.      403
More slow convergence      10
Multiplication of power-series      194
Necessity of conditions in the general convergence theorem      438
Neder      490 655
Neumann, C.      510
Newman      257
Non-absolute convergence of double series and sequences      54
Non-convergence of Fourier’s series      539
Non-convergent series of numbers      2
Non-differentiable functions, construction of      389
Non-uniform convergence, points of      109
Order of Fourier’s coefficients      518 538
Oscillation of sequences of integrals      318
Oscillation of singular integrals      456
Osgood      119 120 121 135 137 293 306
Ottolenghi      73
O—o notation      6
PAL      154
Parseval      575
Parseval’s and Riesz — Fischer theorems for normal orthogonal functions      759
Parseval’s theorem      575
Parseval’s theorem for divergence      718
Parseval’s theorem for double series      718
Partial sums of Fourier’s series      489
Paucker      25
Peak and chasm functions      99
Pereno      412
Perron      489
Perron’s definition of integration      382
Picard      230 637 698
Plancherel      742 752 763
Plessner      626 632 696 697
Poincar $\acute{e}$       498
Points of non-uniform convergence      109
Points of simply uniform convergence      112
poisson      481 629
Poisson summation of divergence      717
Poisson’s summation      629
Poisson’s summation for double series      717
Poisson’s summation of Fourier’s series      629
Pollard      577
Pompeiu      401
Power-series      172
Primitives of functions      284
Pringsheim      8 11 16 20 22 25 26 34 46 54 59 64 111 130 694 722 724
Priwaloff      573 656
Properties of Fourier’s coefficients      573
Properties of measurable functions      178 282
Properties of power-series      192
Raabe      29
Radmacher      763
Rajchman      335 587 647 663 677 678 682
Reiff      6 130
Remainder in Taylor’s theorem      200
Remainder of series of numbers      1
Repeated integrals, inversion of order of      338
Restricted Fourier’s series      686
Riemann      38 514 522 645
Riemann’s summation of Fourier’s series      645
Riemann’s theory of trigonometrical series      645
Riesz — Fischer theorem      577
Riesz, F.      240 249 516 577 600 608 762
Riesz, M.      68 85 90 567 611 612 635 659 682 698
Roche      200
Runge      230
Rychlik      402
Sachs      478 545
Sales      335
Scheeffer      213 214 220
Schl $\ddot{a}$ fli      497
Schl $\ddot{o}$ milch      31 200 643
Schnee      67

1 2

О проекте