|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Rogers C.A. — Hausdorff Measures |
|
|
 |
| Предметный указатель |
Abstract space 2
Analytic set 67
Approximation from within 25 35 97 99 100 119 121 122
Approximation from without 24 34
Approximation of  -sets by -sets by 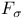 -sets 100 -sets 100
Approximation, rational 133 139—140
Auxiliary measure 109 112
Baire category 74—77
Baire category theorem 75—77
Base for topological space 61 68
Blaschke's selection theorem 91—94 125
Bordered sets 125
Borel sets 22 33 167
Brownian motion 133
Cantor ternary set 67 73
Cantor — Bendixson Theorem 61—63 125
Capacity 133
Caratheodory outer measure 1 128
Caratheodory's lemma 30—32
Cartesian products sets 130—131
Category theorem, Baire 75—77
Category, Baire 74—77
Closed sets 22
Closed sets, space of 90—93 125
Compact sets 60 63 67 68 97 112 119 121 122 124 127
Comparison of measures 75—84
Complete metric space 62
Concentrated sets 74 77
Condensation, point of 61
Construction of measure 20 21
Construction of measure, by Method I 9
Construction of measure, by Method I,  -regularity 24 -regularity 24
Construction of measure, by Method I, ubiquity of 11
Construction of measure, by Method II 27
Construction of measure, by Method II, 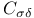 -regularity 34 -regularity 34
Construction of measure, by Method II, metric property 30
Continued fractions 133 135—147
Continued fractions, properties of 136
Continuity, absolute 134 148 166
Continuum Hypothesis 74
Convergence, strong 100 110 121
Countably additive measure 2 8 12
Counting measure 2 84
CURVES 132
Cylinder sets 130—131
Decimal expansion 132
Dense sets 61
density 129 130
Derivative, upper h-derivative 151
Diameter 27 50
Difference, set theoretic 3
Dimension, generalized 78
Dimension, topological 132
Distance sets 131
Distance, between sets 90
Distance, separating sets 28 125
Egoroff's theorem 39
Euclidean space, Lebesgue measure in n-dimensional 40—43
Euclidean space, net measure in 102—103
Existence theorems, for Hausdorff measures 58—77
Finite dimensional space, net measure in 104 122
Finite positive measure, sets of 58 63 67 68 102 119 121 122 132
Fractions, continued 133 135—147
Functions 134—135 147—168
Functions, h-continuous and strongly h-continuous 149 159—168
Functions, non-decreasing continuous 147—148
Functions, partial order of 78
H-continuity and strong h-continuity 149 160—168
Hausdorff measures 50—127
Hausdorff measures, applications of 128—168
Hausdorff measures, definitions of 50—51
Hilbert space 134
Increasing sets lemma 90—101
Information theory 132
Intersection of sets 131—132
Irregular sets 129
Jarnik's theorems 135—147
Lebesgue measure 40—43
Lemma, Caratheodory's 30—32
Lemma, increasing sets 90—101 107
Lemma, increasing sets, for 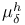 measure 97 measure 97
Lemma, increasing sets, for net measure 107
Line segments 134
Lines, assigning of measures to sets of 132
Lipschitz condition 149 159—165
Mapping theorem 53—54
Mappings and Hausdorff measures 53
Measurability 3
| Measurability of Souslin sets 48
Measurability, criterion for 4 19
Measurable sets 3 4 15 19 20 32 33 39 45—49
Measure see also "Auxiliary measure" "Hausdorff "Lebesgue "Metric "Net "Non-sigma-finite "Outer "Positive "Pre-measure" "Radon "Regular "Sigma-finite "Zero
Measure in topological space 22—26 43
Measure, comparison of 78—84
Measure, construction of 20 21
Measure, construction of, by Method I 9 11 24
Measure, construction of, by Method II 27 30 34
Measure, examples of 2 3 14 68
Measure, partial order of 22
Measureless sets 132
Metric measures 30—33
Metric measures, in topological space 43
Metric space, complete 62
Metric space, measures in 26—40
Metric space, separable 63
Metric, definition of a 26
Net measures 101—122
Net, definition of 101
Non-sigma-finite measure, sets of 78 83 123—127
Non-sigma-finite measure, subsets of 100 127
Nowhere-dense sets 74
Null sets 4 8 59 79 81
Open sets 22
Open sets, assigning positive measure to 68
Order, partial of functions 78
Order, partial of measures 22
Outer measure 1 128
Packing 134
Partial order see "Order"
Perfect sets 61
Point of condensation 61
Point, isolated 74
POLYGONS 134
Positive measure, assigning of, to open sets 68
Positive measure, sets of 63 97 100
Positive measure, subsets of 97 99 124
Pre-measure 9
Projection properties 129 130
Radon measure 1 148 150—151
Radon — Nikodym theorem 150 166
Rational approximation 133 139—140
Real numbers, sets of, in terms of expansions into continued fractions 135—147
Regular measure 13 17 19
Regular measure, 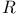 -regular measure 23 -regular measure 23
Regular sets 129
Separable metric space 63
Separation of sets 4
Separation of sets, positive 28
Sets see also "Analytic" "Bordered" "Borel" "Cantor "Cartesian "Closed" "Compact" "Concentrated" "Cylinder" "Dense" "Distance" "Irregular" "Lines" "Measurable" "Measureless" "Nowhere-dense" "Null" "Open" "Perfect" "Real "Regular" "Souslin" "Uncountable" "Zero "Finite "Non-sigma-finite" "Positive" "Sigma-field" "Sigma-finite"
Sets, 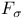 -sets 35 40 50 101 -sets 35 40 50 101
Sets,  -sets 34 35 40 50 -sets 34 35 40 50
Sets, category of 74 11
Sets, countably additive measure on  -field of 8—9 -field of 8—9
Sets, decreasing sequence of 16 18 39 100 112 119
Sets, distance between 90
Sets, increasing sequence of 15 17 31 90 97 100 107
Sets, intersection properties of 131—132
Sets, separation of 4
Sets, separation of, positive 28
Sigma-compact 99
Sigma-field 5
Sigma-field of sets, countably additive measure defined on 8
Sigma-finite measure, sets of 78
Souslin operation 44—49
Souslin set 84—90 97 99 102 119 121 124
Souslin set, measurability of 48
Space see also "Metric space" "Topological "Ultra-metric
Space of closed sets 90—93 125
Space, abstract 2
Space, finite dimensional 104 122
Space, Hilbert 134
Subsets of finite positive measure 122
Subsets of non-sigma-finite measure 100 127
Subsets of positive measure 97 99 124
Surface area 53—58 128
Tangential properties 129 130
Topological space, base for 61 68
Topological space, measures in 22—26
Topological space, metric measures in 43
Ultra-metric space 104—107 122
Ultra-metric space, net measure in 104—107 122
Uncountable sets 67
Vitali argument 133 151—155
Vitali theorem 57
Zero measure, criterion for sets to have 59
Zero measure, sets of 4 8 59 79 81
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 -sets by
-sets by 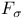 -sets
-sets  -regularity
-regularity 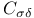 -regularity
-regularity 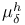 measure
measure 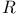 -regular measure
-regular measure  -field of
-field of