|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Honsberger R. — Mathematical Delights |
|
|
 |
| Предметный указатель |
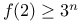 for a certain function f(x) 1 for a certain function f(x) 1
A 1997 USA Olympiad problem involving radical axes 4
A 1998 Putnam problem in triangle-geometry 4
A certain Diophantine equation in n variables 6
A certain sum of binomial coefficients 2
A chessboard problem concerning black squares 1
A counterfeit coin and an equal-arm balance 9
A curious property of a parabola 5
A difficult recursion 15
A game with piles of matches 9
A locus problem concerning equilateral triangles 8
A nasty integral 6
A note on perfect numbers 4
A prize problem of M. V. Subbarao 6
A problem concerning two incircles of triangles 1
A problem from From Erdos to Kiev revisited 13
A problem from In Polya ’s Footsteps revisited 13
A problem on concurrency 7
A problem on concurrent altitudes 1
A property involving an isosceles right triangle 7
A property of 5 points on a sphere 4
A property of a rectangle in a circle 1
A property of a rectangle with an extended side 7
A property of fans of abutting triangles 17
A property of six points in space 1
A property of the incircle and certain tangents in a triangle 7
A property of the series expansion of 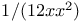 4 4
A property of trapezoids 7
A property of two-colorings of six points (in the Appendix) 2
A property related to two angle bisectors in a triangle 7
A safe-cracking problem 13
A special set of rational points on the unit circle 4
A surprising property of a circle 4
Algebraic number 4
An inequality concerning triangles of perimeter 2 4
An observation concerning algebraic numbers 4
Anastasiu, Doru Popescu 6
Andreescu, Titu 1 Challenges
Angles in a polygon 8 9
Another surprise from Leon Bankoff 6
Arbelos 4
Archimedes’ circles 4
Archimedes’ theorem of the broken chord 13
Archimedes’ Theorem of the Broken Chord (in the Appendix) 14
Arithetic mean-geometric mean inequality 1 8
Arithmetic congruence 9
Ashbacher, Charles 6
Asymptotic functions 6
Atanasov, Emanuil 1
Bankoff, Leon 6
Bankoff’s surprise 6
Barbara, Roy 4
Barry, P.D. 5
Berzsenyi, George 11
Binomial coefficients 2
Bipartite graph 4
Bisecting a series 2
Bloom, David 5
Brahmagupta’s theorem 11
Brown, Bud 5
Bruckman, Paul S. 6
Characterizations of Twin primes 18
Chebyshev polynomials 15
Chessboard, (Bishop’s problem) 4 11
Cipolla, Michele 18
Cipolla’s pseudoprimes 18
Circumcenter of a triangle 4 7
Clement, P.A. 18
Concerning cars A, B, C, D on a highway 1
Concurrency 4 7 11
Constructing a perpendicular with a special tool 9
Convex sets 14
Counterfeit coin 9
Cryptography 10
Cuoco, Al 5
De Moivre’s Theorem 2
Diminnie, Charles R. 15
Diophantine equation 4 6
Dodge, Clayton 4
Dubrovsky, Vladimir 11
Eddie 13
Eddie’s problem 13
Edmunds, E. J. 12
Eisenstein triples 5
ellipse 5 14
Equal-arm balance, heaviest stones 9
Euler line 11
Euler’s congruent numbers 5
Evagelopoulos, George 16
Fermat’s equation 6
Fermat’s Simple theorem 18
Fielstad, Paul 5
Finding all k that make 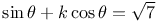 solvable 8 solvable 8
Finding heaviest of 32 stones with an equal-arm balance 9
Finding terms of a certain sequence that are divisible by 11 4
Fomin, Dimitry 9
Fukagawa, Hidetosi 17
Gebhardt, Robert 10
Gelca, Razvan Challenges
Graph, time-distance 1
Graph, time-distance of a function 10
Hahn, Liong-shin 8 13 14
Hammer, Peter 5
Helly’s theorem 14
Heron’s formula 5
How to construct a tangent to an ellipse 5
Incenter of a triangle (vector specification) 6
Incircle of a triangle 7 11
Indiameter of a right triangle 6
Induction 9 14 15
Inequality (geometric) 4
Infinite descent 1
Integer solutions of a system of equations 9
integral 6
Intermediate Value Theorem 6
Just, Erwin 4
Kanel, A. Challenges
Kirichenko, Alexey 9
Knox, Stephen W. 15
Koltchinskii, V. 14
Kovaldzhi, A. Challenges
Kuiper, Amy 6
Kulanin, E. 11
Kushnir, I. 11 Challenges
Larson, Loren 3
Law of Cosines 4 5 17
Leavitt, W.G. 18
Lehmer, D.H. 6
Mabry, Rick 5
Mathematics without words 5
Maurer, Stephen 10
| Mouse-clicking on a 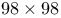 chessboard 4 chessboard 4
Mullin,A.A. 18
Mycielski, Jan 4
Nelsen, Roger B. 5
Novel ways of adding and multiplying geometrically 5
On 2n-gons inscribed in a circle 7
On a certain array of asterisks in a matrix 1
On a certain cryptographic method, base 5 10
On a certain Diophantine equation 4
On a certain equation in two variables 6
On a certain family of intersecting spheres 4
On a certain maximum sum-free subset 16
On a certain quadrilateral in a circle 8
On a certain red-black coloring of the cells of a matrix 4
On a certain regular polygon 7
On a certain star polygon 9
On a certain sum of greatest odd divisors 16
On a certain way of placing 1,2,..., 100 around a circle 9
On a monchromatic similar triangle in a 2-coloring of a plane 15
On a painted 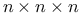 cube 6 cube 6
On a series that is won by a lead of two games 4
On a set of integers: sum of each five divides their product 9
On an octagon and a square in a circle 4
On Archimedes circles in the arbelos 4
On arithmetic progressions 9
On certain 70-million-digit integers 9
On certain prime numbers and powers of 3 16
On certain subsets of {1, 2,..., n} 4 9
On Eisenstein triples 5
On Euler’s congruent numbers 5
On folding the corners of a quadrilateral 8
On integers x, y, such that 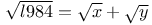 10 10
On lines that bisects both perimeter and area in a triangle 6
On nonintersecting graphs of functions f(x) and g(x) 10
On planting maple, oak, and birch trees 10
On properties of 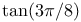 8 8
On reflecting the foot of an altitude in a side of a triangle 7
On removing a member from a certain set of 2n + 1 integers 3
On ten people around a circle 2
On the circumcenter of a certain triangle 7
On the indiameter of a right triangle (in the Appendix) 6
On the locations of points that make certain isosceles triangles 8
On the magnitudes of sums of powers of a desired sequence 8
On the minimum of a certain sequence of surds 8
On the radical axis of two circles (in the Appendix) 4
On the smallest circular cover of a triangle of unit perimeter 14
On the Smarandache functions S(n) and Z(n) 6
On would-be solutions of Fermat’s equation 6
Ordering 1,2,...,100, so neighbors differ by at least 50 9
Orthocenter of a triangle 4
Painted cube 6
parabola 5 Challenges
Pedoe, Dan 17
Perfect number 4
Pernice, Stephen G. 4
Planting trees 10
Polygon 13
Polygon, inscribed 4 7
Polygon, regular 7
Pompeiu 1
Pompeiu’s theorem 1
Prasolov, Viktor 7
Pritsker, Boris 11
Proizvolov, V. 11 Challenges
Proof of Bramagupta’s Theorem (in the Appendix) 1
Proof of Helly’s Theorem 14
Proof that arm/base in a 36°, 72°, 72°-triangle is  9 9
Pseudoprime 18
Quadrilateral 2
Quadrilateral in a circle 8
Quadrilateral, folding corners 8
Rabinowitz, Stanley 12
Radical axis 4 7
Rational points on a circle 4
rectangular array 1 4
Recursion 15
Reflection 7
Ribenboim, Paulo 18
Rotation 7
San Gaku 17
Savchev, Svetoslav 1
Schneider, Leo 2
Schoch, Thomas 4
Segal, Arthur C. 5
Sequence (special) 4 8
Sergusov, I.S.A. 18
Series, expansion 4
Series, expansion, of games 4
Shafaryan, V. 11
Sharygin, I. Challenges
Shier, Douglas 4
Smarandache functions 6
Spanning tree 4
Special sets of integers 3
Spheres 4
Stenlund, Mikko 5
Stereographic projection 5
Subbarao, M.V. 6
Subbarao’s prize problem 6
subsets 2 4
Sum-free sets 16
Tangent, ellipse 5
Tangent, ellipse, trig function 8
The bishop problem 4
The Euler line and isosceles triangles 1
The last problem on the 1988 IMO 1
The number of spanning trees in a 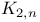 4 4
The sum of certain angles in a certain figure 8
The vector specification of the incenter of a triangle 6
To construct a certain segment across a triangle 12
Todd, Arthur 6
trapezoid 2 7 8
Trawalter, Sophie 6
Triangle, equilateral 1 8
Triangle, equilateral, 36°-72°-72° 9
Triangle, equilateral, area-penmeter bisector 11
Triangle, equilateral, isosceles 8 11
Twin primes 18
Two proofs of Heron’s formula 5
Two “mathematics without words” 5
Two-colorings of the plane 1
Two-colorings of the plane and Appendix 15
Vasilyev, N. Challenges
Velleman, Dan 17
Weiner, Paul A. 4
Wells, David 17
Wetzel, John 4
Wilson’s Theorem 18
Woo, Peter Y. 4
Wu, Rex Challenges
Yiu, Paul 4
Zuehlke, John 6
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



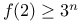 for a certain function f(x)
for a certain function f(x) 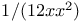
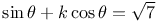 solvable
solvable  chessboard
chessboard  cube
cube