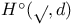Dorfman I. — Dirac Structures and Integrability of Nonlinear Evolution Equations :: Электронная библиотека попечительского совета мехмата МГУ

Главная Ex Libris Книги Журналы Статьи Серии Каталог Wanted Загрузка ХудЛит Справка Поиск по индексам Поиск Форум

Авторизация

Поиск по указателям

Dorfman I. — Dirac Structures and Integrability of Nonlinear Evolution Equations

Обсудите книгу на научном форуме

Нашли опечатку?
Выделите ее мышкой и нажмите Ctrl+Enter

Название: Dirac Structures and Integrability of Nonlinear Evolution Equations

Автор: Dorfman I.

Аннотация:

An introduction to the area for non-specialists with an original approach to the mathematical basis of one of the hottest research topics in nonlinear science. Deals with specific aspects of Hamiltonian theory of systems with finite or infinite dimensional phase spaces. Emphasizes systems which occur in soliton theory. Outlines current work in the Hamiltonian theory of evolution equations.

Язык:

Рубрика: Математика/Математическая Физика/

Статус предметного указателя: Готов указатель с номерами страниц

ed2k: ed2k stats

Год издания: 1993

Количество страниц: 183

Добавлена в каталог: 24.04.2005

Операции: Положить на полку | Скопировать ссылку для форума | Скопировать ID

Предметный указатель

$\tau$ -scheme for the KdV, Benjamin — Ono and Kadomtsev — Petviashvili equations      151—154
$\tau$ -scheme in Hamiltonian framework, symmetries of Dirac structures      154—157
$\tau$ -scheme of integrability      148—165
$\tau$ -scheme of integrability, examples with conserved Dirac structures      157—159
$\tau$ -scheme, Hamiltonian pairs      159—160
$\tau$ -scheme, Lenard scheme      161—165
$\tau$ -scheme, Lie derivatives in constructing      167
$\tau$ -scheme, Liouville equation      164—165
$\tau$ -scheme, sine-Gordon equation      163—164
2-cococycles      35 98—99
3-dihedral group of algebraic structures      137—139
Adler — Gelfand — Dikii method      106—111 113—114
Algebraic theory, Dirac structures      8—32
Belavin — Drinfeld solutions, Yang — Baxter equation      29—32
Benjamin — Ono (BO) equation      153 157
Benney’s moment equations      102—104
Brackets      see “Poisson brackets” “Schouten
Characteristic foliation      19—20
Coboundary      10 14 33—35
Cochains      10
Cocycles      10
Cohomologies      10 12 14 65—68 99
Commutative symmetry algebra      148—154
Complex of formal variational calculus      57—74
Complex of formal variational calculus, cohomology group 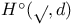$H^{\circ}(\sqrt{}, d)$       65—68
Complex of formal variational calculus, construction      57—60
Complex of formal variational calculus, exactness problem      62—65
Complex of formal variational calculus, general procedure of solving $\delta f/\delta u = g$       69—73
Complex of formal variational calculus, invariant operations expressed in terms of Frechet derivatives      61—62
Complex of formal variational calculus, the equation $\delta f/\delta u = g$ in the ring of polynomials and smooth functions      68—69
Complexes, de Rham      8 12—14 18—19 22 57—60
Complexes, over Lie algebra      11—15
Condition for operator to be Hamiltonian      77—79
Conjugate operator      14—15
Coupled nonlinear wave equation (CNW)      89—97
de Rham complex      8
de Rham complex of a ring      12—14
de Rham complex, construction      57—60
de Rham complex, Dirac structures      18—19
de Rham complex, symplectic operators      22
Density of the functional      59
Differential-geometric type symplectic operators of      146—147
Dirac structures, $\tau$ -scheme      154—159
Dirac structures, algebraic theory      8—32
Dirac structures, basic to Hamiltonian theory      4
Dirac structures, constituting a pair      127—130
Dirac structures, definition      16—17
Dirac structures, finite-dimensional class      18—19
Dirac structures, Hamiltonian operators      22—27
Dirac structures, Lenard scheme of integrability      48—51
Dirac structures, Nijenhuis relations      45—51
Dirac structures, Nijenhuis relations; pairs of Dirac structures      45—51
Dirac structures, pairs of, and Nijenhuis operators      33—56
Dirac structures, reduction to symplectic structures      5—6
Dirac structures, related to Liouville, sine-Gordon, modified KdV and nonlinear Schrodinger equations      131—136
Dirac structures, symplectic operators      20—22
Dubrovin — Novikov-type Hamiltonian structures      104—106
Evolution equations, integrability      34 6—7
Evolution equations, related to local Hamiltonian operators      75—114
Evolution equations, related to local symplectic operators      115—147
Exactness problem in the complex of formal variational calculus      62—65
Faddeev’s auxiliary conditions      5
Foliation, characteristic      19—20
Frechet derivatives, complex of formal variational calculus      60—62
Frechet derivatives, Hamiltonian conditions      77—79
Frechet derivatives, local symplectic operators      116—119
Frobenius theorem      19—20 31
Functional, density of the      59
Graded spaces      8—10
Hamiltonian operators, $\tau$ -scheme      154—157
Hamiltonian operators, Adler — Gelfand — Dikii method of constructing, pairs      106—111 113—114
Hamiltonian operators, conditions in an explicit form      77—79
Hamiltonian operators, Dirac structure      22—27
Hamiltonian operators, Dubrovin — Novikov-type      104—106
Hamiltonian operators, first order, in the one-variable case      80—82
Hamiltonian operators, Kirillov — Kostant-type hydrodynamic structures      102—104
Hamiltonian operators, Korteweg — de Vries and Harry Dym equations      85—89
Hamiltonian operators, Lenard scheme for Hamiltonian and symplectic pairs      51—56
Hamiltonian operators, Lie derivatives in constructing Hamiltonian pairs      159—160
Hamiltonian operators, local, and evolution equations      75—114
Hamiltonian operators, pairs and associated Nijenhuis operators      42—48
Hamiltonian operators, third-order      82—85
Hamiltonian operators, upper bounds for the level of symplectic operators and      142—146
Hamiltonian operators, Virasoro algebra      100—102
Hamiltonian operators, Yang — Baxter equation      29—31
Hamiltonian theory, general algebraic scheme      8—32
Hamiltonian theory, introduction      1—7
Hamiltonian vector fields, $\tau$ -scheme      154—157
Hamiltonian vector fields, Dirac structures      17
Hamiltonian vector fields, symplectic operators      21—22
Harry Dym(HD) equations      85—89
Hereditary or A-operators      56
Homotypy, algebraic      63—64
Integrability, x-scheme      148—165 (see also “Particular subjects”)

Invariant operations expressed in terms of Frechet derivatives      61—62
Invariants and symmetries      15—16
Inverse scattering method      34
Kadomtsev — Petviashvili(KP) equation      153—154 158
KdV (Korteweg-de Vries) equation, $\tau$ -scheme      151—153 158—159 162—163
KdV (Korteweg-de Vries) equation, coupled nonlinear wave equation      89—97
KdV (Korteweg-de Vries) equation, Dirac structures      135
KdV (Korteweg-de Vries) equation, Hamiltonian dynamical system      5—6
KdV (Korteweg-de Vries) equation, Hamiltonian theory      3—7
KdV (Korteweg-de Vries) equation, Lenard schemes for the potential      125—130
KdV (Korteweg-de Vries) equation, local Hamiltonian operators      85—89
KdV (Korteweg-de Vries) equation, Virasoro algebra and two Hamiltonian structures of the      100—102
Kirillov — Kostant structures, Adler — Gelfand — Dikii method      106—111 113
Kirillov — Kostant structures, canonical example of a Poisson structure      4
Kirillov — Kostant structures, deformations of      96—100 113
Kirillov — Kostant structures, hydrodynamic, Benney’s moment equations      102—104
Kirillov — Kostant structures, infinite-dimensional      89—97
Kirillov — Kostant structures, symplectic structure      24
Kirillov — Kostant structures, Virasoro algebra      100—102
KN equation      see “Krichever — Novikov(KN) equation”
Korteweg-de Vries equation      see “KdV (Korteweg-de Vries) equation”
KP equation      see “Kadomtsev — Petriashvili(KP) equation”
Krichever — Novikov(KN) equation, Hamiltonian presentation      6
Krichever — Novikov(KN) equation, Lenard scheme      122—125
Krichever — Novikov(KN) equation, pair of local symplectic operators      120—125 147
Lax type equations      109—111
Lenard scheme, $\tau$ -scheme      161—165
Lenard scheme, applied to the Krichever — Novikov(KN) equation      122—125
Lenard scheme, for Hamiltonian and symplectic pairs      51—56
Lenard scheme, hierarchy of the Liouville equation      134—135
Lenard scheme, integrability for Dirac structures      33 48—51
Lenard scheme, KdV (Korteweg-de Vries) equation      93—94
Lenard scheme, Liouville equation      165
Lenard scheme, local Hamiltonian operators      85—89
Lenard scheme, sine-Gordon equation      133—134 163—164
Lenard scheme, two, for the potential KdV equation      125—130
Lie algebra, commutative symmetry      148—154
Lie algebra, complexes over      11—15
Lie algebra, de Rham complex      8
Lie algebra, deformations of, and Nijennuis operators      33—35
Lie algebra, framework for Dirac structures      6
Lie algebra, graded spaces      9—10
Lie algebra, Lie derivatives      15—16 159—160
Lie algebra, local Hamiltonian operators      90—97
Lie algebra, Poisson bracket      2—3
Lie algebra, skew-symmetry      2—3
Lie algebra, structures in the space of 1-forms      25—27
Lie algebra, Yang — Baxter equation      29—32
Liouville equation      3 41 134—135 164—165
Matrix differential operators, local Hamiltonian operators      75—77
Matrix differential operators, symplecticity conditions      115—117
Matrix differential operators, theorem      101—102
Nijennuis operators, deformations of Lie algebras      33—35
Nijennuis operators, Hamiltonian pairs      42—48
Nijennuis operators, hierarchies of 2-forms generated by regular structures      39—42
Nijennuis operators, Nijenhuis torsion, conjugate to      37—39
Nijennuis operators, pairs of Dirac structures      33—56
Nijennuis operators, pairs of Dirac structures, Nijenhuis relations      45—51
Nijennuis operators, properties of and two Dirac structures      129—130
Nijennuis operators, properties of, symmetry generation      36—37
Nonlinear Schrodinger(NLS) equations      135—136
Poisson brackets, Lenard scheme of integrability      50 52
Poisson brackets, Lie algebra structure      2—3 17—18
Poisson brackets, presymplectic manifold      4—5
Poisson brackets, reference      114
Presymplectic manifold      4—5
Reduction procedure      15—16
references      166—172
Ring of polynomials, complex of formal variational calculus      65 68—69
Schouten bracket, the      27—29 90—92
Schrodinger (NLS) system, nonlinear      135—136
Sine-Gordon equation      133—134 163—164
Skew-symmetry, Hamiltonian operators      26—27
Skew-symmetry, Lie algebra      2—3
Skew-symmetry, local Hamiltonian operators      76—77
Skew-symmetry, local symmetric operators      116—120
Skew-symmetry, mapping      14
Soliton theory      3 114
Structure function      96—100
Superalgebra, Lie      9—10 28
Symmetries and invariants      15—16
Symmetry generation, properties of Nijenhuis operators      36—37
Symplectic manifold      1—2 4—5
Symplectic operators, conditions guaranteeing symplecticity of a pair      54—55
Symplectic operators, constituting a pair      48
Symplectic operators, differential-geometric type      146—147
Symplectic operators, graphs of linear operators      20—22
Symplectic operators, Lenard scheme for Hamiltonian and symplectic pairs      51—56
Symplectic operators, local, and evolution equations related to them      115—147
Symplectic operators, many-variable case with linear dependence on $u_j^{(i)}$       137—142
Symplectic operators, one-variable case: first- and third-order      117—120
Symplectic operators, upper bounds for the level of, and Hamiltonian operators      142—146
Virasoro algebra      100—102
Yang — Baxter equation, Belavin — Drinfeld solutions      29—32

О проекте