|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Elliot P.D.T.A. — Probabilistic Number Theory Two: Central Limit Theorems |
|
|
 |
| Предметный указатель |
kronecker 770 777 313 319 320
Kronecker symbol 110 111 313
Kronecker symbol, primitive character 110 319
Kubik 336
Kubilius 5 6 77 80 775 779 722 723 725 726 728 729 738 739 740 742 744 745 146 147 748 780 787 258 286 12 15 17 22 23 25 26 28 50 145 263 264 267 287 288 303 331 336
Kubilius — Erd s — Kac 9 31 48 125 s — Kac 9 31 48 125
Kubilius — Tur n 6 13 147 152 158 173 182 185 192 199 275 16 29 31 55 113 114 118 266 268 269 272 282 n 6 13 147 152 158 173 182 185 192 199 275 16 29 31 55 113 114 118 266 268 269 272 282
Kubilius — Tur n inequality 6 13 147 192 199 14 29 31 113 118 266 268 269 272 282 n inequality 6 13 147 192 199 14 29 31 113 118 266 268 269 272 282
Kubilius — Tur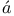 n inequality, dual of 13 147 194 335 55 114 n inequality, dual of 13 147 194 335 55 114
Kubilius’ model 115 146
Kubilius’ model, construction of 779 12 26 38 264 267 303
Kuipers and Neiderreiter 78
Kummer 111 329 338
Kummer’s problem and 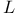 -series 329 338 -series 329 338
L$-series, Dirichlet 14 110 111 114 241 313
L$-series, Kummer’s problem 329 338
L vy 3 14 24 25 31 46 50 51 52 53 57 78 193 195 217 219 2 31 36 95 124 135 167 331 vy 3 14 24 25 31 46 50 51 52 53 57 78 193 195 217 219 2 31 36 95 124 135 167 331
L vy and Khinchine 49 57 135 144 vy and Khinchine 49 57 135 144
L vy metric, distance 14 24 31 33 48 56 95 96 97 132 134 vy metric, distance 14 24 31 33 48 56 95 96 97 132 134
L vy representation, formula 50 vy representation, formula 50
L vy representation, modified 51 124 vy representation, modified 51 124
L vy — Khinchine representation 49 50 57 144 vy — Khinchine representation 49 50 57 144
L vy’s continuity criterion 46 78 vy’s continuity criterion 46 78
Lagrange 339
Lagrange’s equations 339
Lambert 219 334
Lambert series 219 334
Lambert series, tauberian theorem 334
Landau 1 94 111 254 25 239 261 313 320
Landau and Walfisz 100
Lang 101
Large deviation inequality for random variables 127
Large deviations of additive functions 120 287 288 289
Large sieve 9 10 12 13 93 165 183 317 260 328
Lavrik 329
Law, Cauchy 14 136 143
Law, normal 11 17
Law, Normal, (definition) 52
Law, Normal, Gaussian 19 24 26 136 142 148 262 335
Law, Poisson 52 53 148 172 337
Law, stable 11 134 145
Laws, on a finite interval 58
Laws, which cannot occur 169
Lebesgue 16 19 21 22 23 48 59 67 107 222 228 258 260 359 7 63 74 91 92 152 154 155 164 170 171 199 308 314 327
Lebesgue  -class 22 -class 22
legendre 110 154 245 320
Legendre symbol 110 154 245 320
LeVeque 6 75 76 20 22 24 267 286 287
LeVeque’s conjecture 6 20 22 267 286 287
Levin and Falnleib 11 286 145
Levin and Timofeev 10 12 257 258 122 123 125 169 184 185 204 208 209 210
Levin, Barban and Vinogradov 174 27 50
Levin, Timofeev and Tuliaganov 274 275 280 281
Liapounoff 218
Limit law 24
Limiting distribution of a strongly additive function 4
Lindeberg 335
Lindeberg condition 335
Lindeberg — Feller 56 17
Lindeberg — Feller condition 56 17
Linnik 8 9 58 78 93 112 113 183 184 218 317 40
Linnik and Ibragimov 78
Liouville 238 254
Liouville’s function 238 254
Little wood — Hardy (Littlewood and Hardy) 2 77 102 254 348 351 358 255
Littlewood and Offord 217
Littlewood, P lya and Hardy 186 lya and Hardy 186
Lo ve 127 ve 127
Lubell 78
Lukacs 78
M bius 85 251 254 282 301 212 238 bius 85 251 254 282 301 212 238
M bius inversion 85 bius inversion 85
Major Arcs, intervals 7
Mann 77 293
Mann’s theorem 77 293
Manstavicius 286 305
Marcinkiewicz 58
Marek 111
Matthews 72 185
Mean, of a random variable 30
Measure 16
Measure probability 29 30 115
mellin 61 78 279 307 322
Mellin transform, M-transform 61 141 279 307 94 233 322
Mellin — Stieltjes 61 141
Mellin — Stieltjes transform 61 141 279 307
Mendelssohn, Fanny 111
Mendelssohn, Felix 111
Mendelssohn, Moses 772
Mendelssohn, Rebecka 111 112
Mertens 89 242
Method of Tur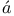 n, commentary on 112 n, commentary on 112
Metric, L vy 14 24 31 33 48 56 95 96 97 132 134 vy 14 24 31 33 48 56 95 96 97 132 134
Minor Arcs, intervals 7 8 9
Mirsky 762 166
Model for multiplicative functions 140
Models for strongly additive functions 115 146
Modified — L vy representation 51 124 135 vy representation 51 124 135
Moments, determination by 60 61
Moments, method of 59
Montgomery 93 185 229 235 317 138
Montgomery and Vaughan 185
Mordell 213
Munroe 18
Narkiewicz 103
Natural boundary 100
Niederreiter and Kuipers 78
Norm,  12 23 12 23
Norm, algebraic 114
Norm, operator 181 186
Normal law 11 17
Normal law (definition) 52 24 26 136 142 148 262 335
Normal law (Gaussian) 19
Normal number of prime factors 2
Normal order of an arithmetic function 2 14 41 43 98
Normal order zero 101
Norton 104 288
Offord and Littlewood 277
Operator, adjoint of 181 182
Operator, Dirichlet-series type 186
Operator, dual of 12 181 182
Operator, norm 181 186
Outer measure 159
P lya — Vinogradov 154 316 321 339 lya — Vinogradov 154 316 321 339
P lya — Vinogradov inequality 154 316 321 339 lya — Vinogradov inequality 154 316 321 339
P lya, Hardy and Littlewood 786 lya, Hardy and Littlewood 786
Pan 184
Pandora 98
Parseval 8 23 228 235 236 77 219 230 260 307
Parseval’s relation 8 23 228 235 236 77 219 230 260
Partitions 2
Paul 330
Perfect numbers 4
Periodic continued fraction 138
Perron 94 95 322 72 89 195
Perron’s theorem 94 95 322 326
Philipp 727 288
Plancherel 22 23
Plancherel’s identity 23 228 235 236 77 219 230 260
Poincar 3 3
poisson 52 21 145 148 172 173 199 337
Poisson law 52 — 53 148 172 337
Positive interval, bounded 72
Postnikov 77 74 78
Prachar 90 97 92 95 247
Prime ideal theorem 94
Prime number theorem 10 90 145 254 283 238
Prime number theorem, elementary proof 10 90 211 248
Prime numbers in arithmetic progressions 90 92 241
Prime Numbers in Arithmetic Progressions, distribution 89
| Primitive root, least positive 158
Probability measure 29 30 115
Probability space 29 115 146
Products of independent random variables 141 142 144
Proper distribution function 25
Purity of type 46 78 292
Quadratic Class Number 14 110 117 313
Quadratic residues, least pair of 153
Quasi-primes 42
R nyi 3 9 72 24 47 92 93 783 784 278 377 17 20 51 294 311 nyi 3 9 72 24 47 92 93 783 784 278 377 17 20 51 294 311
R nyi and Erd nyi and Erd s 333 s 333
R nyi and Tur nyi and Tur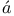 n 6 22 23 286 287 n 6 22 23 286 287
Rado 274
Raikov 58 772 773 172
Ramanujan 7 24
Ramanujan and Hardy 2 3 5 73 74 18 19 43 98 99 102 103 104 290 296 302 303 311
Random variable 29
Random variable, infinitely divisible 49
Relations asymptotic 19 21
Relatively stable 119
Richert and Halberstam 80 185
Riemann 96 97 154 338 341 79 185 193 241 248
Riemann hypothesis 97
Riemann zeta function 96
Riemann zeta function, functional equation 97
Rodosskii 245
Rogozin 32 78 278
rosser 22 24
Roth 72 784
Roth and Halberstam 77
Ruzsa, S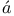 rk rk zy and Erd zy and Erd s 290 296 s 290 296
Ryavec 283 289
Ryavec and Elliott 10 257 258 265 268 283 306 169
Ryavec and Erd s 268 s 268
S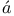 rk rk zy 312 zy 312
S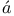 rk rk zy, Erd zy, Erd s and Ruzsa 290 296 s and Ruzsa 290 296
Sathe 23 302
Schmidt, R. 78 77
Schnirelmann 77 292 293 294 296
Schnirelmann density 77 293
Schnirelmann sum 293
Schoenberg (Sch nberg) 4 789 273 274 24 nberg) 4 789 273 274 24
Schur, J. 274
Schwarz — Cauchy 42 68 735 149 150 151 160 161 164 168 174 196 197 201 202 234 243 246 271 300 304 317 338 339 345 348 349 28 42 43 57 117 220 259 278 283
Schwarz, L. 248
see also Notation Asymptotic density, lower 295 297
see also Parseval’s relation Plancherel’s theory 22
Segal 98
Selberg 79 80 84 119 127 129 142 145 176 182 185 213 23 44 215 248 249 250 252 253 254 255 257 269 287
Selberg and Erd s 10 256 211 248 258 s 10 256 211 248 258
Selberg and Erd s, elementary proof of P.N.T. 248 — 253 s, elementary proof of P.N.T. 248 — 253
Selberg’s formula 248 249 250 252 255
Selberg’s sieve method 79 89 120 129 142 145 176 185 213 44 215 269
Shapiro 17 50 335
Shifted primes 9
Shohat — Fr chet 59 78 chet 59 78
Siegel — Walfisz 97 315 339
Sierpinski 76
Sieve, Brun 4 9 80 129 21 25 44
Sieve, large 9 10 12 13 93 165 183 317 260 328
Sieve, Selberg 79 — 89 120 129 142 145 176 185 213 44 215 269
Skew — Hermitian form 166
Slowly decreasing functions 102
Slowly oscillating functions 18
Solovay 340
Spectral radius 12 162
Spectral radius, of Hermitian matrix, operator 12 162
Spemer 32 78 274 276 278
Sperner’s lemma 32 78 214 216 218
Stable law 11 134 145
Stable law, characteristic exponent 135 142
Stable law, modified — L vy representation 135 vy representation 135
Steinhaus 76 76 267 283 24
Steinig and Diamond 261
Stepanov 755
Stieltjes — Fourier 67 305
Stieltjes — Mellin 67 747
Stirling 34 299 302
Stolz 338
Stone — Weiers trass 307
Substitutions, group of 13 183 209
Sums of independent random variables, limit theorems 54
Surrealistic Continuity Theorem 265 269 291
Sziisz 224
Tauber 77 700 707 702 254 357 358 255 260 334
Tauberian theorem, Hardy and Littlewood 77
Tauberian theorem, Hardy and Littlewood (statement) 102 254 348 351 358 255
Taylor 704 298
Tchebycheff 3 36 44 792 235 18 41 81 241
Tchebycheff’s inequality 3 192 18
Tchudakoff 260
Theodorescu and Hengartner 278
Three Series Theorem, Kolmogorov 37 38 77
Timofeev 285 286
Timofeev and Levin 10 12 257 258 122 123 125 169 184 185 204 208 209 210
Timofeev, Tuliaganov and Levin 274 275 280 281
Titchmarsh 10 22 58 59 70 90 94 95 97
Titchmarsh — Brun 90 120 135 160 161 213 32
Tjan 219
Toleuov and Fa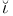 nle nle b 46 51 b 46 51
Total event 29
Truncated additive functions 5
Tuliaganov, Timofeev, and Levin 274 275 280 281
Tur n 3 4 5 6 147 180 181 182 185 16 18 20 22 23 24 41 43 50 98 112 118 119 120 n 3 4 5 6 147 180 181 182 185 16 18 20 22 23 24 41 43 50 98 112 118 119 120
Tur n and Erd n and Erd s 75 s 75
Tur n and R n and R nyi 6 22 23 286 287 nyi 6 22 23 286 287
Tur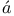 n — Kubilius 6 13 147 152 158 173 182 185 192 199 218 16 29 31 55 113 114 118 266 268 269 272 282 n — Kubilius 6 13 147 152 158 173 182 185 192 199 218 16 29 31 55 113 114 118 266 268 269 272 282
Turan and Erdos inequality 75
Turan — Kubilius inequality 6 13 147 192 199 14 29 31 113 118 266 268 269 272 282
Turan — Kubilius inequality, dual of 13 147 194 335 55 114
Turan’s letter 18 20
Turan’s letter, commentary on 20 24
Turan’s method, commentary on 112
TYPE 26
Uniform distribution (mod1) 66 69
Uniform law (mod1) 66
Urbanik 336
Uzdavinis 134 44 45 51 263 268
van Aardenne — Ehrenfest, de Bruijn and Korevaar 18 77
Variance, random variable 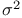 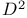 30 — 31 30 — 31
Vaughan and Montgomery 185
Vinogradov — Barban — Bombieri 51 269
Vinogradov — P lya 154 316 321 339 lya 154 316 321 339
Vinogradov — Polya inequality 154 316 321 339
Vinogradov, A.I. 92 93 184 338
Vinogradov, A.I. and Barban 122 126 263
Vinogradov, A.I., Barban and Levin 174 27 50
Vinogradov, I.M. 2 7 8 154 755 41 138 245
Viola and Forti 72 185 186 317
von Mangoldt 97 311 214
von Mangoldt’s function 97 311
Walfisz and Landau 100
Walfisz — Siegel 97 315 339
Weak convergence of distribution functions 24 25
Weak convergence of distribution functions (mod 1 65
Weak convergence of measures 24 30
Weierstrass — Stone 307
Weil 755
Weyl 69 75 284
Weyl’s criterion 69
Weyl’s criterion, quantitative 75
Wiener 68 100
Wiener - Ikehara 100 101
Wiener — Ikehara 100 101 102
Wiener — Ikehara tauberian theorem 100 101
Wintner 3 10 59 78 254 285 24
Wintner and Erd s 4 10 187 214 254 280 24 172 260 332 335 s 4 10 187 214 254 280 24 172 260 332 335
Wintner and Jessen 46 78 193
Wintner — Erdos theorem 187 224
Wirsing 10 11 90 144 225 226 227 254 255 256 273 331 213 217 218 241 256 257 258 259 260 261
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 s — Kac
s — Kac 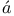 n
n 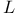 -series
-series  vy
vy  -class
-class  lya and Hardy
lya and Hardy  ve
ve  nle
nle