The physical world is studied by means of mathematical models, which consist of differential, integral, and integro-differential equations accompanied by a large assortment of initial and boundary conditions. In certain circumstances, such models yield exact analytic solutions. When they do not, they are solved numerically by means of various approximation schemes. Whether analytic or numerical, these solutions share a common feature: they are constructed by means of the powerful tool of integration—the focus of this self-contained book.
An outgrowth of the Ninth International Conference on Integral Methods in Science and Engineering, this work illustrates the application of integral methods to diverse problems in mathematics, physics, biology, and engineering. The thirty two chapters of the book, written by scientists with established credentials in their fields, contain state-of-the-art information on current research in a variety of important practical disciplines. The problems examined arise in real-life processes and phenomena, and the solution techniques range from theoretical integral equations to finite and boundary elements.
Specific topics covered include spectral computations, atmospheric pollutant dispersion, vibration of drilling masts, bending of thermoelastic plates, homogenization, equilibria in nonlinear elasticity, modeling of syringomyelia, fractional diffusion equations, operators on Lipschitz domains, systems with concentrated masses, transmission problems, equilibrium shape of axisymmetric vesicles, boundary layer theory, and many more.
Integral Methods in Science and Engineering is a useful and practical guide to a variety of topics of interest to pure and applied mathematicians, physicists, biologists, and civil and mechanical engineers, at both the professional and graduate student level.
 |
|
О проекте
|
|
О проекте



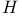 -functions
-functions